Scale diagrams
Topic Notes
What is a scale factor
Diagrams that you see may not always be drawn to its intended exact size. Instead, it may be scaled up to help you see the diagram better, or it may be scaled down so that it can fit on the medium that you're seeing the diagram on. Whichever it may be, scale diagrams may be drawn to a certain scale factor.
Scale factors are the ratio of two corresponding measurements on two similar figures. It is used in scaling and isn't constrained to 2D shapes. You can also use a scale factor with 3D shapes. If you have the scale factor, you can convert a measurement into another similar figure's measurements. An example of this in use is when you're changing a diagram on a paper's measurements to the real-life item.
How to find scale factor
Let's say you've got two figures: figure A and figure B. They are both similar, and figure B is an enlargement of figure A. How do you go about finding the scale factor between the two?
The scale factor, as a reminder, is a ratio, so what we're looking for is a ratio that can relate figure A and B. You can calculate the scale factor when you've got an enlargement by putting a side of the bigger figure divided by the smaller figure. That is:
In this case, it would mean that the scale factor of the enlargement = .
Alternatively, if you were looking at the scale factor of a reduction you'd put the smaller figure's side over the bigger figure's side. That is:
Example problems
See if you can tackle the below scale factor examples that deal with scale drawings and put into use what you know about the scale factor definition!
Question 1:
What is the actual length of this object?
The scale for the image of a leaf is 1 : 0.2.
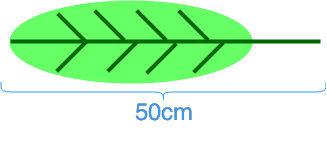
Solution:
Find the unknown:
10cm is the actual length of the leaf.
Question 2:
What is the actual length of this object?
The scale for the image of a building is 1: 406
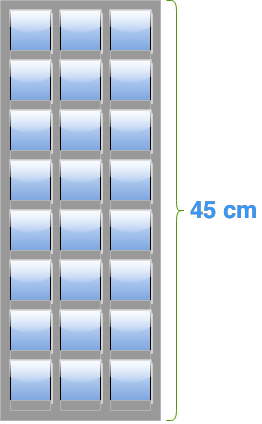
Solution:
Find the unknown:
18270cm is the actual height of the building.
Question 3:
Tony is 6cm tall in a photo. Yet, his actual height is 1.86 m. What is the scale factor?
Solution:
First, we need to convert unit:
So, put numbers in
Simplify it and we get the final answer

Want to double check your answers? Feel free to use this scale conversion calculator to help you out.
Coming up next, building on what you learn in this chapter, you'll be learning about congruent triangles and proving their congruence via SSS, SAS, HL, ASA, and AAS.

