Derivative of trig functions
Before we start learning how to take derivative of trig functions, why don't we go back to the basics? Going back and reviewing the basics is always a good thing. This is because a lot of people tend to forget about the properties of trigonometric functions. In addition, forgetting certain trig properties, identities, and trig rules would make certain questions in Calculus even more difficult to solve. Let's first take a look at the six trigonometric functions.
The 6 Trigonometric Functions
The first trigonometric function we will be looking at is . If we are to graph the function, we will get this:
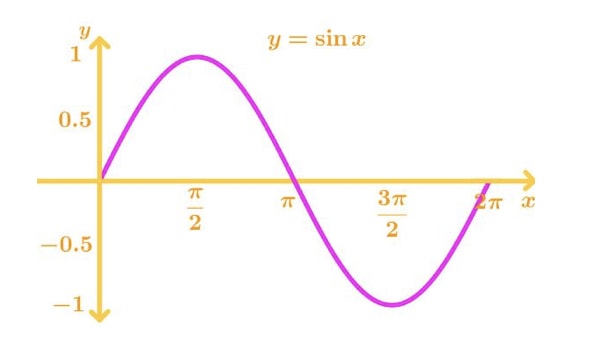
Notice that the function is continuous from [-], and it's a nice smooth curve with no sharp turns. This means that is differentiable at every point, and so we will not have to worry about getting something undefined. In addition, we know that the slope at are . Hence, the derivative of will always be zero at those points.
Next is the function . If we were graph the function, we will get:
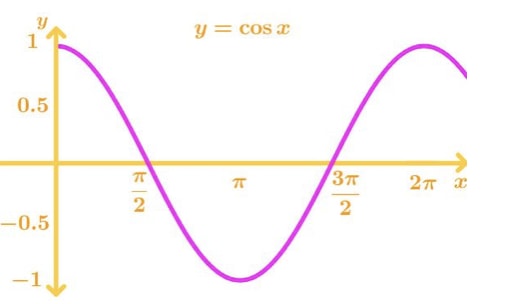
Again note that the function is continuous from [-], and it has a nice smooth curve. So is also differentiable at every point. In addition, the slope is equal to 0 at = 0 . Hence, the derivative will of will be 0 at those points.
Now the function is a bit more interesting. It can be rewritten as , the graph of this function looks like this:
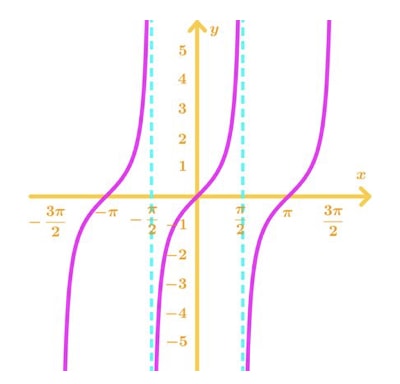
Notice the values at are undefined, and have vertical asymptotes. This means the derivative of will be not differentiable at those points. One interesting thing to note here is that the slope of is never negative or 0. Hence, we should expect the derivative of to always be positive.
Next are the reciprocal functions of sin cos tan. First, the reciprocal of is . We see the graph of looks like this:
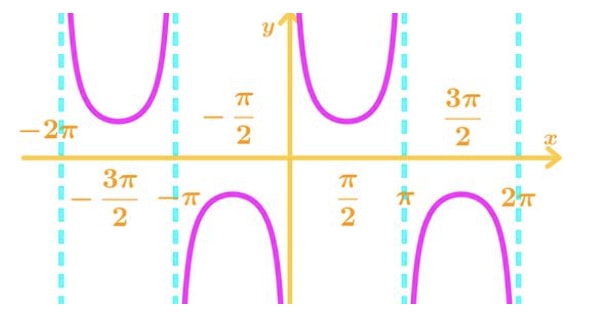
Again, there are vertical asymptotes at = 0 . So they are not differentiable at those points. In addition, the slope of the function at are 0. So the derivatives are always 0 at those points.
Now the next function is , which is the reciprocal of . Graphing gives:
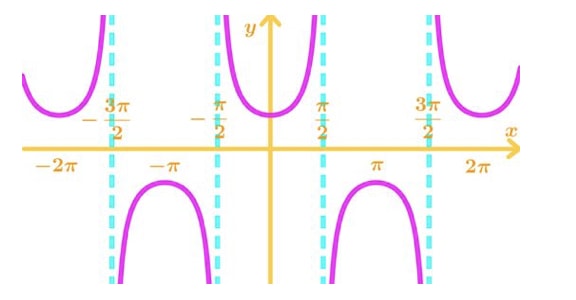
This is very similar to , but the difference is that the role's of the values has been switched. Now the values at are the vertical asymptotes and the value at are when the tangent slopes of the function are 0. Hence the derivative of the function is not differentiable at and the derivative is 0 at .
The last function is .
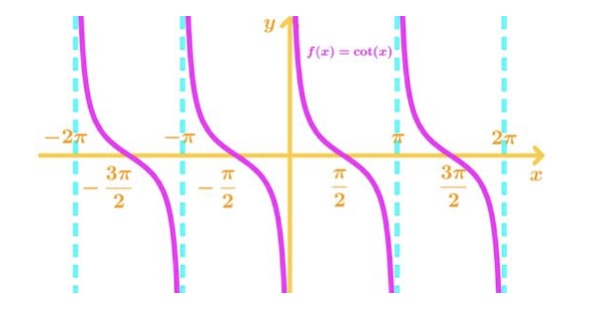
Note that the vertical asymptotes are at and the slope of this never positive or 0. Hence, the derivative of this function is always positive, and not differentiable at .
Now that we are finished looking at the 6 trig functions, let's now review some of the trigonometric identities that come in handy when taking derivatives.
Trigonometric identities
The first six identities are reciprocal identities, which come in handy when you want your derivatives in a certain form.

Now the most important identities are these three.
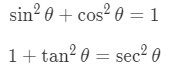
They are very useful when it comes to simplifying derivatives. Now that we've got the basics down, let's go ahead and actually take a look at the derivative of trig functions.
Trig derivatives
The six trig function derivatives are as follows:
1. The derivative of is:
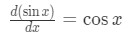
2. The derivative of is:
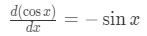
3. The derivative of is:
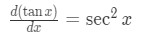
4. The derivative of is:

5. The derivative of is:

6. The derivative of is:
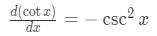
If you really want to know how we get the derivatives, then look at this article below:
Derivative of inverse trig functions
The article shows that the derivative of sin and cosine can be found using the definition of derivative, and the rest can be found with the quotient rule. Make sure you memorize these derivatives well! We will be using these to derive even harder trigonometric functions. You may also want to review the chain rule since a lot of hard trig derivatives require it.
Derivative of sin^2x
We may know that the derivative of sin is , but what about the derivative of ? Let's start with defining the function
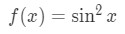
Let's move the square term so that the function becomes:
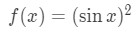
We are going to use the chain rule here. Recall that the chain rule states if you have a function within a function, call it
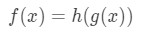
Then the derivative of this function will be
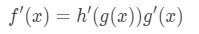
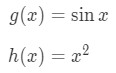
Then, the derivative of these functions will be:
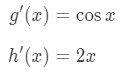
And so the derivative of the function f(x) will be:
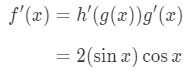
Which is the derivative of . It wasn't as hard as we thought! Let's look at around hard one.
Derivative of cos^2x
Again, we know the derivative of cosine is , but what about the derivative of ? We set the function to be
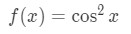
Again, if we rearrange the square in the function, then we will see that
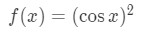
Using the chain rule again, we set:
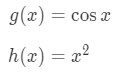
Taking the derivative of these functions gives:
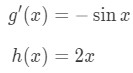
So the derivative of the function will be:
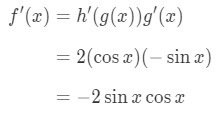
This is very similar to the derivative of , except we have an extra negative sign! Nevertheless, this is the derivative of . Let's try to find the derivative of another squared trigonometric function.
Derivative of sec^2x
Again, the key idea of taking the derivative of is using the chain rule. We define the function to be
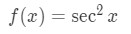
Rearranging the square in the function gives us:
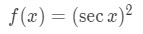
We set:
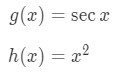
Taking the derivative of secant and gives:
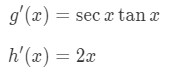
Again in case you forgot, the derivative of sec is . Hence, the derivative of is
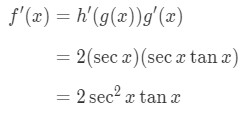
You should know by now that the procedure in taking the derivative of , and are the same. So let's take a look at other trigonometric derivatives.
Derivative of sinx^2
Now before you get confused, this is a function that looks similar to . However, is different from . So of course, there derivatives will also be different. We define the function to be:
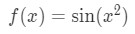
Note that we will use the chain rule here. We set
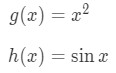
We know the derivative of g(x) is 2x and the derivative of is . So
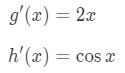
Hence the derivative of the function will be:
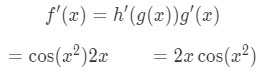
Now let's try the same thing for finding the derivative of !
Derivative of cosx^2
We define the function to be:
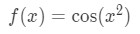
Using the chain rule we set:
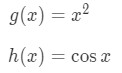
Taking the derivative of g(x) gives 2x and taking the derivative of cosine gives . So
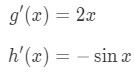
Hence the derivative of the function is:
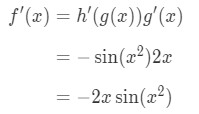
Let's do two more before we start doing even harder trigonometric functions.
Derivative of tanx^2
Again, the function we have is:
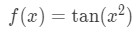
Using the chain rule, we set g(x) and h(x) to be:
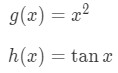
The derivative of is 2x, and the derivative of is , so
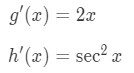
Using the chain rule formula, we get the derivative of tanx^2 is:
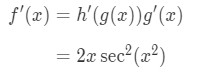
This one is a bit harder because the derivative seems to have two squares; one from secant and one from x. Lastly, let's look at the derivative of .
Derivative of cscx^2
The function we have is:
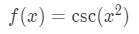
In order to do chain rule, we let two functions, g(x) and h(x), to be:
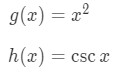
The derivative of is 2x, and the derivative of csc is -, so
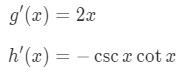
Using the chain rule formula, we get that the derivative of is:
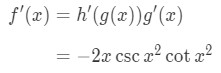
http://tutorial.math.lamar.edu/Classes/CalcI/DiffTrigFcns.aspx
Now it's time move on and take a look at some questions which have applications to the slope of a function.
Slope of a trigonometric function
Suppose we want to find the slope of the function:
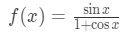
At the point x=0. How would we do it? Well, realize take find the slope of a function is just the same as taking the derivative. This will require using the quotient rule since the function is a quotient.
Recall that the quotient rule says the following:
If you have a function
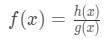
Then the derivative of this function will be:
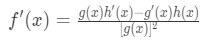
So if we were to set
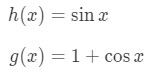
Then their derivatives will be:
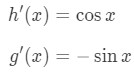
Hence, using the quotient rule gives the derivative:
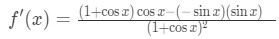
Simplifying this equation gives:
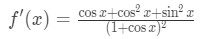
To simplify this equation, we would want to use the trigonometric identity:
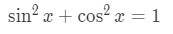
So our equation becomes:
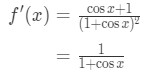
Now that we have the derivative, all we have to do is plug in the point x=0 to get the slope. Hence,
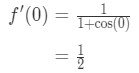
Thus, the slope of the function at the point x = 0 is exactly . However, what if we were given the tangent slope of a function at a specific point, and we need to find that point?
Finding the points given the slope
Suppose you are given that the function is:
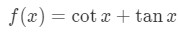
And you want to find the set of points which the slope of the tangent line is equal to 0. This means we need to take the derivative and set it equal to 0 to find the x values. Note that the derivative of is and the derivative of is –, so
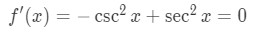
Note that the reciprocal identities state that:
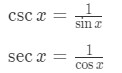
Hence, our equation becomes:
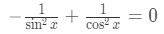
Let's try to solve for x in this equation. See that:
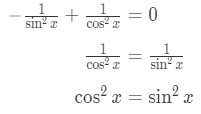
Moving the to the other side gives:
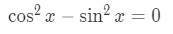
Recall the trigonometric identity
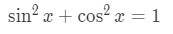
We can actually isolate by itself in the equation so that:
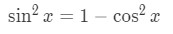
Substituting this into our equation gives:
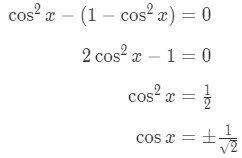
Now we need to look at the positive case and then the negative case. For the positive case we have:
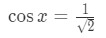
See that since x is not bounded, then were are infinitely many solutions. In fact, we know the solutions are:
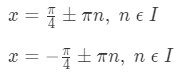
Now if we were to look at the negative case, then we have:
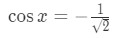
Again, there are infinitely many solutions for this. The solutions for this equation will be:
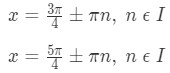
Now let's take a look at the four solutions as a whole. Notice that the two pairs are the same
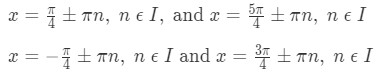
So we can just exclude the two solutions and say that the solutions are
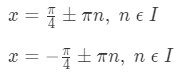
So we can conclude that these are the set of points in which the slope of the function is equal to 0.
Derivative of Inverse Trigonometric Functions
Now the Derivative of inverse trig functions are a little bit uglier to memorize. Note that we tend to use the prefix "arc" instead of the power of -1 so that they do not get confused with reciprocal trig functions. Regardless, they mean the same thing. For example, derivative of arctan is the same as the derivative of .
Here are the inverse trig derivatives that you will need to know.

Note that the arctan derivative is similar to the derivative of arccot, except there is an extra negative sign. Coincidently, we see that the derivative of arcsin is similar to the derivative of arccos. The difference again is the negative sign. We will not be doing any examples of Derivative of inverse trig functions here. However if you are interested, then please look at this article here:
Derivative of inverse trig functions
The article here gives a detailed step-by-step solution in deriving these derivatives.






