if:
then:
Differential Rules
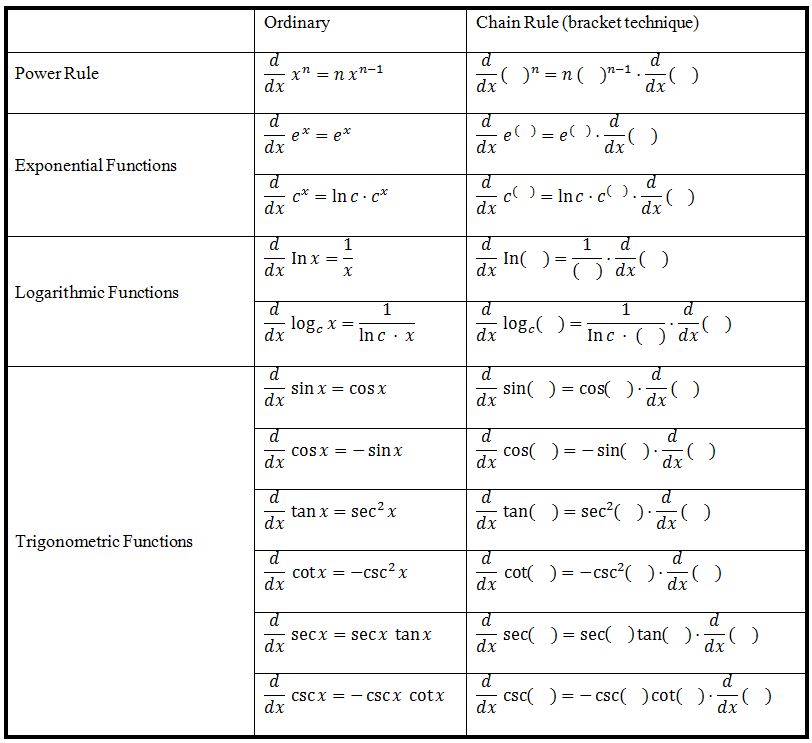
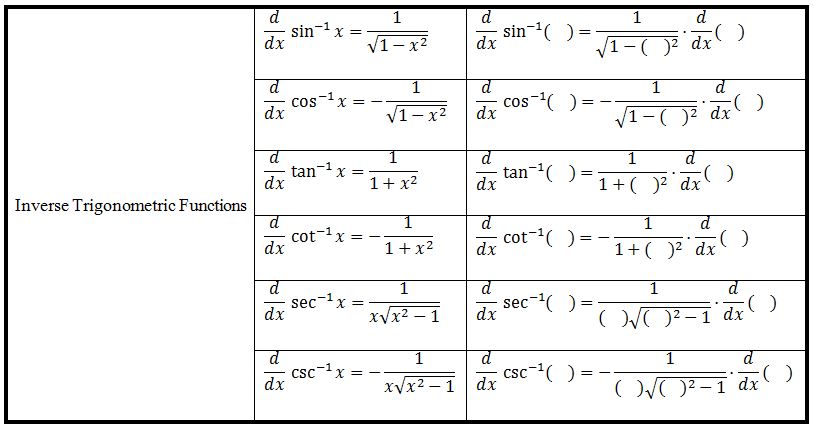





The Chain Rule is a fundamental concept in differential calculus, essential for calculating derivatives of composite functions. This powerful technique allows us to break down complex functions into simpler parts, making derivative calculations more manageable. The introduction video provides a clear and concise explanation of the Chain Rule, helping students grasp its significance and application. It's crucial to understand that the Chain Rule is used in virtually every derivative calculation, whether we explicitly recognize it or not. This ubiquity makes it an indispensable tool in calculus. By mastering the Chain Rule, students can tackle a wide range of mathematical problems, from basic differentiation to advanced applications in physics and engineering. The video demonstration offers valuable insights into how the Chain Rule works, providing a solid foundation for further exploration of differential calculus and its practical applications in various fields of study.
Differentiate: Rational Functions dxd(4x3+7)101
We need to find the derivative of the rational function (4x3+7)101. This function is in the form of a rational function, which can be challenging to differentiate directly. However, we can simplify the process by converting it into a power function.
To apply the power rule, we need to express the given rational function as a power function. Recall that a rational function of the form xn1 can be rewritten as x−n. Applying this to our function, we get:
(4x3+7)101=(4x3+7)−10
Now that we have the function in the form (4x3+7)−10, we can apply the power rule. The power rule states that the derivative of xn is nxn−1. For our function, the exponent n is -10. Therefore, we bring down the exponent and subtract 1 from it:
dxd(4x3+7)−10=−10(4x3+7)−11
Since the function inside the parentheses is not just x but a polynomial 4x3+7, we need to apply the chain rule. The chain rule states that we must multiply by the derivative of the inner function. The inner function here is 4x3+7, and its derivative is:
dxd(4x3+7)=12x2
Therefore, applying the chain rule, we get:
dxd(4x3+7)−10=−10(4x3+7)−11⋅12x2
Next, we simplify the expression by combining the constants and terms:
−10⋅12x2(4x3+7)−11=−120x2(4x3+7)−11
Finally, we convert the expression back to its rational form. Recall that a negative exponent indicates that the term is in the denominator:
−120x2(4x3+7)−11=(4x3+7)11−120x2
Thus, the derivative of the given rational function (4x3+7)101 is (4x3+7)11−120x2. This step-by-step process demonstrates how to use the power rule and chain rule in combination to differentiate a complex rational function.
Q1: What is the Chain Rule in calculus?
A1: The Chain Rule is a fundamental principle in calculus used for differentiating composite functions. It states that if y = f(u) and u = g(x), then the derivative of y with respect to x is given by dy/dx = f'(u) * du/dx. In simpler terms, it allows us to break down complex functions into simpler parts for differentiation.
Q2: When should I use the Chain Rule?
A2: You should use the Chain Rule when dealing with composite functions, which are functions within functions. Examples include sin(x²), e^(3x+1), or (x² + 3). Whenever you see a function that can be broken down into an outer function and an inner function, the Chain Rule is likely necessary.
Q3: What is the "bracket technique" in applying the Chain Rule?
A3: The bracket technique is a method used to visually organize composite functions when applying the Chain Rule. It involves placing brackets around the inner function and treating it as a single variable when differentiating the outer function. This technique helps maintain the structure of complex functions and ensures correct application of the Chain Rule.
Q4: How does the Chain Rule differ for simple and complex functions?
A4: The fundamental approach of the Chain Rule remains the same for both simple and complex functions. However, complex functions often require multiple applications of the rule and may involve more intricate algebraic manipulations. Simple functions typically involve straightforward derivatives and multiplications, while complex functions may lead to more elaborate expressions.
Q5: What are common mistakes to avoid when using the Chain Rule?
A5: Common mistakes include forgetting to apply the Chain Rule when necessary, incorrectly identifying inner and outer functions, and forgetting to multiply the results of each differentiation step. To avoid these, always check for composite functions, work from the "outside in," and remember that the Chain Rule involves the product of derivatives, not their sum.
Mastering the chain rule in calculus requires a solid foundation in several key mathematical concepts. One of the most crucial prerequisites is understanding composite functions. These functions form the backbone of the chain rule, as it essentially deals with differentiating composite functions. By grasping how functions can be nested within one another, students can better comprehend the chain rule's application in calculus.
Another vital concept to grasp is the power of a power rule. This rule is fundamental in calculus, particularly when dealing with the chain rule. It helps students understand how exponents behave when applied to expressions, which is crucial when differentiating complex functions. Similarly, the power of a product rule and the power of a quotient rule are essential prerequisites. These rules provide the foundation for understanding more complex differentiation techniques, including the chain rule.
As students progress in their calculus journey, they'll encounter more advanced topics that build upon the chain rule. For instance, functions expressed as power series often require the application of the chain rule for differentiation. Understanding this concept helps students see the broader applications of the chain rule in higher-level mathematics.
The chain rule also plays a crucial role when dealing with the derivative of inverse trigonometric functions. These functions are often composed with other functions, making the chain rule indispensable for their differentiation. Similarly, the derivative of exponential functions frequently involves the chain rule, especially when dealing with composite exponential functions.
By thoroughly understanding these prerequisite topics, students can approach the chain rule with confidence. The chain rule is not an isolated concept but rather a culmination of various mathematical principles. It serves as a bridge between basic differentiation techniques and more advanced calculus concepts. Mastering the chain rule opens doors to solving complex real-world problems in physics, engineering, and economics, where functions are often composed of multiple layers.
In conclusion, the journey to mastering the chain rule is paved with these essential prerequisite topics. Each concept builds upon the others, creating a robust foundation for understanding this fundamental calculus principle. By dedicating time to these prerequisites, students not only prepare themselves for the chain rule but also develop a deeper appreciation for the interconnectedness of mathematical concepts in calculus.
