System of linear-quadratic equations

0/1
Introduction
Lessons
  1. • The solutions to a system of equations are the points of intersection of the graphs.
    • For a system consisting of a linear equation and a quadratic equation:
    linear equation: y=mx+by = mx + b
    quadratic equation: y=ax2+bx+cy = a{x^2} + bx + c
    There are 3 cases to consider:

    case 1: 2 solutions case 2: 1 solution case 3: no solutions

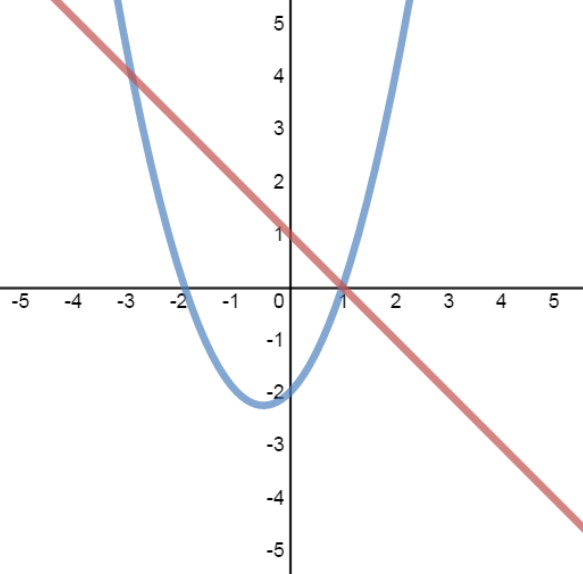
    System of linear-quadratic equations with two solutions

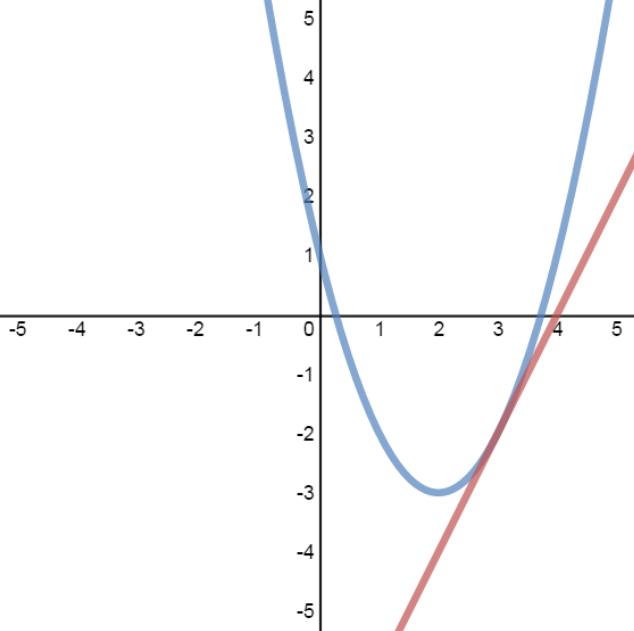
    System of linear-quadratic equations with one solution

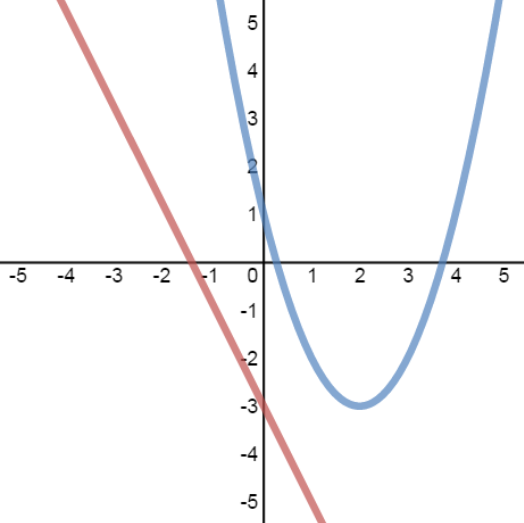
    System of linear-quadratic equations no solution
0/6
Examples
Lessons
  1. Case 1: System with 2 Solutions
    1. Solve the system:
      y=−x+1y = - x + 1
      y=x2+x−2y = {x^2} + x - 2
    2. Verify the solutions graphically
  2. Case 2: System with 1 Solution
    1. Solve the system:
      2x−y=82x - y = 8
      y=x2−4x+1y = {x^2} - 4x + 1
    2. Verify the solutions graphically
  3. Case 3: System with No Solutions
    1. Solve the system:
      10x+5y+15=010x + 5y + 15 = 0
      y=x2−4x+2y = {x^2} - 4x + 2
    2. Verify the solutions graphically
Free to Join!
StudyPug is a learning help platform covering math and science from grade 4 all the way to second year university. Our video tutorials, unlimited practice problems, and step-by-step explanations provide you or your child with all the help you need to master concepts. On top of that, it's fun - with achievements, customizable avatars, and awards to keep you motivated.

  • Easily See Your Progress

    We track the progress you've made on a topic so you know what you've done. From the course view you can easily see what topics have what and the progress you've made on them. Fill the rings to completely master that section or mouse over the icon to see more details.

  • Make Use of Our Learning Aids

    Last Viewed
    Practice Accuracy
    Suggested Tasks

    Get quick access to the topic you're currently learning.

    See how well your practice sessions are going over time.

    Stay on track with our daily recommendations.

  • Earn Achievements as You Learn

    Make the most of your time as you use StudyPug to help you achieve your goals. Earn fun little badges the more you watch, practice, and use our service.

  • Create and Customize Your Avatar

    Play with our fun little avatar builder to create and customize your own avatar on StudyPug. Choose your face, eye colour, hair colour and style, and background. Unlock more options the more you use StudyPug.
Topic Notes
The solutions to a system of equations are the points of intersection of their graphs. There are 3 cases you will come across when trying to solve the system. There can be 2 solutions, 1 solution or even no solutions.
Basic Concepts
Related Concepts