Use cosine ratio to calculate angles and sides (Cos = $\frac{a}{h}$ )

Trigonometric ratios

cosine rule

The cosine rule tells us that when we have a right triangle, $cosine = \frac{a}{h}$. The "a" in this case stands for adjacent. The "h" stands for the hypotenuse, which can be found through the pythagorean theorem. In order to find cosine, all you'll need is the adjacent side and the hypotenuse.

sine rule

When you come across sine, you can find the answer for it in a right triangle by taking the opposite side over the hypotenuse in the form of $sine = \frac{o}{h}$.

tangent rule

For the tangent rule, when you have a right triangle, you can use the opposite over the adjacent sides of the triangle to find your ratio. This means that $tan = \frac{o}{a}$.

SohCahToa

When you hear SohCahToa, it's not immediately obvious what it means. But it's actually an easier way for you to remember how to use sine, cosine, tangent that we just learned. These three are the main functions that you'll deal with in trigonometry problems.

Soh Cah Toa stands for:

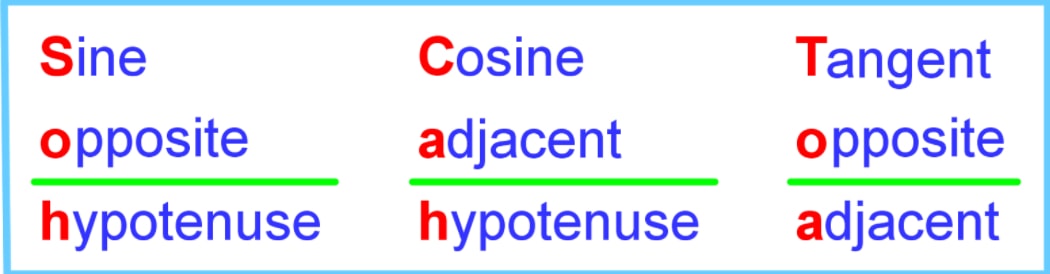
It can help you find the length of a side of a right triangle as long as you have an angle $\theta$ and some info on the other sides of the triangle.

Example problems

In this chapter, we're actually going to focus on the cosine rule. This means we'll only be working with the "$Cah$" portion of $SohCahToa$. Try out the following trig problems alongside us to learn how to solve questions using the cosine rule.

Question 1

Determine each cosine ratio using calculator

a) $\cos$ 50°

Simply put the number into your calculator and you should get $0.64$.

b) $\cos$ -50°

Simply put the number into your calculator and you should get $0.64$.

$\cos$ 50° and $\cos$ -50° both = $0.64$. Why?

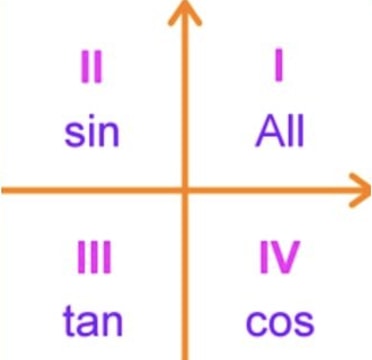
This above ASTC chart helps you figure out which trig ratio is positive in which quadrant. $\cos$ 50° lies in quadrant I, where all the trig ratios are positive. $\cos$ -50° lies in quadrant 4, where cosine is positive. This is why we get $0.64$ for both $\cos$ 50° and $\cos$ -50°.

Question 2

Determine the angle to the nearest degree

Solution

a) $\cos \theta = 0.24$

Use the inverse cosine in the calculator which has a little $-1$ beside $cos$:

$\arccos (0.24) = 76$°

b) $\cos \theta = -0.45$

Use the inverse cosine in the calculator to find:

$\arccos (-0.45) = 117$°

Question 3

Determine the angles and sides using cosine

Solution:

a) Find angle $A$ and $B$:

$\cos \theta = \frac{adjacent}{hypotenuse}$

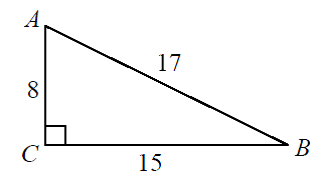
Angle $A$

$\cos A = \frac{8}{17}$

To solve it in your calculator

$\arccos \frac{8}{17} = 62$°

Angle $B$

$\cos B = \frac{15}{17}$

To solve it in your calculator

$\arccos \frac{15}{17} = 28$°

b) Find the value of "$x$" using cosine

$\cos \theta = \frac{adjacent}{hypotenuse}$

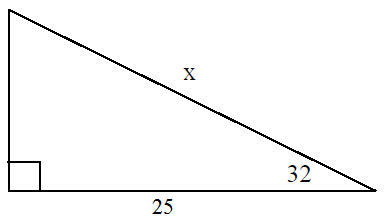
$\cos$ 32° = $\frac{25}{(x)}$

$x = \frac{25}{\cos 32}$

$x = 29$

Still curious about the cosine rule? Try out this online law of cosines calculator!

Next up, you're going to learn more about the law of cosines, how to find exact trigonometric ratios, and touch on what the unit circle is in trigonometry.