Using Exponents to Describe Numbers: A Comprehensive Guide Unlock the power of exponents in mathematics. Learn how to efficiently represent numbers using exponential notation, simplify complex expressions, and apply exponent rules with confidence.
Intros
0/1 watched
Examples
0/9 watched
- Write in exponential notation, and calculate.
- Complete the table.
Repeated Multiplication Exponential Form Value 9 × 9 × 9 × 9 ____ 2 × 2 × 2 ____ ____ ___________ ____ 25 ___________ ____ 216 ___________ ____ - Complete the table.
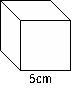
Repeated Multiplication Exponential Form Value (-6) × (-6) × (-6) × (-6) ____ (-8) × (-8) × (-8) ____ (-1) × (-1) × (-1) ____ ____ ___________ ____ ___________ ____ -1024 - The side length of the cube below is 5 cm. Write the volume of the cube in repeated multiplication form and as a power.
- Write 16 as a power where the exponent is 4 and the base is:






