Understanding Integer Multiplication: From Basics to Mastery

Dive into the world of integer multiplication with our comprehensive guide. Learn visual methods, explore patterns, and tackle real-world problems to enhance your mathematical prowess.

Write the multiplication statement for each diagram.

Step 1: Introduction to the Problem

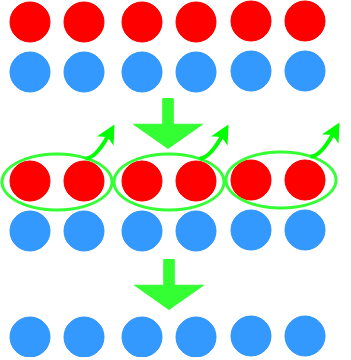
Hi, welcome to this question right here. This might seem challenging at first, but it's actually quite simple once you understand the concept. We are trying to figure out the result when two integers are multiplied together. To make this easier, we will use a diagram to illustrate the process. This visual representation will help you understand the multiplication of integers more clearly.

Step 2: Understanding the Diagram

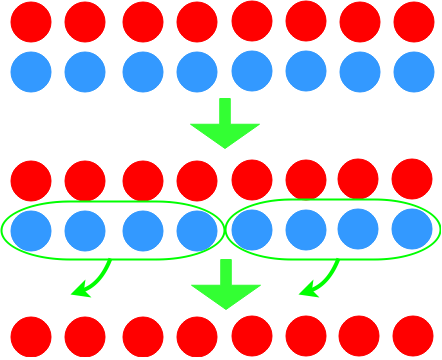
In the diagram, you will see red circles and blue circles. For the purpose of this explanation, treat the red circles as positive integers and the blue circles as negative integers. Initially, we have six positive circles and six negative circles, which balance each other out to zero. This is because six positives and six negatives cancel each other out.

Step 3: Identifying the Elements

Next, observe the green arrows in the diagram. These arrows indicate that we are taking away some circles. The act of taking away is represented by a negative sign. Now, count the number of circles being taken away. In this case, we are removing three green circles. This is represented as negative three (-3).

Step 4: Counting the Positive Circles

Within each of the green circles that we are taking away, there are two red circles. Remember, the red circles are positive. Therefore, each green circle contains two positive integers. This is represented as plus two (+2).

Step 5: Formulating the Multiplication Statement

Now, we combine these elements to form the multiplication statement. We are taking away three sets of two positive circles. This can be written as -3 (the number of sets being taken away) multiplied by +2 (the number of positive circles in each set). The multiplication statement is thus -3 * +2.

Step 6: Calculating the Result

To find the result, multiply the numbers together. Three times two equals six. Since we are multiplying a negative number by a positive number, the result is negative. Therefore, -3 * +2 equals -6. This means that when you take away three sets of two positive circles, you are left with six negative circles.

Step 7: Conclusion

In conclusion, the diagram helps us visualize the multiplication of integers. By identifying the elements and understanding their significance, we can easily formulate and solve the multiplication statement. The final result of -3 * +2 is -6, which is represented by the six negative circles left in the diagram.

1. What is the rule for multiplying integers with different signs?

   When multiplying integers with different signs (one positive and one negative), the result is always negative. For example, 5 × (-3) = -15 and (-4) × 2 = -8. This rule is often summarized as "unlike signs make negative."

2. How do integer chips help in understanding multiplication?

   Integer chips provide a visual representation of multiplication. Red chips represent positive integers, while blue chips represent negative integers. When multiplying, you either "insert" or "remove" chips based on the factors. This method helps students visualize the process and understand why multiplying two negative numbers results in a positive product.

3. Why does multiplying two negative numbers result in a positive product?

   Multiplying two negative numbers results in a positive product because it's equivalent to "removing" a negative amount a negative number of times. For example, (-3) × (-4) can be thought of as removing 3 negative chips 4 times, which results in adding 12 positive chips, giving a final product of 12.

4. How can I quickly determine the sign of a product when multiplying multiple integers?

   To quickly determine the sign of a product when multiplying multiple integers, count the number of negative factors. If there's an even number of negative factors, the product will be positive. If there's an odd number of negative factors, the product will be negative. For example, in (-2) × 3 × (-4) × 5, there are two negative factors, so the product will be positive.

5. What are some real-world applications of integer multiplication?

   Integer multiplication has numerous real-world applications, including:

   • Financial calculations (e.g., calculating profits and losses)
   • Physics problems (e.g., force calculations)
   • Everyday scenarios like party planning or grocery shopping
   • Area calculations for home improvement projects
   • Estimating fuel costs for travel
   Understanding integer multiplication is crucial for solving problems in various fields and everyday situations.

Understanding integer multiplication is a crucial skill in mathematics, but to master it, students must first grasp several fundamental concepts. One of the most important prerequisites is comparing and ordering numbers, particularly when dealing with positive and negative numbers. This skill forms the foundation for understanding how integers behave in multiplication.

Before diving into multiplication, students should be comfortable with adding integers. Techniques such as adding integers with chips can help visualize the process, which is essential when transitioning to multiplication. The application of integer operations in real-world scenarios further reinforces these concepts, making integer multiplication more relatable and easier to grasp.

A solid understanding of the distributive property of multiplication is crucial when working with integer multiplication. This property helps students break down complex multiplication problems into simpler parts, making the process more manageable and less intimidating.

While it may seem unrelated at first, proficiency in multiplying decimals can greatly benefit students when learning integer multiplication. The principles of place value and the mechanics of multiplication remain consistent, whether dealing with decimals or integers.

Lastly, comparing and ordering rational numbers is an essential skill that ties directly into integer multiplication. Understanding how rational numbers behave in multiplication scenarios helps students develop a more comprehensive grasp of number systems and their operations.

By mastering these prerequisite topics, students build a strong foundation for understanding integer multiplication. Each concept contributes to a deeper comprehension of how integers interact when multiplied, making the learning process smoother and more intuitive. As students progress, they'll find that these fundamental skills not only aid in integer multiplication but also serve as building blocks for more advanced mathematical concepts.

It's important to note that while some students may be tempted to skip over these prerequisites, doing so can lead to gaps in understanding and difficulties down the line. Taking the time to thoroughly explore and practice these foundational concepts will pay dividends in the long run, not just for integer multiplication, but for mathematics as a whole. By approaching the subject with a solid grounding in these prerequisite topics, students set themselves up for success and a more enjoyable learning experience in mathematics.

• Place value
• Determining Common Factors
• Adding integers

• Order of operations (PEMDAS)
• Using exponents to describe numbers
• Exponent rules