Probability outcomes for coins, dice, and spinners
Topic Notes
In this lesson, we will learn:
- What is probability in math?
- How to write probability as a fraction
- How to list all the outcomes for probability events (using coins, dice, and spinners)
Notes:
- Probability is math for events that only sometimes happen. The chances of something happening can be likely or unlikely to happen.
- Calculating probability is like predicting the future. We are trying to get a measure of the chances that something will happen.
- Arithmetic math (all basic operations; adding, subtracting, multiplying, dividing numbers) is certain. In comparison, probability math is uncertain--but still predictable.
- Probability can be given as a fraction, following the formula format:
- Outcomes are all the possible endings that could happen for a situation.
- Some simple probability situations that are often used in math problems include: tossing a coin, rolling a six-sided die, and spinning the arrow on a spinner.
- Coins:

- If you toss a coin, it will land on a flat side—either on heads or tails
- So, there are 2 possible outcomes to a coin toss: heads or tails
- The probability of landing on heads is (heads) =
- The probability of landing on heads is (tails) =
- Dice:
 (a six-sided die, if singular)
(a six-sided die, if singular) - When rolling a die, it will land with one of its six flat faces facing up (on top). There are six sides labelled from 1 to 6.
- So, there are 6 possible outcomes to a die roll: 1, 2, 3, 4, 5, or 6
- The probability of landing on any one side (1-6) is one out of six chances
- (1) = (2) = (3) = (4) = (5) = (6) =
- Spinners:
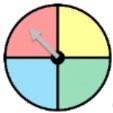 (can have any number of equal parts; labels with numbers, letters, etc.)
(can have any number of equal parts; labels with numbers, letters, etc.) - When using a spinner, the arrow will land on one of the marked regions. In this case, there are 4 different coloured regions.
- So, there are 4 possible outcomes for this spinner: red, yellow, green, or blue
- The probability of landing on any one of the colors is one out of four chances
- (Red) = (Yellow) = (Green) = (Blue) =
Introduction to Probability Outcomes
Welcome to the fascinating world of probability! Our introduction video sets the stage for understanding this essential mathematical concept. Probability is a branch of mathematics that deals with events that may or may not occur, making it both intriguing and practical. When we talk about probability, we often explore outcomes related to common objects like coins, dice, and spinners. These simple items help us grasp the fundamental ideas of likely and unlikely events. For instance, when flipping a coin, there are two possible outcomes: heads or tails. With dice, we have six potential results, while spinners can have various numbers of outcomes depending on their design. By studying these examples, we begin to understand how probability applies to real-world situations. Whether an event is likely or unlikely depends on the number of favorable outcomes compared to the total possible outcomes. This introduction lays the groundwork for deeper exploration into the world of probability and its wide-ranging applications in science, finance, and everyday decision-making.
Understanding Probability
Probability is a fascinating branch of mathematics that differs significantly from arithmetic math. While arithmetic deals with concrete numbers and definite outcomes, probability focuses on predicting the future and measuring the likelihood of events occurring. This concept is crucial in various fields, from weather forecasting to financial planning.
At its core, probability is about quantifying uncertainty. Unlike arithmetic math, where 2 + 2 always equals 4, probability deals with events that may or may not happen. This introduces the concept of certain events versus uncertain events. A certain event has a 100% chance of occurring, while an uncertain event has a chance between 0% and 100%.
To express probability mathematically, we use the probability fraction formula. This formula is written as:
Probability = (Number of favorable outcomes) / (Total number of possible outcomes)
This fraction always results in a value between 0 and 1, where 0 represents an impossible event and 1 represents a certain event. For example, when flipping a fair coin, the probability of getting heads is 1/2, because there's one favorable outcome (heads) out of two possible outcomes (heads or tails).
Let's explore some real-world examples to illustrate the concept of probability. Consider the probability of getting struck by lightning versus experiencing a sunny day in Hawaii. The National Weather Service estimates that the odds of being struck by lightning in a given year are about 1 in 500,000. We can write this as a probability fraction:
P(struck by lightning) = 1 / 500,000 = 0.000002 or 0.0002%
This extremely low probability makes getting struck by lightning an uncertain event, bordering on impossible. On the other hand, Hawaii is known for its sunny weather. Let's say that, on average, 300 out of 365 days in a year are sunny in Hawaii. We can calculate the probability of a sunny day as:
P(sunny day in Hawaii) = 300 / 365 0.82 or 82%
This high probability makes a sunny day in Hawaii a much more certain event, though still not guaranteed.
Understanding probability allows us to make informed decisions in uncertain situations. It helps us assess risks, plan for the future, and understand complex systems. While arithmetic math gives us exact answers, probability provides a framework for dealing with the uncertainties of life.
In conclusion, probability is a powerful tool for predicting the future and measuring uncertainty. By using the probability fraction formula and understanding the concept of certain versus uncertain events, we can quantify the likelihood of various outcomes. Whether we're analyzing weather patterns, making financial investments, or simply deciding whether to carry an umbrella, probability helps us navigate the uncertainties of our world with greater confidence and clarity.
Probability Outcomes for Coins
When it comes to a coin toss, the possible outcomes are straightforward yet fascinating from a probability perspective. A standard coin has two sides: heads and tails. These two sides represent the only possible outcomes when tossing a coin, making it a classic example of a binary outcome in probability theory.
Heads and tails are the two faces of a coin. The "heads" side typically features a portrait or significant symbol, while the "tails" side often displays a value or design. When you toss a coin, it will land showing either heads or tails, assuming it doesn't land on its edge (an extremely rare occurrence that we generally disregard in probability calculations).
Calculating the probability of landing on either heads or tails is straightforward. In a fair coin toss, each outcome has an equal chance of occurring. This is where the probability fraction comes into play. The probability of an event is calculated by dividing the number of favorable outcomes by the total number of possible outcomes. In the case of a coin toss, there is one favorable outcome (either heads or tails) out of two possible outcomes (heads and tails).
Therefore, the probability fraction for getting heads is 1/2, and the same applies to tails. This can also be expressed as 0.5 or 50%. It's important to note that the sum of all probabilities in a set of outcomes must equal 1 (or 100%), which is why heads and tails each have a 1/2 probability, totaling 1 when combined.
To illustrate this concept, let's consider some examples. If you toss a coin once, the probability of getting heads is 1/2, and the same for tails. If you were to toss the coin 100 times, probability theory suggests that you would expect to see approximately 50 heads and 50 tails. However, it's crucial to understand that this is an expected average over many trials, not a guaranteed outcome for any specific set of tosses.
The reason why the probability is 1/2 for each outcome lies in the physical properties of the coin and the nature of the toss. A fair coin is designed to be balanced, with equal weight distribution between both sides. When tossed with sufficient force and allowed to spin freely, the coin has an equal chance of landing on either side. This balance and the random nature of the toss ensure that neither heads nor tails has an inherent advantage.
Understanding the probability of coin tosses has practical applications beyond simple games of chance. It forms the foundation for more complex probability concepts and is often used in statistics, decision-making processes, and even in scientific experiments where random selection is required. The simplicity of the coin toss makes it an excellent tool for teaching basic probability concepts.
In conclusion, the coin toss exemplifies a simple yet powerful concept in probability. With only two possible outcomes - heads and tails - each having a probability of 1/2, it provides a clear illustration of equal odds. This fundamental understanding of probability in coin tosses serves as a stepping stone to more complex probability scenarios, making it a crucial concept in mathematics, statistics, and everyday decision-making processes.
Probability Outcomes for Dice
When rolling a six-sided die, there are six possible outcomes: 1, 2, 3, 4, 5, and 6. Understanding the probability of each outcome is crucial for various games, statistical analyses, and mathematical concepts. Let's explore the fascinating world of dice probability and how it applies to a standard six-sided die.
To calculate the probability of rolling any specific number on a six-sided die, we use a simple probability fraction. The probability is expressed as the number of favorable outcomes divided by the total number of possible outcomes. In the case of a six-sided die, there is only one favorable outcome for each number (one way to roll a 1, one way to roll a 2, and so on), and there are six possible outcomes in total.
Therefore, the probability of rolling any specific number on a six-sided die is 1/6. This means that for each roll, you have a one in six chance of getting any particular number. This probability applies equally to all numbers on the die, from 1 to 6.
Why does each outcome have a 1/6 probability? The reason lies in the fundamental principles of probability and the design of a standard die. A fair six-sided die is constructed to be as close to perfectly symmetrical as possible, with each face having an equal chance of landing face-up when rolled. This ensures that no particular number is favored over another, resulting in an equal probability for each outcome.
To illustrate this concept, let's consider some examples:
1. Rolling a 3: The probability of rolling a 3 is 1/6. There is only one face with the number 3, and there are six faces in total.
2. Rolling an even number: The probability of rolling an even number (2, 4, or 6) is 3/6 or 1/2. There are three favorable outcomes out of six possible outcomes.
3. Rolling a number greater than 4: The probability is 2/6 or 1/3, as there are two favorable outcomes (5 and 6) out of six possible outcomes.
Understanding these probabilities is essential for many games that involve dice. For instance, in board games like Monopoly or Yahtzee, knowing the likelihood of rolling certain numbers can inform strategic decisions. In more complex games or simulations, multiple dice may be used, compounding the probabilities and creating more intricate outcome possibilities.
It's important to note that while the theoretical probability of each outcome is 1/6, in practice, the results of actual die rolls may vary due to factors such as slight imperfections in the die or the rolling technique. However, over a large number of rolls, the results should approach the theoretical probability.
Dice probability serves as an excellent introduction to broader concepts in probability theory and statistics. It provides a tangible and easily understandable example of how probability works in real-world scenarios. Whether you're a student learning about probability for the first time or a seasoned statistician, the humble six-sided die remains a powerful tool for exploring and explaining the principles of chance and probability.
Probability Outcomes for Spinners
Spinners are fascinating tools used in probability and statistics to demonstrate random outcomes. These circular devices, often divided into colored sections, provide a hands-on approach to understanding chance and likelihood. In the realm of probability, spinners serve as excellent examples for calculating and predicting various outcomes.
To determine the possible outcomes for a spinner, we first need to examine its structure. A typical spinner consists of a circular disc divided into different colored sections. Each section represents a distinct outcome when the spinner is rotated and allowed to stop. The key to understanding spinner probability lies in analyzing these colored sections and their proportions.
Let's consider a basic example: a spinner with four equal sections colored red, blue, green, and yellow. In this case, there are four possible outcomes, each corresponding to one of the colors. To calculate the probability of the spinner landing on a specific color, we use the probability fraction. This fraction is determined by dividing the number of favorable outcomes by the total number of possible outcomes.
For our four-color spinner, the probability of landing on any single color is 1/4 or 0.25. This is because there is one favorable outcome (the desired color) out of four total possible outcomes. This equal probability for each color occurs when the sections are of equal size. However, spinners can have varying section sizes, which alters the probabilities.
Consider a different spinner configuration: a six-section spinner with three red sections, two blue sections, and one green section. In this case, the probability of landing on red is 3/6 or 1/2, as three out of the six sections are red. For blue, the probability is 2/6 or 1/3, and for green, it's 1/6. These fractions clearly show that red has the highest probability, followed by blue, and then green.
Spinners can become more complex, incorporating various colors and section sizes. For instance, imagine a spinner with eight sections: four large sections (two red and two blue) and four small sections (all yellow). If the large sections are twice the size of the small ones, we need to adjust our calculations. In this case, we might consider the spinner as having 12 equal parts: four red, four blue, and four yellow. The probability of landing on red or blue would be 4/12 or 1/3 each, while yellow would have a probability of 4/12 or 1/3 as well.
Understanding spinner probabilities is crucial in many real-world applications. Games of chance often use spinners or similar randomizing devices. In education, spinners are valuable tools for teaching probability concepts to students. They provide a visual and interactive way to explore outcomes and predict likelihoods.
As we delve deeper into probability theory, spinners can be used to demonstrate more advanced concepts. For example, they can illustrate compound events by spinning multiple times or using multiple spinners simultaneously. This leads to discussions about independent and dependent events, conditional probability, and the multiplication rule of probability.
In conclusion, spinners are versatile tools in the study of probability. By examining their colored sections and using the probability fraction, we can accurately calculate the likelihood of various outcomes. Whether used in simple games or complex statistical models, spinners provide a tangible way to understand and apply probability concepts. As we continue to explore the world of chance and likelihood, these spinning discs remain a fundamental and engaging tool in probability education and analysis.
Comparing Probability Outcomes
When exploring probability, coins, dice, and spinners are common tools used to understand the concept of chance. Each of these items offers unique probability outcomes, making them valuable for comparing and contrasting different scenarios. Let's delve into how these objects differ in their probability outcomes and how the number of possible outcomes affects the likelihood of each event.
Starting with coins, we encounter the simplest probability scenario. A standard coin has only two possible outcomes: heads or tails. This means that for a fair coin, the probability of getting either outcome is 1/2 or 50%. The limited number of outcomes makes coins ideal for introducing basic probability concepts.
Moving on to dice, we find a more complex probability situation. A standard six-sided die has outcomes ranging from 1 to 6. With six possible outcomes, the probability of rolling any specific number is 1/6 or approximately 16.67%. This increased number of outcomes compared to a coin results in a lower probability for each individual event.
Spinners introduce even more variability in probability outcomes. Unlike coins and dice, which have fixed numbers of outcomes, spinners can be designed with any number of sections. For instance, a spinner with four equal sections would have a 1/4 or 25% chance of landing on each section. However, spinners can also have unequal sections, leading to different probabilities for each outcome.
The number of possible outcomes directly affects the probability of each event. As the number of outcomes increases, the probability of any specific outcome generally decreases, assuming all outcomes are equally likely. This principle is evident when comparing a coin toss (1/2 for each outcome) to a die roll (1/6 for each outcome).
Probability scenarios become more interesting when we combine different tools. For example, consider tossing a coin and rolling a die simultaneously. This combination creates 12 possible outcomes (2 coin outcomes × 6 die outcomes). The probability of combined events, such as getting heads and a 3, is 1/12 or approximately 8.33%.
Calculating compound probabilities involves multiplying the individual probabilities of independent events. For instance, the probability of combined events like getting heads on a coin toss (1/2) and rolling a 6 on a die (1/6) would be calculated as 1/2 × 1/6 = 1/12. This method applies to more complex scenarios as well, such as spinning a four-section spinner and rolling two dice.
Understanding these principles allows us to analyze more complex probability situations. For instance, what's the probability of rolling an even number on a die and getting tails on a coin? Since there are three even numbers on a die (2, 4, 6), the probability is (3/6 × 1/2) = 1/4 or 25%.
As we explore more intricate probability scenarios, it's crucial to remember that the total probability of all possible outcomes must always equal 1 (or 100%). This fundamental rule helps verify calculations and understand the relationship between different outcomes in a probability experiment.
In conclusion, comparing probability outcomes for coins, dice, and spinners reveals how the number of possible outcomes affects individual event probabilities. By combining these tools and calculating compound probabilities, we can explore a wide range of scenarios, from simple coin tosses to complex multi-step experiments. This understanding forms the foundation for more advanced probability concepts and their real-world applications in fields such as statistics, science, and decision-making.
Real-World Applications of Probability
Probability concepts learned through simple objects like coins, dice, and spinners form the foundation for understanding complex real-world applications. These basic principles extend far beyond classroom exercises, playing crucial roles in various fields such as weather forecasting, sports analytics, and game theory. By exploring these applications, students can gain a deeper appreciation for the practical significance of probability in everyday life.
Weather forecasting is a prime example of probability in action. Meteorologists use complex models that incorporate probability to predict the likelihood of different weather conditions. For instance, when you see a "30% chance of rain" in a forecast, it's a direct application of probability concepts. This percentage is derived from multiple simulations and historical data, much like how we calculate the probability of getting heads in a coin toss, but on a much larger and more complex scale.
In sports analytics, probability plays a pivotal role in strategy development and player performance assessment. Teams and analysts use probability-based models to predict outcomes of games, evaluate player statistics, and make strategic decisions. For example, in baseball, the concept of "on-base percentage" is a probability measure of how often a player reaches base. This statistic helps managers make informed decisions about lineup orders and player substitutions, much like how understanding the probability of rolling a specific number on a die can inform strategy in board games.
Game theory, which studies strategic decision-making, heavily relies on probability concepts. In many real-world scenarios, from business negotiations to political strategies, understanding the likelihood of different outcomes is crucial. For instance, in a business negotiation, assessing the probability of a competitor's actions (similar to predicting an opponent's move in a game) can significantly influence decision-making strategies.
In daily life, probability concepts learned through simple games translate into practical decision-making skills. Understanding the likelihood of events helps in making informed choices, from deciding whether to carry an umbrella based on the weather forecast to choosing investment options based on market probabilities. Even in social situations, like estimating the chances of getting tickets to a popular concert, we unconsciously apply probability concepts learned through basic examples.
The insurance industry is another area where probability plays a central role. Insurance companies use complex probability models to assess risk and set premiums. These models are essentially sophisticated versions of the simple probability calculations we perform with dice or spinners. Understanding these principles can help individuals make better decisions about their insurance needs and coverage options.
In the field of medicine, probability is crucial for diagnosing diseases and determining treatment efficacy. Doctors use probability-based reasoning when interpreting test results or deciding on treatment plans. For instance, understanding the probability of false positives in medical tests is similar to calculating the likelihood of specific outcomes in repeated coin tosses.
Even in the entertainment industry, probability concepts find applications. Game shows often use probability-based challenges, and understanding these concepts can enhance the viewing experience and strategy for contestants. Similarly, in casino games, knowing the probabilities involved can inform responsible gambling practices and strategy development.
By recognizing these real-world applications, students can see how the basic probability concepts they learn using coins, dice, and spinners extend to complex, impactful scenarios. This understanding not only enhances their academic learning but also equips them with valuable skills for decision-making and risk assessment in various aspects of life. Whether it's in career choices, financial planning, or everyday decisions, the principles of probability learned through simple examples provide a foundation for navigating the uncertainties of the real world.
Conclusion
Understanding probability outcomes for coins, dice, and spinners is crucial in mastering basic probability concepts. The introduction video provides a solid foundation for grasping these fundamental ideas. Coins offer simple binary outcomes, while dice and spinners introduce multiple possibilities, each with its own probability. Students should focus on practicing calculations for various scenarios, such as determining the likelihood of rolling a specific number on a die or landing on a particular color on a spinner. By consistently working through different probability problems, learners can develop a strong intuition for chance events. As confidence grows, exploring more advanced probability topics becomes possible, opening doors to complex statistical analysis and real-world applications. Remember, probability is not just about numbers; it's about understanding uncertainty and making informed decisions based on likely outcomes. Mastering these basics paves the way for deeper insights into data science, risk assessment, and predictive modeling.
Introduction to Probability Outcomes for Coins, Dice, and Spinners:
What is probability?
Step 1: Understanding Probability
Probability is a branch of mathematics that deals with events that may or may not happen. It helps us understand the likelihood of different outcomes. In probability, we often talk about events that are either likely or unlikely to happen. For example, getting struck by lightning is very unlikely, while having a sunny day in Hawaii is very likely. Probability helps us quantify these chances.
Step 2: Likely vs. Unlikely Events
When discussing probability, we categorize events as likely or unlikely. Likely events are those that have a high chance of occurring, while unlikely events have a low chance. For instance, the probability of getting struck by lightning is very low, making it an unlikely event. Conversely, the probability of having a sunny day in Hawaii is high, making it a likely event.
Step 3: Comparing Probability Math with Arithmetic Math
Arithmetic math deals with certain outcomes, such as adding, subtracting, multiplying, and dividing numbers. In arithmetic, we know the exact numbers and operations needed to get a definite answer. For example, if you have two bags with five marbles each, you can be certain that the total number of marbles is 10. Probability math, on the other hand, deals with uncertain outcomes. We use probability to predict what might happen, but we can't be 100% sure until the event occurs.
Step 4: Predicting Outcomes with Probability
Probability is like predicting the future. We make educated guesses about what might happen based on the likelihood of different outcomes. For example, when flipping a coin, we can't be sure if it will land on heads or tails, but we can predict that there is a 50% chance for each outcome. This is because there are only two possible outcomes, and each is equally likely.
Step 5: Probability Fractions
In probability, we often express the likelihood of an event as a fraction. The numerator (top number) represents the number of desired outcomes, while the denominator (bottom number) represents the total number of possible outcomes. For example, the probability of a coin landing on heads is 1/2, because there is one desired outcome (heads) and two possible outcomes (heads or tails).
Step 6: Practical Examples
Let's consider a practical example. What is the probability of winning a prize draw in a class of 22 students? The total number of possible outcomes is 22 (one for each student). Since only one student can win, the probability of any one student winning is 1/22. This fraction represents the likelihood of winning the prize draw.
Step 7: Conclusion
Probability helps us understand and predict the likelihood of different events. By comparing likely and unlikely events, using probability fractions, and applying these concepts to practical examples, we can better grasp the chances of various outcomes. Whether flipping a coin, rolling a die, or spinning a spinner, probability provides a mathematical framework for making informed predictions.
FAQs
-
What is the probability of getting heads when flipping a coin?
The probability of getting heads when flipping a fair coin is 1/2 or 50%. This is because a coin has two equally likely outcomes (heads and tails), and there is one favorable outcome (heads) out of the two possible outcomes.
-
How do you calculate the probability of rolling a specific number on a six-sided die?
The probability of rolling any specific number on a standard six-sided die is 1/6 or approximately 16.67%. This is calculated by dividing the number of favorable outcomes (1, as there's only one face with the desired number) by the total number of possible outcomes (6, as there are six faces on the die).
-
How does the number of sections on a spinner affect the probability of landing on a specific color?
The number of sections on a spinner directly affects the probability of landing on a specific color. If all sections are equal in size, the probability is calculated by dividing 1 by the total number of sections. For example, on a spinner with 4 equal sections, the probability of landing on any specific color is 1/4 or 25%. If the sections are unequal, the probability is proportional to the size of each section relative to the whole spinner.
-
What is the difference between theoretical and experimental probability?
Theoretical probability is the expected probability based on the number of possible outcomes, calculated mathematically. For example, the theoretical probability of rolling a 3 on a six-sided die is 1/6. Experimental probability is based on the actual results of repeated trials. While experimental probability should approach theoretical probability over a large number of trials, it may vary in smaller sample sizes due to random chance.
-
How can understanding probability in coins, dice, and spinners help in real-world situations?
Understanding probability through these simple objects provides a foundation for analyzing more complex real-world scenarios. It helps in decision-making under uncertainty, risk assessment, and strategic planning. For example, this knowledge can be applied in fields like weather forecasting (predicting the likelihood of rain), finance (assessing investment risks), or even in everyday decisions like choosing the best route to avoid traffic based on probabilities of congestion.
Prerequisite Topics
Understanding probability outcomes for coins, dice, and spinners is a fundamental concept in mathematics and statistics. While this topic doesn't have specific prerequisites listed, it's important to recognize that a strong foundation in basic mathematical concepts is crucial for grasping these probability concepts effectively.
To fully comprehend probability outcomes for coins, dice, and spinners, students should have a solid understanding of fractions, decimals, and percentages. These mathematical tools are essential for expressing probabilities accurately. For instance, when calculating the probability of rolling a specific number on a die, you'll need to express it as a fraction (e.g., 1/6) or convert it to a decimal or percentage.
Additionally, a basic understanding of set theory can be beneficial. While not explicitly required, familiarity with sets and subsets can help in visualizing and organizing possible outcomes in probability scenarios. This knowledge aids in identifying favorable outcomes and the total number of possible outcomes, which are crucial components in probability calculations.
Basic arithmetic skills are also important prerequisites. Students should be comfortable with addition, subtraction, multiplication, and division, as these operations are frequently used in probability calculations. For example, when determining the probability of multiple events occurring together, multiplication of individual probabilities is often necessary.
Furthermore, an introduction to basic statistical concepts can provide valuable context. While not strictly required, understanding concepts like randomness and sample space can enhance a student's grasp of probability outcomes. These ideas help in conceptualizing the nature of chance events and the range of possible results.
Logical reasoning skills are another implicit prerequisite. The ability to think critically and analyze different scenarios is crucial when working with probability. Students need to be able to identify all possible outcomes and understand how different events relate to each other.
Lastly, familiarity with graphical representations can be helpful. While not a strict prerequisite, the ability to interpret and create simple graphs and charts can aid in visualizing probability distributions, especially when dealing with spinners or more complex probability scenarios.
By ensuring a strong foundation in these fundamental mathematical and logical concepts, students will be better equipped to tackle the intricacies of probability outcomes for coins, dice, and spinners. This solid base allows for a smoother transition into more advanced probability topics and applications in various fields, from statistics to data science and beyond.

