Multiplying decimals by powers of 10
Topic Notes
In this lesson, we will learn:
- What are powers of 10?
- How to move the decimal place when multiplying decimals by powers of 10
- How to understand decimal multiplication using base ten (block) models
Notes:
- All place values are related to their neighboring place values by a factor of 10. A place value is 10 times more than the place to its right and 10 times less than the place to its left.
- Each place value can be represented as a power of ten
- Recall: repeated addition becomes multiplication, ex. 10 + 10 + 10 = 3 ×10
- Repeated multiplication becomes powers (or exponents), ex. 10×10×10 = 103
- You can see that the exponent is the same as the number of zeroes represented
- What happens when you multiply by powers of ten?
- When you multiply any number by 1, it stays exactly the same!
- What happens when you multiply by 10? 100? 1000?
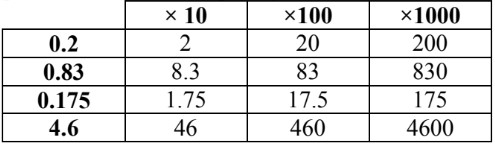
- You can use the number of zeroes as the number of places that a decimal must jump to the right when multiplying by powers of ten.
- If you run out of numbers when moving the decimal to the right, fill those spaces with trailing zeroes!
- We can also use base ten (block) models to multiply decimals with powers of 10. There are two different models, depending on what represents one whole:
- If one whole is represented by a hundred block (square): ex. multiplying 5 tens blocks by 10 will give you 5 wholes.
- If one whole is represented by a thousand cube: ex. multiplying 3 ones blocks by 100 will give you 3/10 wholes (or 3 tenths).
 × 10 = 50 tens = 5 wholes =
× 10 = 50 tens = 5 wholes = 
× 100 = 3 hundreds = 3/10 wholes =