In this section, we will be showing and describing transformations of given figures on coordinate planes. In the previous section, we used only one type of transformation for each question. In contrast, in this section, we will be using several types of transformations for each question. For example, we will be given a figure on a coordinate plane and asked to describe what combination of translations, reflections and rotations would need to occur to move this figure from one location to another on the plane.
Mastering Horizontal and Vertical Distances on Coordinate Planes Unlock the power of coordinate geometry! Learn essential skills for translations, reflections, and rotations. Boost your problem-solving abilities and excel in geometry with our comprehensive guide.
Get the most by viewing this topic in your current grade. Pick your course now.
Intros
Introduction to Transformations on Coordinate Planes
Transformations on coordinate planes are fundamental concepts in geometry that involve moving or altering geometric shapes. The three main types of transformations are translations on coordinate planes, reflections, and rotations. Translations involve sliding a figure in any direction without changing its size or shape. Reflections flip a figure over a line, creating a mirror image. Rotations turn a figure around a fixed point. Understanding these transformations is crucial for grasping more advanced geometric concepts. The introduction video provides a clear explanation of how to work with horizontal and vertical distances in transformations. This knowledge is essential for accurately plotting points and moving shapes on coordinate plane. By mastering these basic transformations, students can develop a strong foundation for more complex geometric operations and problem-solving skills. The video serves as an excellent starting point for exploring the fascinating world of coordinate geometry and spatial relationships.
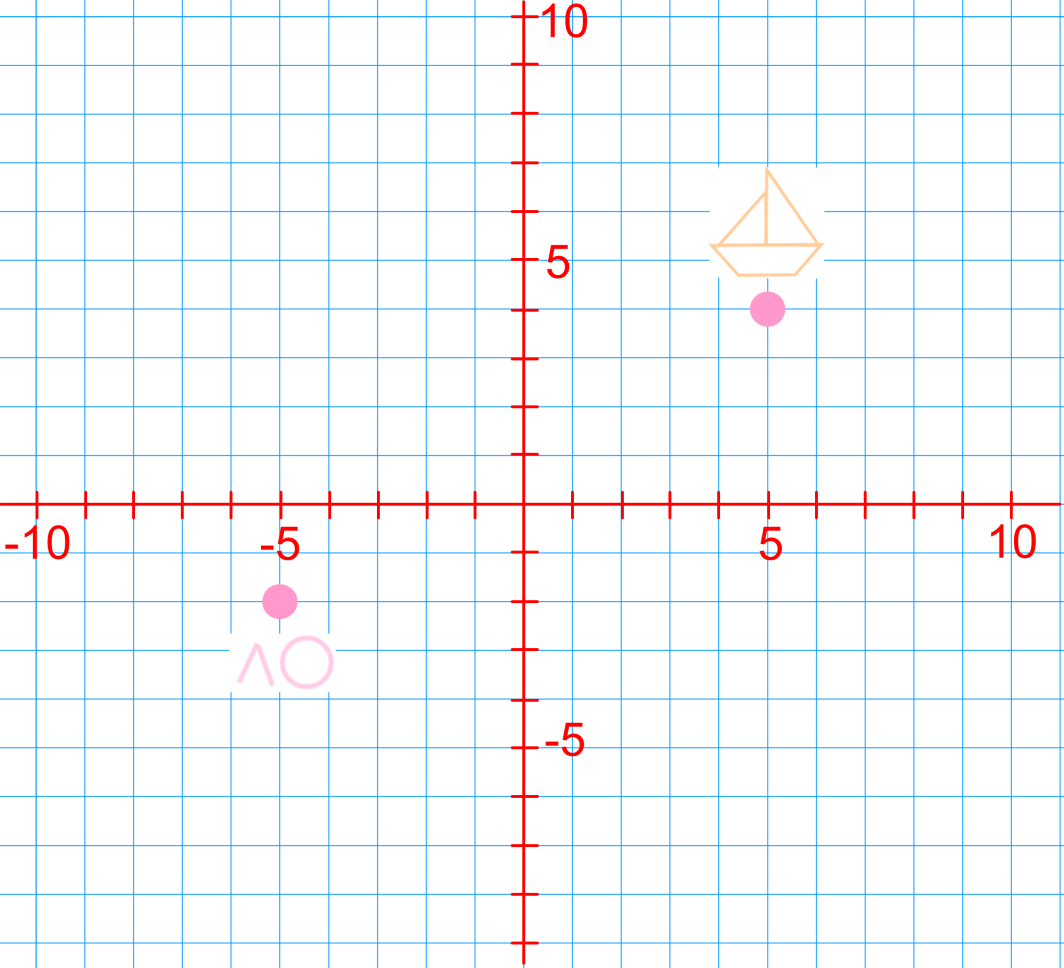
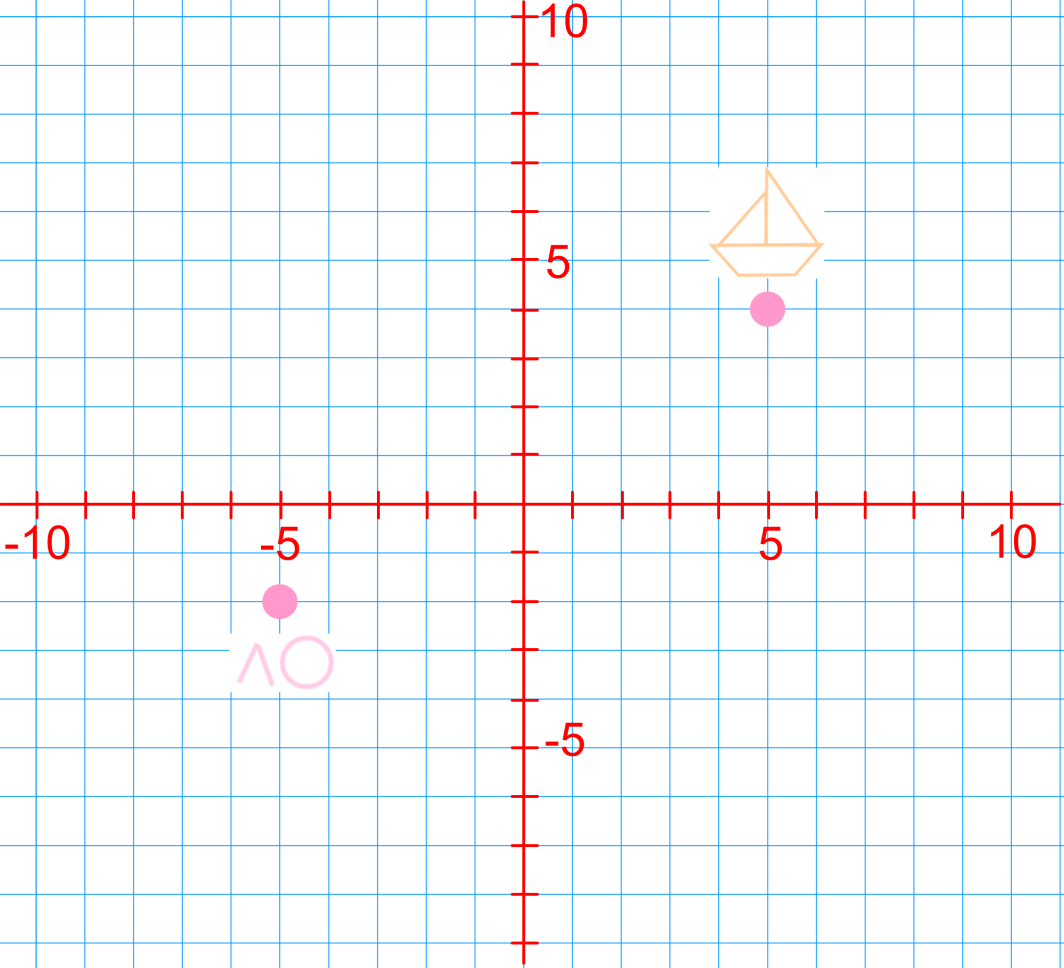
Use translations, reflections and rotations to get the swimmer to the boat.
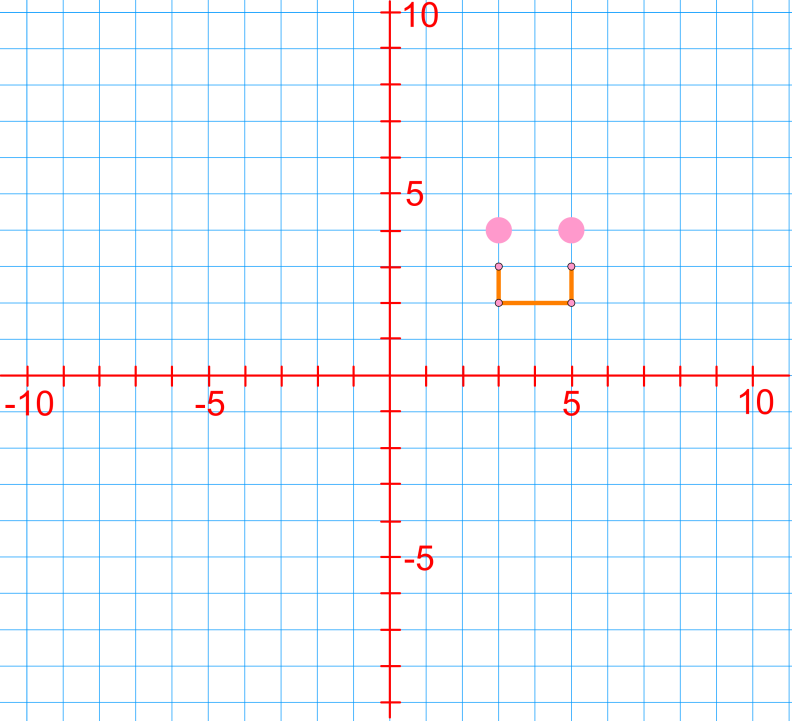
Step 1: Reflection
The first step to move the swimmer to the boat is to use a reflection. We will reflect the swimmer across the y-axis. Imagine the y-axis as our line of reflection, which we will label as R. To perform the reflection, we need to draw a line that is perpendicular to the y-axis from the swimmer's current position. This line will help us determine the new position of the swimmer after the reflection.
Start by counting the number of units the swimmer is from the y-axis. Suppose the swimmer is 5 units to the left of the y-axis. To reflect the swimmer, we will move the same number of units to the right of the y-axis. Therefore, the swimmer will be 5 units to the right of the y-axis after the reflection.
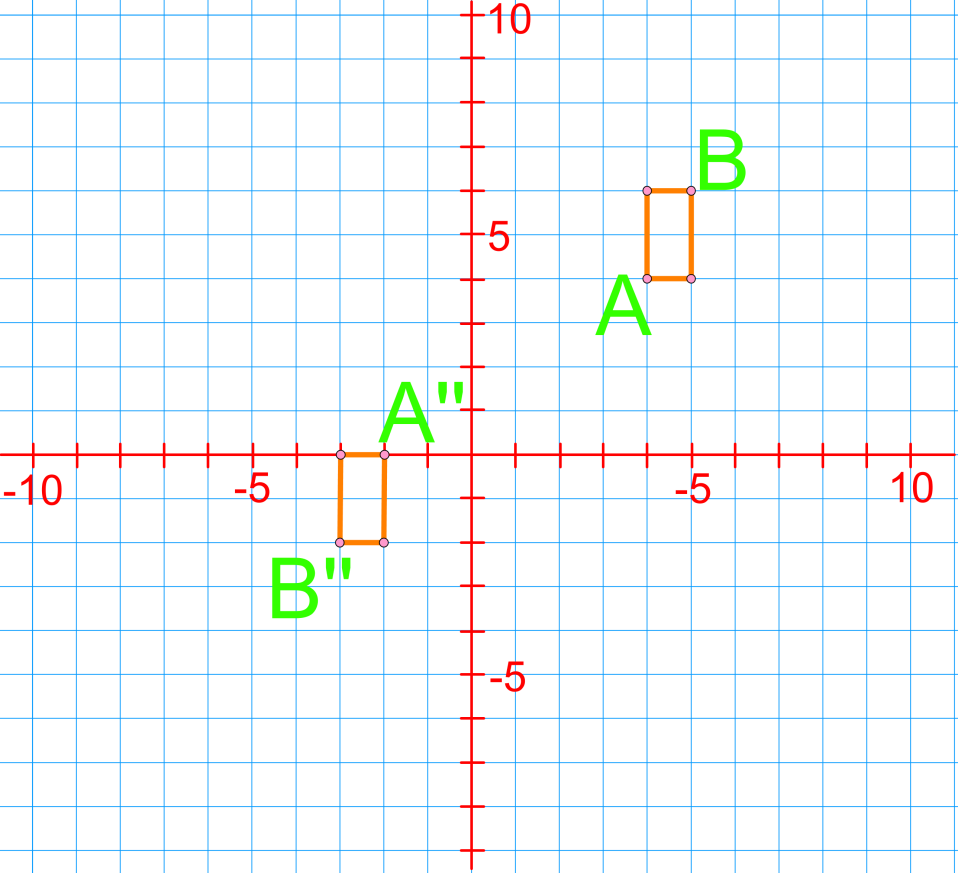
Step 2: Translation
After reflecting the swimmer, the next step is to use a translation to move the swimmer vertically. We need to move the swimmer up along the y-axis to align with the boat's position. Since both the swimmer's new position and the boat are at the same x-coordinate (x = 5), we do not need to move left or right.
To determine how many units to move up, count the number of units from the swimmer's current position to the boat's position. Suppose the swimmer needs to move up 6 units to reach the boat. We will translate the swimmer 6 units up along the y-axis.
Step 3: Final Position
After performing the reflection and translation, the swimmer will have reached the boat. The reflection moved the swimmer to the right side of the y-axis, and the translation moved the swimmer vertically up to the boat's position. By following these steps, we have successfully used translations, reflections, and rotations to get the swimmer to the boat.
Here are some frequently asked questions about transformations on coordinate planes:
-
What are the three main types of transformations on coordinate planes?
The three main types of transformations on coordinate planes are translations, reflections, and rotations. Translations involve moving a shape in any direction without changing its size or orientation. Reflections create a mirror image of a shape across a line. Rotations involve turning a shape around a fixed point.
-
How do you perform a translation on a coordinate plane?
To perform a translation, add the horizontal movement to the x-coordinate and the vertical movement to the y-coordinate of each point. For example, to translate a point (3, 4) by 2 units right and 1 unit up, the new coordinates would be (5, 5).
-
What happens when you reflect a shape over the y-axis?
When reflecting a shape over the y-axis, the x-coordinates of all points change sign while the y-coordinates remain the same. For instance, a point at (3, 2) would be reflected to (-3, 2).
-
How do you rotate a point 90 degrees clockwise around the origin?
To rotate a point 90 degrees clockwise around the origin, swap the x and y coordinates and change the sign of the new y-coordinate. For example, the point (2, 3) rotated 90 degrees clockwise becomes (3, -2).
-
Why is the order important when combining different transformations?
The order of transformations is important because different sequences can lead to different final positions. Generally, translations are performed first, followed by rotations, and then reflections. Changing this order can result in a different final position for the transformed shape.
Understanding horizontal and vertical distances is crucial in mathematics, particularly in geometry and algebra. To fully grasp this concept, it's essential to have a solid foundation in several prerequisite topics. These topics provide the necessary skills and knowledge to comprehend and apply the principles of horizontal and vertical distances effectively.
One fundamental prerequisite is Conics - Circle, which involves moving shapes on the coordinate plane. This topic helps students visualize how objects can be positioned and moved in two-dimensional space, directly relating to the concept of horizontal and vertical distances.
Another important prerequisite is understanding reflection across the x-axis. This concept teaches students how to manipulate shapes and points by flipping them over the x-axis, which is crucial when dealing with vertical distances. Similarly, reflection across the y-axis is equally important, as it relates to horizontal distances and how objects can be mirrored on the coordinate plane.
The study of parallel and perpendicular line equations is particularly relevant when exploring horizontal and vertical distances. This topic helps students understand the relationships between lines and how to calculate the perpendicular distance to a reflection line, which is often a key component in problems involving horizontal and vertical distances.
Lastly, combining transformations of functions is a critical prerequisite. This topic teaches students how to apply multiple transformations to a function or shape, which is often necessary when working with complex problems involving horizontal and vertical distances.
By mastering these prerequisite topics, students will be well-equipped to tackle more advanced concepts related to horizontal and vertical distances. They will have the necessary tools to visualize and manipulate shapes on a coordinate plane, understand reflections and their impact on distances, work with parallel and perpendicular lines, and apply multiple transformations to solve complex problems.
It's important to note that these prerequisites build upon each other, creating a strong foundation for understanding horizontal and vertical distances. For example, the knowledge gained from studying conics and circles can be applied when working with reflections, which in turn helps in understanding parallel and perpendicular lines. This interconnectedness of topics highlights the importance of thoroughly grasping each prerequisite before moving on to more advanced concepts.
In conclusion, a solid understanding of these prerequisite topics is essential for students to fully comprehend and apply the principles of horizontal and vertical distances. By investing time in mastering these foundational concepts, students will be better prepared to tackle more complex problems and applications in geometry and algebra.