Likelihood for simple events

In this lesson, we will learn:

• What is the likelihood of different probability events?
• The likelihood of an event can either be: impossible, unlikely, as likely as not, likely, or certain
• How to understand likelihood using a probability line (probability number line)

Notes:

• The probability of an event is a value of how likely it is to happen. We can quantify the chances of something happening using a probability fraction: $P$(event) = $\frac{number\,outcomes\,wanted}{total\,number\,possibilities}$


• The likelihood of an event can be described using the following phrases:
• Impossible - it will never happen
• Unlikely - low chances of happening (less than half the chances)
• As likely as not - just as likely to happen as to not happen (even chances; exactly half the chances)
• Likely - high chances of happening (more than half the chances)
• Certain - it will always happen (happen for sure)


• How does the value of the probability fraction represent the likelihood of an event?
• It can tell us how likely or unlikely something is to happen.
• The tool we can use to help us understand likelihood is the probability number line (probability line)

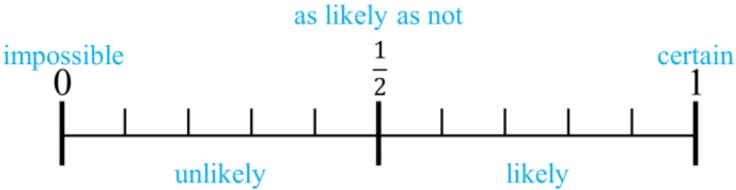
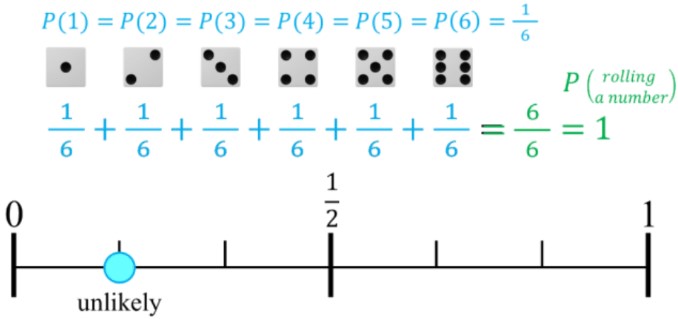
• The probability line goes from 0 to 1 because the smallest probability fraction for any event is 0 out of any number of outcomes. The greatest probability fraction for any event is that it always happens (100% of the time = fraction of 1 whole).


• Ex. For rolling a six-sided die, there is a 1 in 6 chance to land on any numbered side. Rolling any of the six numbers has a low chance (all the outcomes are unlikely events).
• If you add all the probability fractions of all outcomes, the answer will be 1 whole (considering every single possible result)