Rules for adding integers
You see numbers in your everyday life all the time. Numbers can be either positive or negative. When you see a number written without a "+" or "-" sign, that is, without a sign, it usually means that the number is positive. For example, if you saw the number 6 exactly the way we wrote it here, it means positive 6.
Adding positive numbers involve just simple addition, and subtracting them is just simple subtraction.
How to add negative numbers
When we talk about negative numbers, things get a bit more difficult. A negative number has a "-" sign in front of it. For example, -6 would mean negative 6.
What happens when we try to add two negative numbers? Two negatives actually make a positive! When you see two minus signs next to each other, you'll know to add it instead. Why does it work like this? Let's take an example dealing with your bank account.
If you've got $10 in the bank, but the bank accidentally removed $5, it'd look like: $10 - $5 = $5. You'd be left with $5 in your account. Since it was the bank's mistake and they want to take away a mistake (the -$5), it'll look like $5 - (-$5) = $5 + $5 = $10. They're remove the negative (the mistake in this case), which in the end, makes it a positive, so you'll end up with $10 in your bank again.
An example of two negatives in real life can be seen through double negatives when we're speaking. If we told you to not cross the road, it would mean just that. But if we told you to not not cross the road, it means you should cross the road. In a similar sense, you can use this to help you when adding negative numbers.
A good rule of thumb is that when you see two like signs, add the numbers together (i.e. two "-" signs, or two "+" signs). If you see unlike signs (i.e. one "-" and one "+"), it becomes a negative and you'll have to subtract it.
Adding and subtracting integers examples
Let's try some number line examples to see how adding and subtracting positive and negative integers work.Question 1:
What addition statement does each of the following diagrams represent? Then, find the sum.
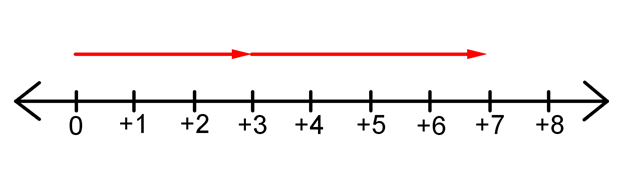
Solution:
In this question, the first arrow = +3.
The second arrow = +4.
This is an addition statement and the final sum of the numbers gives us 7.
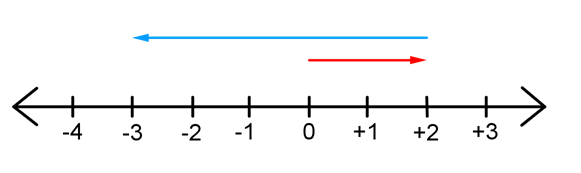
Question 2:
Use a number line to add
(+2) + (+4)=
Solution:
The first term we have is a +2. This means that the first arrow we'd draw will represent +2.
The second arrow we draw represents +4.
Then we'll get the final answer of landing on 6 on the number line.
Question 3:
Use a number line to add
(-8) + (-3)=
Solution:
Our first arrow represents -8
The second arrow we will draw represents -3
In the end, we'll get a total of -11.
If you need to check your calculations on positive and negative integer calculation, try out this online calculator.
Looking for more topics to review? Learn what the order of operations are with PEMDAS, and how to deal with subtraction and addition of decimal numbers. There are models to help you add and subtract fractions as well such as adding fractions with like denominators. You can also try your hand at divisibility rules that can help you find factors of whole numbers.
In this lesson, we will learn:
- Adding 1-digit Numbers Vertically – Same Signs
- Adding 1-digit Numbers Vertically – Opposite Signs
- Adding 2-digit Numbers Vertically
Notes:
- Two like signs produce a positive sign.
- Two unlike signs produce a negative sign. The order of the integers is interchangeable.
- If units add up to 10 or more, we carry the 10 to the next digit by adding 1 to it.
- To add two numbers with LIKE signs, first we add up the two numbers regardless of the sign, then prefix the number with the sign they share.
- To add two numbers with UNLIKE signs, we re-order the integers so that the integer with the higher magnitude goes first, then subtract the numbers regardless of the signs, lastly we keep the sign of the number with the higher magnitude.