Reflections and rotations of shapes

In this lesson, we will learn:

• How to draw reflections on a grid with vertical, horizontal, or diagonal mirror lines
• How to find order of rotational symmetry for shapes with multiple symmetry lines
• How to rotate shapes around a point 90°, 180°, 270°, and 360° clockwise or counter-clockwise

Notes:

• For line symmetry, a mirror test check if reflected halves match

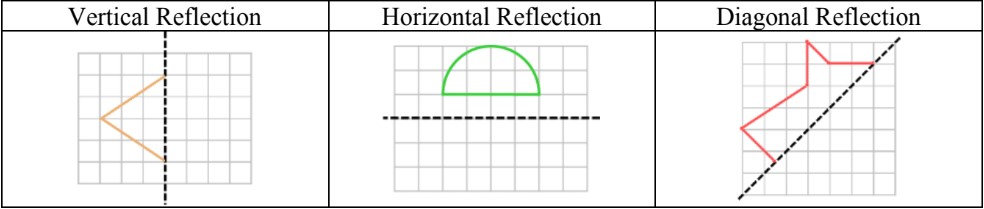
• When drawing reflections of shapes, a grid and a line of symmetry are used
• For reflections, the line of symmetry will be called the “mirror line” instead
• There can be vertical, horizontal, and diagonal mirror lines

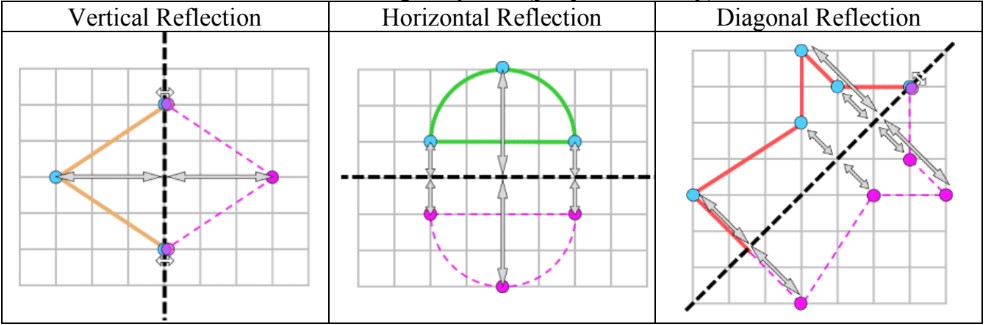
• Choose some exact points of the original shape on the grid
• To draw the reflection of these points, they must be the same distance away from the mirror line as the original points (perpendicularly)

• Another type of symmetry is called rotational symmetry
• If a shape has multiple lines of symmetry: when you spin it around in a full circle (360°), it will look exactly the same more than once

Ex. a triangle has 3 lines of symmetry; rotating a full circle, it matches the original 3 times

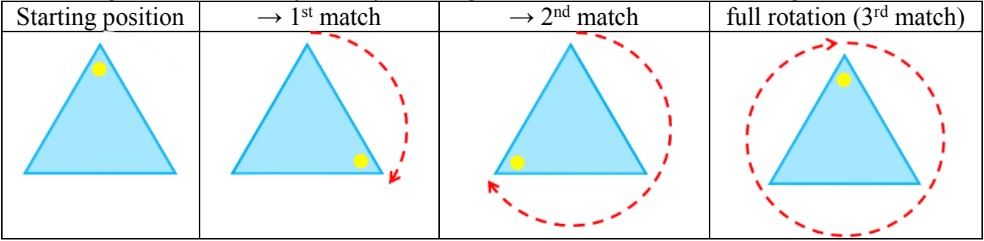
• Therefore, the order of rotational symmetry for this regular triangle is = 3

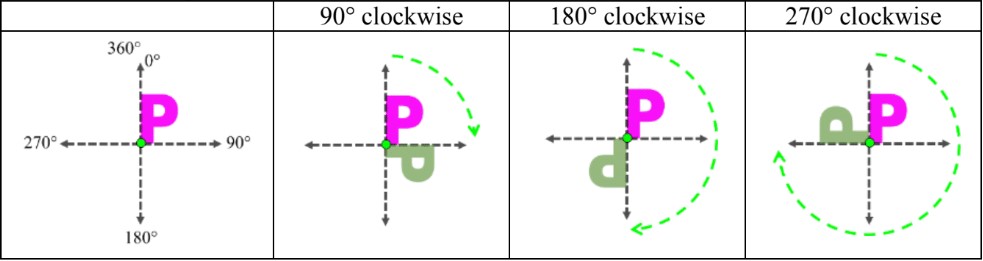
• When rotating shapes, the shape will be spun around a point (usually the middle):
• either clockwise or counter-clockwise
• To one of 4 main rotation angles: 90°, 180°, 270°, and 360°

Ex. Rotate the shape of the letter “P” clockwise 90°, 180°, and 270° around the point