When you are asked to make an object bigger or smaller, you will ask, "by how much?" In this case, scale factor can help answer the question. In this lesson, we will practice how to enlarge and reduce objects according to the scale factors given to us.
Intros
0/1 watched
Examples
0/6 watched
Introduction to Scale Factors in Enlargements and Reductions
Welcome to our lesson on scale factors in enlargements and reductions. This fundamental concept in geometry plays a crucial role in various real-world applications. Our introduction video provides a visual demonstration of how scale factors work, making it easier to grasp this important mathematical principle. Throughout this lesson, we'll explore how to use scale factors to enlarge and reduce objects accurately. You'll learn to manipulate shapes and figures, maintaining their proportions while changing their size. Understanding scale factors is essential for fields like architecture, engineering, and graphic design. We'll cover both enlargement, where objects become larger, and reduction, where they become smaller. By the end of this lesson, you'll be able to confidently apply scale factors to various geometric problems, enhancing your spatial reasoning skills and mathematical proficiency.
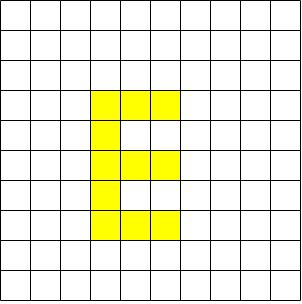
Redraw the letters below using a scale factor of 2.
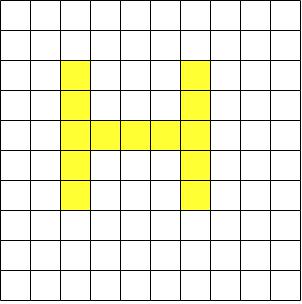
Step 1: Understanding the Scale Factor
To begin with, it's important to understand what a scale factor is. A scale factor is a number which scales, or multiplies, some quantity. In this case, we are using a scale factor of 2, which means that every dimension of the letter will be doubled. This includes both the height and the width of each part of the letter.
Step 2: Analyzing the Original Letter
Let's take a close look at the original letter 'H' in the image. The letter is composed of three main parts: two vertical lines and one horizontal line in the middle. Each of these parts has specific dimensions. For instance, if the height of the vertical lines is 5 units and the width is 1 unit, these measurements will be crucial for our scaling process.
Step 3: Applying the Scale Factor to the Height
First, we need to apply the scale factor to the height of the letter. If the original height of the vertical lines is 5 units, multiplying this by the scale factor of 2 gives us a new height of 10 units. This means that the new vertical lines will be twice as tall as the original ones.
Step 4: Applying the Scale Factor to the Width
Next, we apply the scale factor to the width of the letter. If the original width of the vertical lines is 1 unit, multiplying this by the scale factor of 2 gives us a new width of 2 units. Similarly, the width of the horizontal line in the middle will also be doubled from 1 unit to 2 units.
Step 5: Redrawing the Vertical Lines
Now that we have the new dimensions, we can start redrawing the letter. Begin with the vertical lines. Each vertical line will now be 10 units tall and 2 units wide. Draw two vertical lines with these new dimensions, ensuring they are parallel and spaced appropriately to maintain the structure of the letter 'H'.
Step 6: Redrawing the Horizontal Line
Next, redraw the horizontal line in the middle of the letter. The new horizontal line will be 2 units wide and will span the distance between the two vertical lines. Ensure that this line is positioned in the middle of the vertical lines to accurately represent the letter 'H'.
Step 7: Final Adjustments and Verification
After redrawing all parts of the letter with the new dimensions, take a moment to verify your work. Ensure that all parts of the letter have been scaled correctly and that the overall structure of the letter 'H' is maintained. The new letter should be twice as large in both height and width compared to the original.
Conclusion
By following these steps, you can accurately redraw the letter 'H' using a scale factor of 2. This process can be applied to any letter or shape, allowing you to scale it up or down as needed. Understanding and applying scale factors is a fundamental skill in geometry and can be very useful in various mathematical and real-world applications.
Here are some frequently asked questions about enlargements and reductions with scale factors:
1. How do you find the scale factor of an enlargement or reduction?
To find the scale factor, divide a dimension of the new shape by the corresponding dimension of the original shape. For example, if a line in the original shape is 5 cm and the corresponding line in the new shape is 15 cm, the scale factor is 15 ÷ 5 = 3.
2. What is the enlargement and reduction of scale?
Enlargement occurs when the scale factor is greater than 1, making the new shape larger. Reduction happens when the scale factor is between 0 and 1, resulting in a smaller shape. Both transformations maintain the original shape's proportions.
3. How do you find the scale factor and enlargement?
To find the scale factor, compare corresponding dimensions of the original and new shapes. For enlargement, multiply the original dimensions by the scale factor. For example, if the scale factor is 2, a 5 cm side becomes 10 cm in the enlarged shape.
4. Is scale factor 0.5 an enlargement?
No, a scale factor of 0.5 is a reduction. It means the new shape is half the size of the original. Any scale factor between 0 and 1 results in a reduction, while factors greater than 1 create enlargements.
5. How does scale factor affect area and volume?
The area changes by the square of the scale factor, while volume changes by its cube. For example, with a scale factor of 2, the area increases by 2² = 4 times, and the volume increases by 2³ = 8 times.
Understanding enlargements and reductions with scale factors is a crucial concept in geometry and mathematics. However, to fully grasp this topic, it's essential to have a solid foundation in several prerequisite areas. These fundamental concepts not only provide the necessary background knowledge but also enhance your ability to apply scale factors effectively.
One of the key prerequisites is calculating angles and sides using sine ratio. This trigonometric skill is vital when dealing with scaled figures, especially in complex shapes where angles play a significant role. Similarly, calculating angles and sides using cosine ratio complements this knowledge, providing a comprehensive understanding of trigonometric relationships in scaled figures.
The applications of polynomial functions are also relevant to enlargements and reductions. These functions can describe how dimensions change with different scale factors, offering a mathematical model for scaling operations. Understanding polynomials helps in analyzing the effects of scaling on various geometric properties.
When working with three-dimensional objects, knowledge of the surface area of 3D shapes becomes crucial. Scaling affects surface area differently than it does volume, and understanding this relationship is key to solving problems involving enlarged or reduced 3D objects.
Additionally, familiarity with common factors of polynomials can be beneficial when simplifying expressions related to scale factors, especially in more advanced problems where algebraic manipulation is required.
Lastly, understanding calculating perimeter and area of complex polygons is essential. Scale factors directly impact these measurements, and being able to work with intricate shapes allows for a more comprehensive application of enlargement and reduction concepts.
By mastering these prerequisite topics, you'll build a strong foundation for understanding enlargements and reductions with scale factors. This knowledge will enable you to tackle more complex problems, visualize scaled figures accurately, and apply these concepts in real-world scenarios. Remember, each of these topics contributes uniquely to your overall comprehension, forming a interconnected web of mathematical knowledge that enhances your problem-solving skills in geometry and beyond.