In this lesson, we will learn:
- To recall the definition of electrolysis and some examples of its use.
- To draw an electrolytic cell used in electrolysis.
- How to find electrolysis operating conditions using cell potential calculations.
- How to use Faraday’s law to relate charge flow to reaction stoichiometry.
Notes:
- Electrolysis is the breaking up (-lysis) of substances using electric current (electro-) – hence the name.
It can be done with dissolved or molten ionic solutions such as sodium chloride (NaCl) or aluminum oxide (Al2O3) to obtain the pure elements from their ions. This is very useful because some valuable substances like aluminum metal are not found naturally in their elemental form because they are too reactive. They must be produced from their ions.
In terms of energy, electrolysis reactions are the opposite of a redox cell reaction:- In a redox or galvanic cell, the reaction is spontaneous and chemical potential energy is used to produce electrical energy. In terms of thermodynamics, Gibbs free energy of the system drives the forward reaction to make products.
- In an electrolysis reaction, the process is not spontaneous, so electrical energy is needed for the reaction to make products. In terms of thermodynamics, the forward reaction results in a loss of Gibbs free energy of the surroundings, so it is unfavourable.
- Electrolysis is often used on binary salts – containing only two different ions – in order to obtain the constituent ions as their pure elements. This is how NaCl is electrolyzed to obtain chlorine gas (Cl2) and sodium which reacts to form NaOH, another important chemical.
Because only two oppositely charged ions are involved with binary salts, both ions will take part in the opposite processes of reduction and oxidation to return to their elemental form. When binary salts are electrolyzed, there are a few differences to the cell compared to redox (galvanic) cells:- Because the compounds being electrolyzed are water-soluble, inert electrodes are used, normally carbon or platinum.
- No salt bridge is necessary. This is because electrolyte can mix as there’s usually only one electrolyte; the ionic substance that is being electrolyzed! It is in aqueous or molten form so a current can flow easily.
See the diagram below: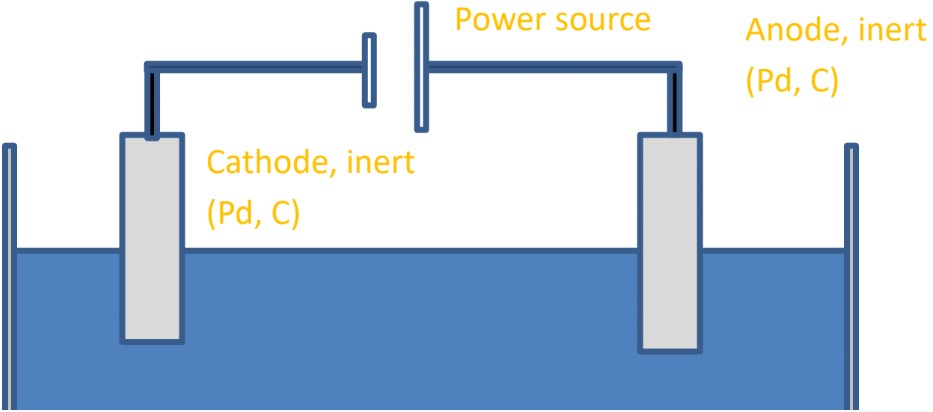
- As said above, electrolysis reactions are not spontaneous!
This means that instead of the cells producing electricity, electrolysis cells need voltage to be applied. You can calculate the voltage just like you calculate redox cell potential in Calculating cell potential. Your calculated E0cell should be less than zero for an electrolytic cell.
For example, with sodium chloride, NaCl, being electrolyzed to produce sodium and chlorine:
Na+ + e-→Na E0red = -2.71 V
Cl2 + 2e-→2Cl- E0red = +1.36 V
NaCl electrolysis reduces Na+ to Na metal and oxidizes Cl- ions to Cl2 so the Cl half-equation and E0red value needs reversing. Applying this to the cell potential equation:E0cell = E0red (reduction) + E0ox (oxidation)
We can work out:E0cell = -2.71 + (-1.36) = -2.71 -1.36 = -4.07 V
This means that at least 4.07 V must be applied to the cell for the reaction to start occurring spontaneously. Higher voltage is applied usually because there is resistance in the cell and it is not at standard conditions. - Remember that electrolysis in aqueous solution means in water – the water solvent can be reduced or oxidized itself. Taking the example of NaCl again, in aqueous solution there are four possible reactions going on in the cell, each with different reduction potentials.1 Assuming neutral pH:
- The oxidation of chlorine: 2Cl-→Cl2 + 2e- where E0ox = -1.36 V
- The oxidation of water: 2H2O (l) → O2 + 4e- + 4 H+(aq) where E0ox = -1.23 V
- The reduction of water: 2H2O (l) + 2e-→H2 (g) + 2OH- (aq) where E0red = -0.83 V
- The reduction of sodium: Na+(aq) + e-→Na where E0red = -2.71 V
If we applied 4.07 V to the system, what would react? The reaction that is preferable is the one with the least required voltage! Like a spontaneous redox reaction, the half-equations with the greatest E0red and E0ox are preferred.
‘Preferred’ means this reaction is much more likely to happen. The other reaction(s) can occur but are less likely to.
One reduction and one oxidation ‘half reaction’ must happen. Think about the voltage differences (potential difference!) between the half-equations:- The reduction potential of water is less negative than sodium metal, so only water gets reduced to hydrogen gas at the cathode (where reduction occurs).
- The oxidation potential for water is less negative than chlorine but they are very similar.
The real process of NaCl electrolysis is this:2 NaCl (aq) + 2H2O(l)→2Na+ (aq) + 2OH- (aq) + H2 (g) + Cl2 (g)
Chlorine gas (Cl2) is obtained, as is hydrogen gas and NaOH which can both be isolated and sold. - You need to look at the conditions that occur in your electrolytic cell. When you have a substance to be electrolyzed, check the ions that will be produced:
- If H+ is produced, your conditions will be acidic.
- If OH- is made, it will be basic.
- If neither, assume it is neutral.
2OH- + Br-→H2O + BrO- + 2e- E0 = +0.76 V
This half-reaction could only occur in basic conditions. - As electrical energy is either used up, as in electrolytic cells, or generated in voltaic cells, we can measure the flow of electrons (the current) using an ammeter.
Faraday’s law relates this current to the stoichiometry and rate of a reaction: - = charge (in coulombs)
- = current (in amps)
- = time (seconds)
- In other words, the higher the current, the more charge per unit time passes through the cell and the faster a reaction at an electrode will occur (e.g. more Cu (s) being deposited, gaining mass, or a Ni (s) electrode being oxidised, losing mass).
- The mass change of a copper electrode during an experiment;
- The number of moles of copper this mass equates to;
- The number of moles of electrons liberated;
- The amount of charge this amount of electrons carries
- The time taken or the current reading of the cell.
- = mass change of substance at electrode (in g)
- = electric charge (Coulombs)
- = molar mass of substance at electrode
- = the Faraday constant, 96485 C mol-1
- = the number of electrons transferred per ion (e.g. 2 for Ca2+ ions).
- WORKED EXAMPLE: Using Faraday’s laws.
Calculate how long a 7A current must be applied to deposit 1.5 g of Zn metal at an electrode.
We are solving for time, so rearranging this equation for t gives us this:
Where:
This equation is important because when rearranged for , we measure the current as a rate of charge over time. Quantity of charge is proportional to quantity of mass change at an electrode.
This is where the value of the Faraday constant comes from – 96485 C mol-1. It means that one mole of electrons carries 96485 Coulombs of charge. For example, if a copper electrode was being oxidised in the half-reaction:
We know that one mole of copper liberates two moles of electrons, which has 2 × 96485 C of charge. Since moles are related to the mass of a sample substance, we now have a relationship between:
Faraday’s laws of electrochemistry can be summarized by the following:
Where:
As the equation above shows, increasing q will increase the rate of deposition (Mn+ (aq) ions becoming M (s) at the electrode) or liberation, the reverse process.
Substituting q for in the equation above gives:
This relates all the factors mentioned above.
We have the values m = 1.5; F = 96485; z = 2 (for 2e- per Zn2+ ion); I = 7; M = 65.38.
With so many variables, a good way to ‘proof’ your answer is to solve for the units. We are solving for t, so our units should end in s (seconds).
The calculation shows that it takes ten and a half minutes for this much of a mass change to take place. Alternatively, you can use the conversion factor method to go from mass to time:
Whichever method you use, we received the same answer because the units cancel!
1 Sourcefor data: ATKINS, P. W., & DE PAULA, J. (2006).Atkins' Physical chemistry. Oxford, Oxford University Press






