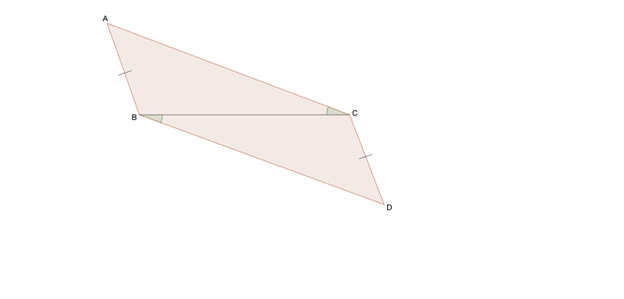
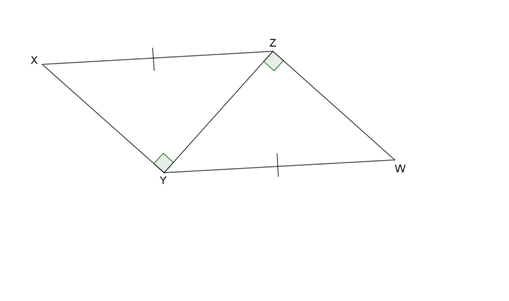
Introduction to Congruency and Similarity in Triangles
Welcome to our exploration of congruency and similarity in triangles! These concepts are fundamental in geometry and have wide-ranging applications. Congruency refers to triangles that are identical in shape and size, while similarity describes triangles with the same shape but potentially different sizes. Our introduction video is an essential starting point, offering a clear visual explanation of these concepts. It will help you grasp the key differences and similarities between congruent and similar triangles. Understanding these principles is crucial for solving more complex geometric problems. As we delve deeper, you'll see how congruency involves equal angles and sides, while similarity focuses on proportional sides and equal angles. This knowledge forms the foundation for many geometric proofs and real-world applications in fields like architecture and engineering. Let's begin this exciting journey into the world of triangles, where you'll discover how these seemingly simple shapes hold powerful mathematical truths!