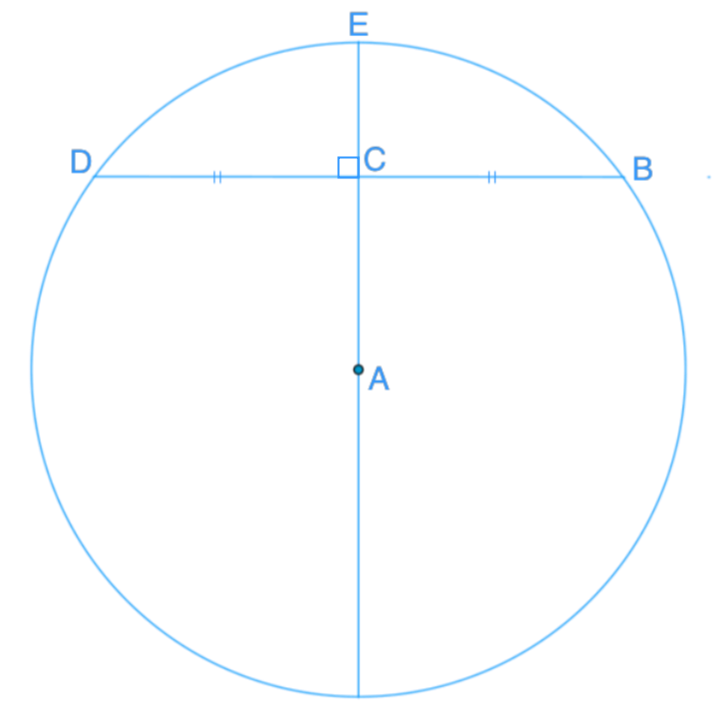
- If AC is 4 cm and BD is 15 cm, what is the diameter of the circle?
- Zoe wants to set up her round dining table by putting a candle in the centre of it. How can she do that by using a tangent? Explain your answer with a diagram.
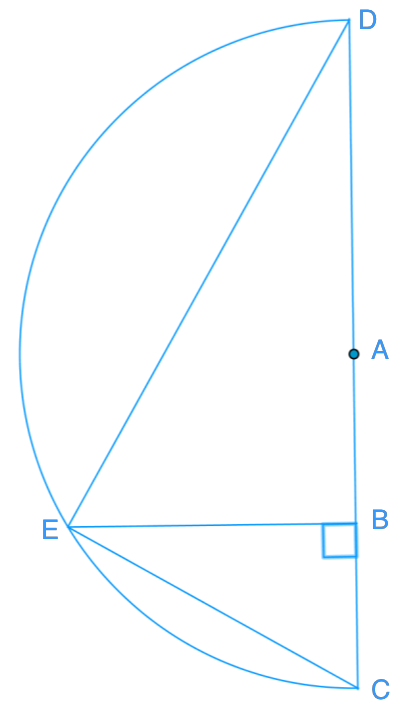
- Below is a segment of a circle, and A is the center of the circle. The radius is 15 cm, BC is 7.5 cm, and DE is 24 cm.
Determine.
Free to Join!
Easily See Your Progress
 We track the progress you've made on a topic so you know what you've done. From the course view you can easily see what topics have what and the progress you've made on them. Fill the rings to completely master that section or mouse over the icon to see more details.
We track the progress you've made on a topic so you know what you've done. From the course view you can easily see what topics have what and the progress you've made on them. Fill the rings to completely master that section or mouse over the icon to see more details.Make Use of Our Learning Aids
Earn Achievements as You Learn
 Make the most of your time as you use StudyPug to help you achieve your goals. Earn fun little badges the more you watch, practice, and use our service.
Make the most of your time as you use StudyPug to help you achieve your goals. Earn fun little badges the more you watch, practice, and use our service.Create and Customize Your Avatar
 Play with our fun little avatar builder to create and customize your own avatar on StudyPug. Choose your face, eye colour, hair colour and style, and background. Unlock more options the more you use StudyPug.
Play with our fun little avatar builder to create and customize your own avatar on StudyPug. Choose your face, eye colour, hair colour and style, and background. Unlock more options the more you use StudyPug.
Introduction to Chord Properties in Circles
Welcome to our exploration of chord properties in circles! This fascinating topic in geometry is essential for understanding the relationships between lines and circular shapes. Our introduction video serves as an excellent starting point, providing a visual and interactive way to grasp these concepts. As we delve into chords, you'll discover how they intersect circles and the unique properties they possess. We'll examine how chords relate to the circle's center, radius, and circumference, uncovering mathematical patterns that have intrigued scholars for centuries. Whether you're a math enthusiast or just beginning your geometry journey, understanding chord properties will enhance your problem-solving skills and spatial reasoning. The video will guide you through key definitions, theorems, and practical applications, making complex ideas accessible and engaging. So, let's embark on this circular adventure together and unravel the mysteries of relationships between lines and circles in geometry!
Below is a segment of a circle, and A is the center of the circle. The radius is 15 cm, BC is 7.5 cm, and DE is 24 cm.

Determine.
∠CED
Step 1: Understand the Problem
First, we need to understand the given information and what we are required to find. We have a circle with center A and a radius of 15 cm. The chord BC is 7.5 cm, and the chord DE is 24 cm. We are asked to determine the angle ∠CED.
Step 2: Identify the Center and Radius
Identify the center of the circle, which is point A. The radius of the circle is given as 15 cm. This means that any line segment from the center A to any point on the circumference of the circle is 15 cm long.
Step 3: Analyze the Chords
We are given two chords: BC and DE. The length of BC is 7.5 cm, and the length of DE is 24 cm. These chords are segments within the circle, and their lengths are provided to help us understand the geometry of the circle.
Step 4: Determine the Diameter
Since the radius is 15 cm, the diameter of the circle is twice the radius, which is 30 cm. This is important because any angle inscribed in a semicircle (formed by the diameter) is a right angle (90 degrees).
Step 5: Identify the Inscribed Angle
We need to determine the angle ∠CED. This angle is formed by the points C, E, and D. Since DE is a chord passing through the center A, it forms a diameter of the circle.
Step 6: Apply the Inscribed Angle Theorem
According to the Inscribed Angle Theorem, any angle inscribed in a semicircle is a right angle. Since DE is the diameter, the angle ∠CED, which is inscribed in the semicircle, must be 90 degrees.
Step 7: Conclusion
Based on the Inscribed Angle Theorem and the given information, we can conclude that the angle ∠CED is 90 degrees. This is because any angle inscribed in a semicircle formed by the diameter of the circle is always a right angle.
Q1: What is a chord in a circle?
A: A chord is a line segment that connects two points on the circumference of a circle. It doesn't necessarily pass through the center of the circle. The diameter is a special chord that passes through the center and is the longest possible chord in a circle.
Q2: How does the perpendicular bisector of a chord relate to the center of the circle?
A: The perpendicular bisector of a chord always passes through the center of the circle. This property is crucial for finding the center of a circle when given two chords, as the intersection of their perpendicular bisectors will be the center point.
Q3: What is the relationship between an inscribed angle and the central angle that subtends the same arc?
A: An inscribed angle is always half the measure of the central angle that subtends the same arc. This relationship can be expressed as: Inscribed Angle = 1/2 × Central Angle. This property is fundamental in solving many circle geometry problems.
Q4: How does the length of a chord relate to its distance from the center of the circle?
A: The length of a chord is inversely related to its distance from the center of the circle. Chords closer to the center are longer, while those farther from the center are shorter. This relationship can be expressed mathematically using the Pythagorean theorem: c² = 4(r² - d²), where c is the chord length, r is the radius, and d is the distance from the center to the chord.
Q5: What is the intersecting chords theorem and how is it applied?
A: The intersecting chords theorem states that when two chords intersect inside a circle, the product of the lengths of the segments of one chord equals the product of the lengths of the segments of the other chord. Mathematically, if chords AB and CD intersect at point E, then AE × EB = CE × ED. This theorem is useful in solving problems involving intersecting lines within circles, such as in pizza cutting scenarios or geometric constructions.
To fully grasp the concept of chord properties in geometry, it's crucial to have a solid foundation in several prerequisite topics. Understanding these fundamental concepts will greatly enhance your ability to comprehend and apply chord properties effectively.
One of the key prerequisites is the relationship between two variables. This concept is essential when exploring the relationships between lines and circular shapes, which are integral to chord properties. By mastering this algebraic concept, you'll be better equipped to analyze the geometric relationships within circles.
Another important topic to grasp is the introduction to nonlinear equations. Chord properties often involve circular equations, which are nonlinear in nature. Familiarity with these equations will help you understand the mathematical representations of chords and their relationships to circles.
The study of perpendicular bisectors is particularly relevant when examining chord properties. Perpendicular bisectors of chords play a significant role in understanding the symmetry and relationships within circles, making this concept crucial for a comprehensive understanding of chord properties.
Trigonometry also plays a part in chord properties, especially when dealing with angles and lengths. The ability to use sine ratios to calculate angles and sides is valuable, particularly when working with congruent right triangles formed by chords and radii.
Perhaps one of the most directly related topics is central and inscribed angles in circles. This concept is fundamental to understanding chord properties, as it deals with the relationships between angles formed by chords and the circle itself. Mastering this topic will provide you with essential insights into chord behavior and properties.
Lastly, the applications of the Pythagorean theorem are indispensable when studying chord properties. This theorem is frequently used to calculate chord lengths and their relationships to the circle's radius, making it a vital tool in your geometric toolkit.
By investing time in understanding these prerequisite topics, you'll build a strong foundation for exploring chord properties. Each concept contributes uniquely to your overall comprehension, allowing you to approach chord-related problems with confidence and clarity. Remember, mathematics is interconnected, and mastering these fundamental topics will not only aid in understanding chord properties but also enhance your overall mathematical proficiency.



