Sign In
Sign Up
flag-uk

Unlock the Secrets of Consumption, Saving Plans & Marginal Propensity
Dive into essential economic concepts with our clear, visual explanations. Understand how spending and saving change with income, and gain valuable insights into personal finance and broader economic trends.

  1. Intros0/3 watched
  1. 0/3
Now Playing:Consumption and savings plan and marginal propensity – Example 0a
Intros
0/3 watched
  1. Consumption & Savings Function
    • Factors of Consumption and Savings
    • Disposable Income
    • 45 Degree Line
    • Consumption & Savings Functions
  2. Marginal Propensities
    • Marginal Propensity to Consume
    • Change in Consumption and Disposable Income
    • Marginal Propensity to Save
    • Change in Savings and Disposable Income
Consumption & saving plans, and marginal propensity
Jump to:NotesConceptFAQsPrerequisites
Notes

In this chapter, we are going to focus on the Keynesian model.

In this model, we look at aggregate expenditure, which is the sum of consumption expenditure, investment, government expenditure, and exports minus imports. We want to see how this is linked to real GDP.

Note: This section focuses mostly on the consumption expenditures

Consumption & Savings Function

There are a few factors that impact consumption expenditures and savings:
  1. Disposable income
  2. Real interest rate
  3. Wealth
  4. Expected future income

Disposable Income is equal to aggregate income subtracted by taxes and added by transfer payments.

In this section, we will keep real interest, wealth, and expected future income constant. By doing so, we can find the relationship between consumption expenditure and disposable income.

45° Line: is the line y=xy = x on the graph.

Consumption & Savings Plans, and Marginal Propensity


You will see this line when explaining other functions.

Consumption Function: is the relationship between consumption expenditures and disposable income.

Consumption & Savings Plans, and Marginal Propensity


Notice from the graph:
  1. Function is a line that is upward sloping
  2. If the function is below the 45° line, then were saving
  3. If the function is above the 45° line, then were dissaving.

Saving Function: is the relationship between saving plans and disposable income.

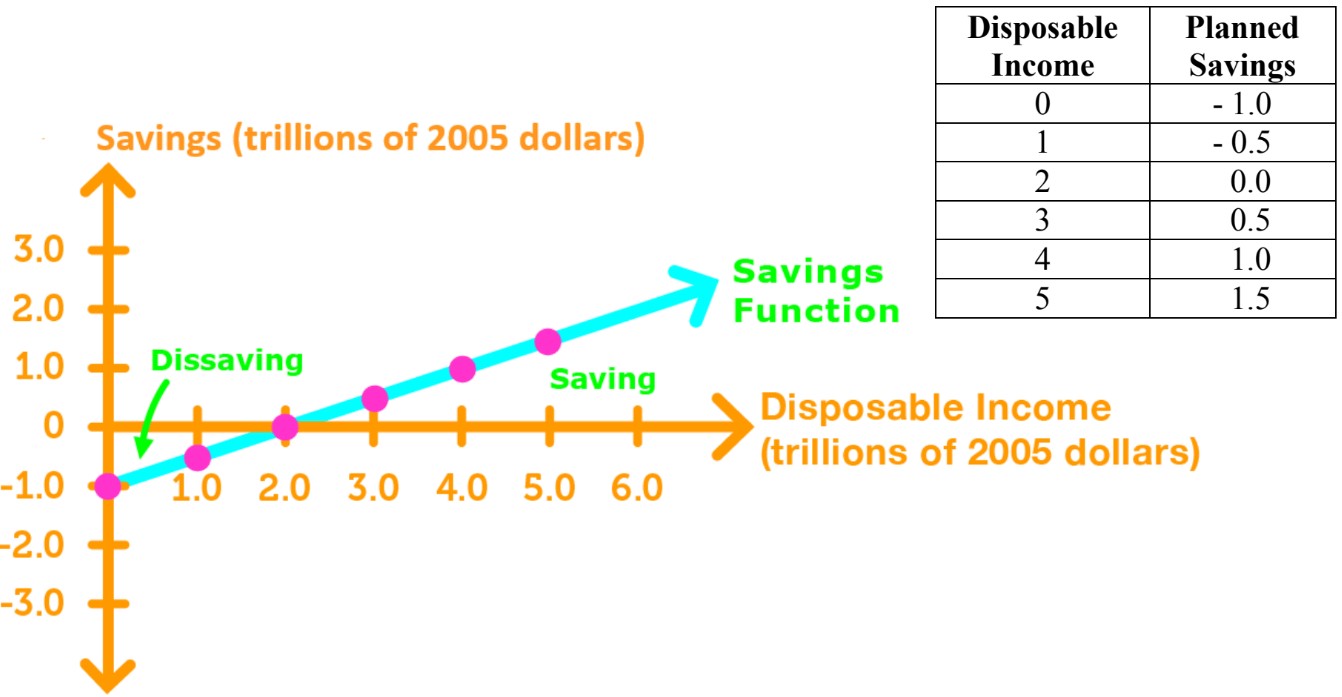
Consumption & Savings Plans, and Marginal Propensity


Notice from the graph:
  1. Function is a line that is upward sloping.
  2. If the function is above the xx-axis, then were saving
  3. If the function is below the xx-axis, then were dissaving.

Marginal Propensities

There are two marginal propensities we will be looking at

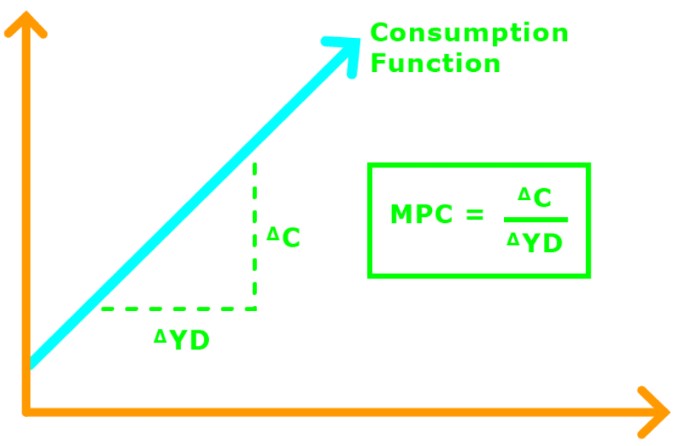
  1. Marginal Propensity to Consume (MPC): is the portion of the change in disposable income which will be used on consumption. To calculate this, we use the following formula

    MPC=ΔCΔYDMPC = \frac{\Delta C} {\Delta YD}

    Where:
    ΔC\Delta C = change in consumption
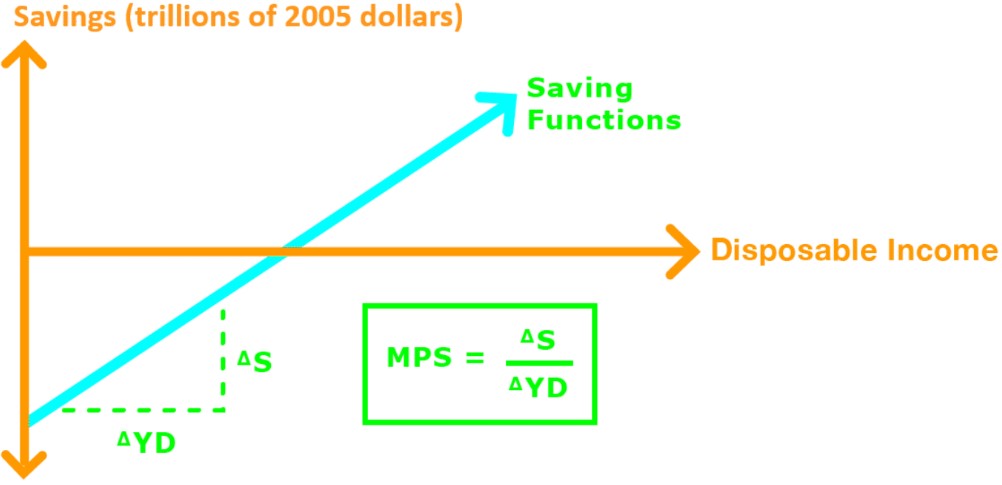
    ΔYD\Delta YD = change in disposable income

  2. Marginal Propensity to Save (MPS): is the portion of the change in disposable income which will be saved. To calculate this, we use the following formula

    MPS=ΔSΔYDMPS = \frac{\Delta S} {\Delta YD}

    Where:
    ΔS\Delta S = change in savings
    ΔYD\Delta YD = change in disposable income

Property of Marginal Propensity: since disposable income is only used for either saving or consumption, then it must be true that

ΔC+ΔS=ΔYD\Delta C + \Delta S = \Delta YD

Dividing both sides of the equation by ΔYD\Delta YD gives

ΔCΔYD+ΔSΔYD= \frac{\Delta C} {\Delta YD} + \frac{\Delta S} {\Delta YD} = 1

Since we know that MPC=ΔCΔYDMPC = \frac{\Delta C} {\Delta YD} and MPS=ΔSΔYDMPS = \frac{\Delta S} {\Delta YD} , then

MPC+MPS= MPC + MPS = 1


Applications of Marginal Propensities

Marginal propensities can be applied to a few things

  1. Consumption Function: the marginal propensity to consume is the slope of the consumption function.

    Consumption & Savings Plans, and Marginal Propensity

  2. Saving Function: the marginal propensity to save is the slope of the savings function.

    Consumption & Savings Plans, and Marginal Propensity

  3. Marginal Propensity to Import: is the portion of the change in real GDP that is spent on imports. To calculate this, we use the following formula

MPM=ΔMΔYMPM = \frac{\Delta M} {\Delta Y}


Where:
ΔM\Delta M = change in imports
ΔY\Delta Y = change in income
Concept

Introduction to Consumption, Saving Plans, and Marginal Propensity

Welcome to our exploration of key economic concepts! In this section, we'll dive into consumption functions, savings functions, and marginal propensities. These fundamental ideas are crucial for understanding how individuals and economies manage their resources. The consumption function shows how spending changes with disposable income, while the savings function illustrates how much people save at different income levels. Marginal propensity, on the other hand, reveals how much of each additional dollar earned is spent or saved. Our introduction video provides a clear, visual explanation of these concepts, making them easier to grasp. As we progress, you'll see how these functions interact and influence economic decisions. Whether you're a student or just curious about economics, understanding these concepts will give you valuable insights into personal finance and broader economic trends. Let's start this journey together and unravel the mysteries of consumption, savings, and marginal propensities!

FAQs

  1. What is the difference between the consumption function and the savings function?

    The consumption function shows how much people spend at different income levels, while the savings function shows how much they save. The consumption function is typically represented as C = a + bY, where 'a' is autonomous consumption and 'b' is the marginal propensity to consume. The savings function is S = -a + (1-b)Y. They are complementary, as what's not consumed is saved.

  2. How does the marginal propensity to consume (MPC) affect economic policy?

    The MPC significantly influences the effectiveness of fiscal policies. A higher MPC means that consumers spend more of each additional dollar of income, leading to a larger multiplier effect. This makes fiscal stimulus measures, such as tax cuts or increased government spending, more effective in boosting economic activity. Policymakers consider the MPC when designing economic interventions to maximize their impact.

  3. What factors can influence an individual's marginal propensity to save (MPS)?

    Several factors can affect the MPS, including real interest rates, wealth, expected future income, economic uncertainty, and cultural factors. Higher real interest rates tend to increase MPS as saving becomes more rewarding. Economic uncertainty often leads to higher MPS as people save more for precautionary reasons. Cultural norms and personal financial goals also play a significant role in determining an individual's saving behavior.

  4. How does the concept of marginal propensity apply to international trade?

    In international trade, the marginal propensity to import (MPM) is a key concept. It measures how much of each additional dollar of national income is spent on imports. A higher MPM indicates that a larger portion of income increases goes towards buying foreign goods and services. This concept is crucial for understanding trade balances and the impact of economic growth on a country's import demand.

  5. Can marginal propensities change over time, and what are the implications?

    Yes, marginal propensities can change over time due to various economic, social, and demographic factors. Changes in MPC or MPS can have significant implications for economic forecasting and policy effectiveness. For example, a declining MPC over time might signal more cautious consumer behavior, potentially leading to slower economic growth. Policymakers and businesses need to monitor these trends to adjust their strategies and expectations accordingly.

Prerequisites

Understanding consumption and saving plans, along with the concept of marginal propensity, is crucial in economics. While there are no specific prerequisite topics listed for this subject, it's important to recognize that a strong foundation in basic economic principles is essential. These fundamental concepts provide the groundwork for comprehending more complex economic theories and models.

To fully grasp the intricacies of consumption and saving plans, as well as marginal propensity, students should have a solid understanding of microeconomics and macroeconomics. These fields provide the context for analyzing individual and aggregate economic behavior, which is at the core of consumption and saving decisions.

Additionally, familiarity with basic mathematical concepts, such as algebra and graphing, is beneficial. These skills are often used to illustrate economic relationships and trends, particularly when examining the marginal propensity to consume or save.

Another valuable prerequisite is an understanding of consumer behavior and decision-making processes. This knowledge helps in analyzing how individuals allocate their income between consumption and savings, which is central to the topic at hand.

Furthermore, a grasp of income and wealth distribution concepts can provide valuable insights into how different economic groups make consumption and saving decisions. This understanding is crucial when exploring the variations in marginal propensities across different income levels.

While not explicitly listed as prerequisites, concepts such as opportunity cost, supply and demand, and economic equilibrium are fundamental to understanding the broader economic context in which consumption and saving plans operate. These principles help explain the trade-offs individuals face when deciding how to allocate their resources.

Lastly, an awareness of economic indicators and their interpretation can be highly beneficial. This knowledge allows students to connect theoretical concepts of consumption, savings, and marginal propensity to real-world economic data and trends.

By building a strong foundation in these areas, students will be better equipped to delve into the specifics of consumption and saving plans, and to understand the nuances of marginal propensity. This comprehensive approach ensures a more thorough and meaningful grasp of the subject matter, enabling students to apply these concepts to both academic studies and real-world economic analysis.