Sign In
Sign Up
flag-uk

Short Run Product Curves: Unlocking Production Efficiency
Dive into the world of short run product curves and boost your microeconomics knowledge. Understand total, marginal, and average product curves to analyze production efficiency and make informed business decisions.

  1. Intros0/4 watched
  2. Examples0/14 watched
  1. 0/4
  2. 0/14
Now Playing:Short run product curve – Example 0a
Intros
0/4 watched
  1. Short Run Product Curve Overview:
  2. Short Run Product Curve Overview:
    Short-Run Constraint
    • A short time period
    • Capital is fixed
    • Only labour, raw materials, etc. can be changed
    • Short-run decisions can easily change
    • Increasing output in the short-run
  3. Short Run Product Curve Overview:
    3 Product Curves
    • Total Product Curve
    • Marginal Product Curve
    • Average Product Curve
Examples
0/14 watched
  1. Deriving Marginal & Average Product Curve
    Consider the following information:

    Labor (workers per week)

    Output (candies per week)

    0

    0

    1

    15

    2

    35

    3

    60

    4

    80

    5

    95

    6

    105

    1. Draw the total product curve

    2. Calculate and draw the marginal product curve

    3. Calculate and draw the average product curve

Short run product curve
Jump to:NotesConceptFAQsPrerequisites
Notes

Short-Run Constraint

Short run: is a time period in which the quantity of one or more resources used for production is fixed.


In the short run:

  1. Capital (tools, computers, buildings) is fixed
  2. Resources like labour can be changed
  3. Decisions can be easily changed

In this section, we will look at how the changes in labour affect the output in production.



3 Product Curves

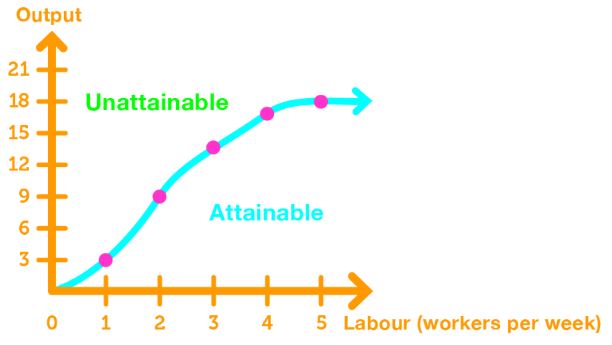
Total Product: the maximum output a given quantity of labor can produce.

Total product curve

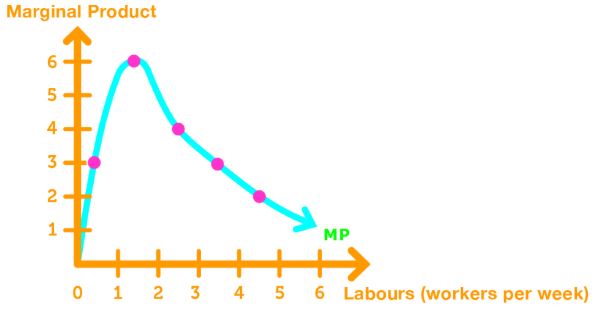
Marginal Product: The additional output gained from increasing one-unit of labor.

Marginal product curve

Average Product: The total product divided by the quantity of labor.

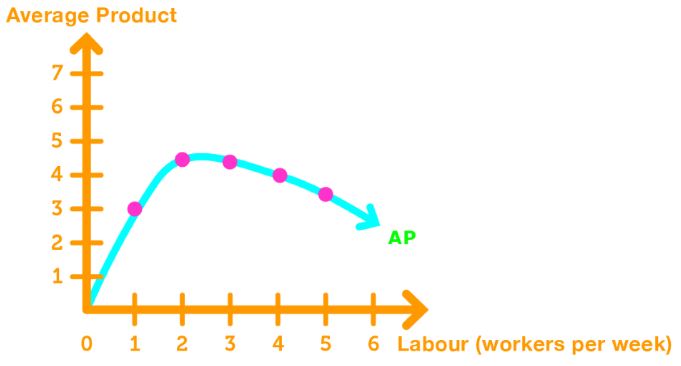
Average product curve

Law of Diminishing Returns

Notice from the marginal and average product curve that the law of diminishing returns applies.

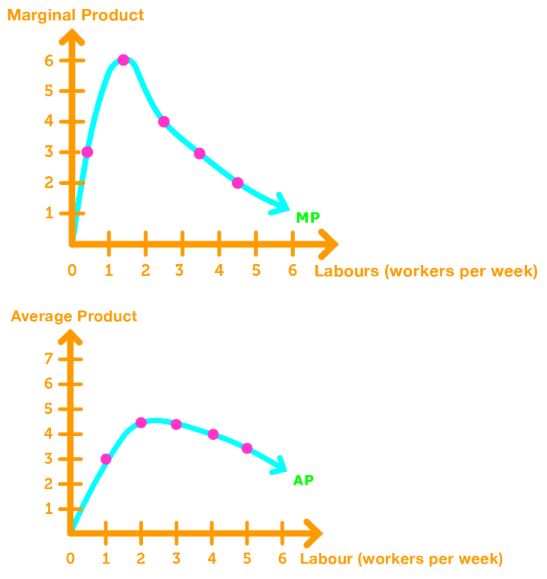
Law of diminishing returns

Notice the 2 features:

  1. Both curves increase due to specialization and division of labour.
  2. Both curves decrease later due to less access to capital, and less space to work.

In other words,

Hire more workers  \, →  \, Less productivity of workers \, →  \, Less gain in output


Maximizing Average Product

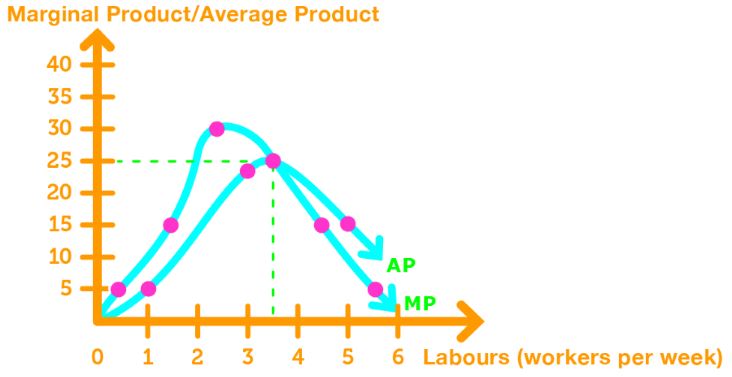
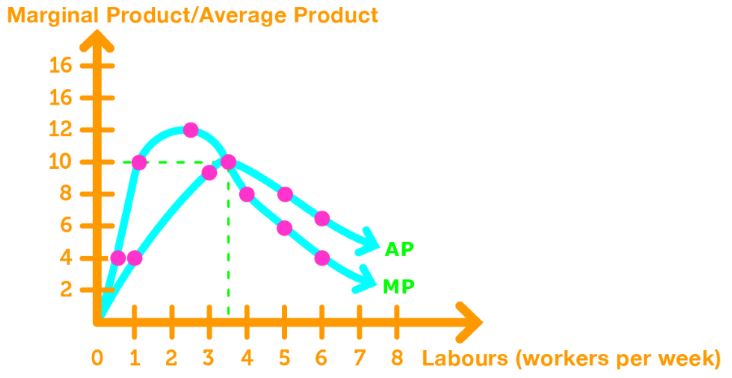
How can we maximize average product? Let’s look at the marginal & product curve in one graph.

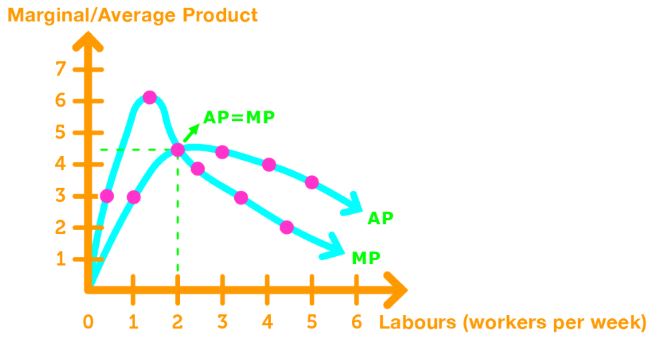
Marginal & product curve

  1. When MP > AP, the additional one-unit increase gives more output than the average output gained.
  2. When MP < AP, the additional one-unit increase gives less output than the average output gained.
  3. When MP = AP, the additional one-unit increase gives the same output as the average output gained.

Therefore, average product is maximized when MP = AP.

Concept

Introduction to Short Run Product Curves

Short run product curves are essential tools in microeconomics that help us understand how production changes as we vary a single input, typically labor, while keeping other factors constant. Our introduction video provides a visual and engaging overview of these curves, making it easier to grasp their significance in economic analysis. This article delves into three key components of short run production: total product, marginal product, and average product curves. Each of these curves offers unique insights into the production process and efficiency. The total product curve shows the overall output as we increase the variable input. The marginal product curve illustrates the additional output gained from each extra unit of input. Lastly, the average product curve represents the output per unit of input. By exploring these curves, we can better understand the relationships between inputs and outputs in the short run, providing valuable insights for business decision-making and economic policy analysis.

FAQs

Here are some frequently asked questions about short run product curves:

1. What is the explanation of the total product curve?

The total product curve shows the relationship between the quantity of a variable input (usually labor) and the total output produced, keeping other inputs fixed. It typically starts at the origin, rises at an increasing rate, then at a decreasing rate, and eventually flattens or declines, reflecting the law of diminishing returns.

2. What does the product curve explain?

Product curves explain the relationship between inputs and outputs in production. They illustrate how output changes as more of a variable input is added, while other inputs remain constant. This includes the total product curve (showing total output), marginal product curve (showing additional output from each extra unit of input), and average product curve (showing output per unit of input).

3. Why is the total product curve S-shaped?

The total product curve is S-shaped due to the changing returns to the variable input. Initially, there are increasing returns as specialization and efficiency improve (steep rise). Then, diminishing returns set in as fixed inputs become constraints (curve flattens). Finally, negative returns may occur if too much of the variable input is added (potential decline).

4. How do you find the average product curve?

To find the average product curve, divide the total product by the number of units of the variable input at each level. Plot these values on a graph with the variable input on the x-axis and the average product on the y-axis. The resulting curve typically rises, reaches a peak, and then declines, reflecting changes in production efficiency.

5. What is the difference between the average product curve and the marginal product curve?

The average product curve shows the output per unit of input, while the marginal product curve shows the additional output from one more unit of input. The marginal product curve intersects the average product curve at its peak. When marginal product is above average product, the average is rising; when it's below, the average is falling.

Prerequisites

Understanding the short run product curve is crucial in economics, but to fully grasp its concepts, it's essential to have a solid foundation in certain prerequisite topics. Two key areas that significantly contribute to comprehending the short run product curve are areas between curves and perfect competition in the short run.

The concept of areas between curves is fundamental when analyzing the relationship between marginal and average product curves, which are integral components of the short run product curve. By understanding how to calculate and interpret these areas, students can better visualize and quantify the changes in productivity over different levels of input. This mathematical foundation enables a more profound comprehension of the economic principles at play in short run production.

Moreover, grasping the intricacies of perfect competition in the short run provides crucial context for the short run product curve. This prerequisite topic elucidates the short run constraints in economics, which directly influence how firms make production decisions. By familiarizing themselves with the characteristics of perfect competition, students can more easily understand why and how the short run product curve behaves as it does under various market conditions.

The areas between curves concept helps in visualizing the relationship between total, average, and marginal product curves. This mathematical tool is invaluable when analyzing the stages of production and identifying key points such as the point of diminishing returns. By mastering this prerequisite, students can more effectively interpret the shape and implications of the short run product curve.

Similarly, knowledge of perfect competition in the short run provides the economic framework within which the short run product curve operates. Understanding how firms behave in a perfectly competitive market in the short run helps explain why they continue to produce even when facing losses, and how this relates to the shape of the short run product curve.

By thoroughly studying these prerequisite topics, students build a strong foundation for understanding the short run product curve. The mathematical skills gained from analyzing areas between curves combined with the economic principles of perfect competition in the short run create a comprehensive toolkit for interpreting and applying the short run product curve concept in various economic scenarios.

In conclusion, mastering these prerequisite topics is not just about accumulating knowledge; it's about developing a holistic understanding of how different economic and mathematical concepts interrelate. This interconnected knowledge allows for a deeper appreciation of the short run product curve and its significance in economic analysis and decision-making.