Given the following diagram:
Specify the direction as a:
Draw a suitable diagram for each of the following bearings:
Convert
3 main types of bearings:
- Standard compass bearings
- Compass bearings
- True bearings
Cardinal Points: the 4 basic standard bearings (East, South, West, North)
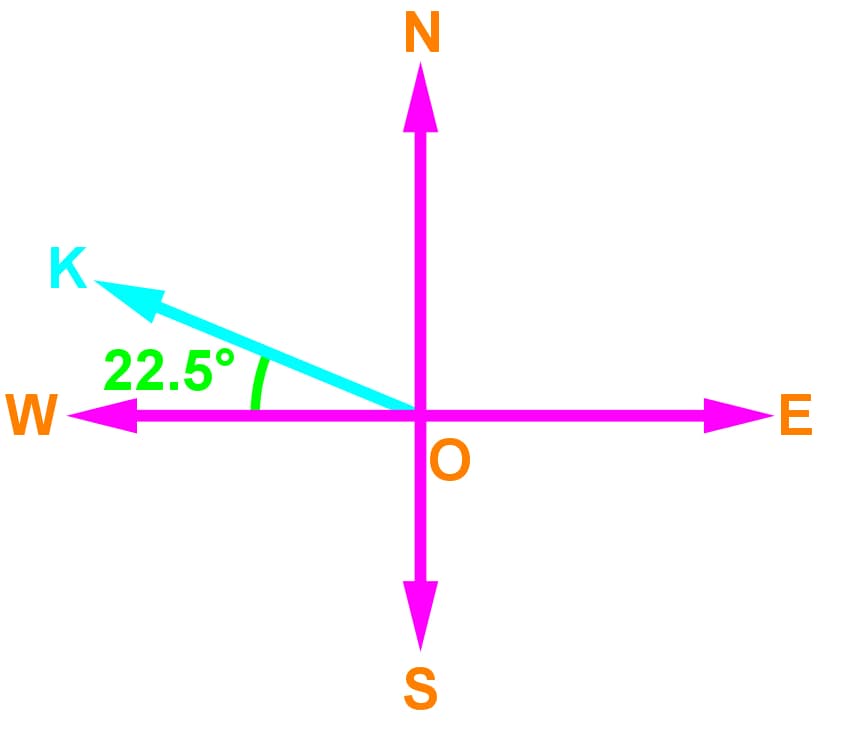
Compass bearings: the number of degrees east or west of north of south.
True bearings:
- Angle measured clockwise to the bearing line from the north
- The symbol T is to indicate that it is true bearing, but sometimes it is omitted
- For bearings less than 100°, use 3 digits with the first digit being a 0. (e.g. 045°T)
Bearings in mathematics are a crucial concept used to describe directions and angles relative to a fixed point, typically north. This system is essential in navigation, surveying, and various real-world applications. In daily life, bearings help us understand maps, give directions, and navigate using compasses or GPS devices. The bearing meaning in math extends beyond simple compass directions, incorporating precise angular measurements. Understanding bearings is vital for pilots, sailors, hikers, and anyone involved in outdoor activities or transportation. To grasp this concept fully, an introduction video can be incredibly beneficial. Such a video typically demonstrates how to measure and calculate bearings, explains their relationship to angles and compass points, and provides practical examples. By watching an introductory video, learners can visualize the concept, making it easier to comprehend and apply in both mathematical problems and real-world scenarios. This foundation is crucial for further studies in geometry, trigonometry, and navigation.
Introduction to bearings Standard compass bearings
In this section, we want to give you an introduction to bearings because it's just so useful in our daily lives. It doesn't matter if you're in the ocean or on land. If you're lost, being able to do bearings might actually save your life. That's why we want to talk about this topic. So, let's begin with this lesson.
To start off, we need to know the definition of bearings. Bearings are used to locate objects or the direction of a journey on a 2D plane. That means you want to be able to pinpoint yourself on a map using bearings.
Now, to do so, there are actually three ways. Three types of bearings. The first type is the standard compass bearing. And here's the example. We don't worry about this. It might be a bit overwhelming, but we will go over it in details in just a moment. Second one is compass bearings. And last one is the true bearings. So, let's go over these one by one and give you an example and explain how to find the corresponding bearings.
First one, obviously, is the standard compass bearings. The word standard here has a meaning. You know, when you pull a compass, how many arrows do you see? You see four arrows in general, right? Those are the standard directions. So, these are the four standard directions you see on the compass. And they are the E, which is the east, obviously. S is south. West is W. And the N is north. These are the four most general standard compass bearings you see on a compass. So, these four directions are called the cardinal points, okay? This is a specific name for these four directions.
Now, here comes a question. Even though these are the most general form, what's going to happen if we have a direction in between these directions? For example, if I have a direction pointing in between the north and the east, right in the middle, okay? What do we do? Over here, whenever we're solving for degrees, we always start counting on the north, right? From the north, that is the zero degrees. And we want to go clockwise, right? We go clockwise, like that. So, if north is zero degrees, then east, in that case, will be 90 degrees, because that is the right angle, right? All right, midway from zero to 90, okay? That midpoint over here, what is the degree of that? Well, we just have to divide 90 by 2. 90 divided by 2, it's 45. That means this angle over here, it's 45 degrees.
Okay, so let me rephrase the question. This midpoint over here would just be 45 degrees, but how do we express it using these alphabets? The north is N, east is E, so on and so forth. Well, what we can do is that we can combine these two directions into one. So, we use the north or the south as reference, and we take into account the east and west to find the angle. So, for example, this 45 degrees, we will use the north because it's closer to the north than the south, right? So, use north as a reference, and then from north, you go towards east, right? So, we will have northeast. So, this angle, according to this diagram, 45 degrees right here, it's denoted by northeast. It's called northeast, okay?
So, that's what you do if you were to find an angle in between these four cardinal points, okay? So, using the same logic, we'll be able to find the southeast, southwest, and northwest, right? These extra four points on top of the four cardinal points. Now, one step forward, one step ahead. What happens if I have an arrow in between the north and the northeast, okay? Right in the middle. So, you see, from northeast, that's 45 degrees, okay? So, if I have an arrow in between them, so that means we are doing 45 degrees divided by 2. 45 divided by 2, that will give us 22.5 degrees. So, this angle over here, it's actually 22.5 degrees.
So, we want to denote 22.5 degrees using these standard compass bearings, all right? So, again, what we can do is that we can use either north or south as our reference, and then we point towards the northeast or northwest. So, for example, for NE, northeast, start from the north, okay? If it's closer to the north than the south, it wouldn't make sense if you say south, northeast, right? It's way too far from there. So, you will say north, northeast. So, NNE, so that will be NNE for 22.5. It's NNE, north, northeast, okay? So, that is denoted on this diagram over here, all right?
However, the difference to find these in-between angles from here is that we don't just use the north and the south. We will use the east and the west as reference angles as well, okay? So, for example, if I want to find an angle right in between over here, it wouldn't make sense to use north or south, right? Because they are still way too far from this angle. So, the closest reference angle in this case, sorry, reference bearing will be the east, all right? East is just right next to it, right? So, what we will do is that we will say east, northeast. Because east, you point, switch it a bit towards the northeast. So, it's ENE, all right? ENE over here, all right?
So, using the same idea, we will be able to find all these in-between standard bearings. These ones, all right? And all in all, these will be the 16 standard compass bearings you can find, all right? 16 of them in total. So, let me make a quick conclusion. Starting off, we will have four basic standard compass bearings which have a special name. They're called the cardinal points. To find the in-between numbers, you will first use the north or south as the mid reference angles. To find the in-betweens, all right? So, to get the northeast, southeast, southwest, and northwest. However, if you want to further find the in-between points, you would not only use north and south, but also east and west to find in-between points. So, you get 16 standard compass bearings in all, all right?
Here are some frequently asked questions about bearings in mathematics:
To calculate bearings, follow these steps:
The three main rules for bearings are:
To find the bearing from A to B:
True bearing is measured from true north, not magnetic north. To find it:
An example of a bearing is 045°, which means 45 degrees clockwise from north (northeast). Another example is 270°, which represents due west. Bearings are always measured clockwise from north and expressed as three-digit numbers between 000° and 359°.
Understanding the fundamentals is crucial when delving into the world of "Introduction to bearings." While there are no specific prerequisite topics listed for this subject, it's important to recognize that a solid foundation in basic mechanical engineering concepts can greatly enhance your comprehension of bearings and their applications.
Bearings are essential components in various mechanical systems, and having a grasp of general physics principles, such as friction, force, and motion, can provide valuable context. These fundamental concepts form the backbone of understanding how bearings function and why they are so important in machinery and equipment.
Additionally, familiarity with materials science can be beneficial when studying bearings. Different types of bearings are made from various materials, each with its own properties and applications. Understanding the characteristics of metals, plastics, and ceramics used in bearing construction can help you appreciate the design choices and performance capabilities of different bearing types.
Basic knowledge of mechanical design principles can also prove useful when learning about bearings. This includes understanding concepts like load distribution, stress analysis, and rotational dynamics. These principles play a crucial role in bearing selection, installation, and maintenance.
While not strictly prerequisites, having some background in manufacturing processes and quality control can provide valuable insights into how bearings are produced and tested. This knowledge can help you appreciate the precision and care that goes into creating reliable bearings for various applications.
Lastly, a general understanding of lubrication principles can be advantageous when studying bearings. Many bearings rely on proper lubrication to function effectively and efficiently. Knowing the basics of lubricant types, properties, and application methods can enhance your comprehension of bearing maintenance and longevity.
Although there are no specific prerequisite topics listed for "Introduction to bearings," approaching the subject with a well-rounded understanding of these related areas can significantly enhance your learning experience. It's important to remember that learning is a continuous process, and you can always revisit and strengthen your knowledge of these foundational concepts as you progress in your study of bearings.
As you embark on your journey to understand bearings, keep in mind that curiosity and a willingness to explore related fields can greatly enrich your learning experience. Don't hesitate to dive into supplementary materials or seek out additional resources to build a strong foundation for your study of bearings and their fascinating role in the world of mechanical engineering.
