Circle Graphs: Mastering Data Interpretation and Creation Unlock the power of circle graphs! Learn to interpret, create, and analyze these essential data visualization tools. Perfect for students and professionals seeking to enhance their analytical skills.
Intros
0/2 watched
Examples
0/10 watched
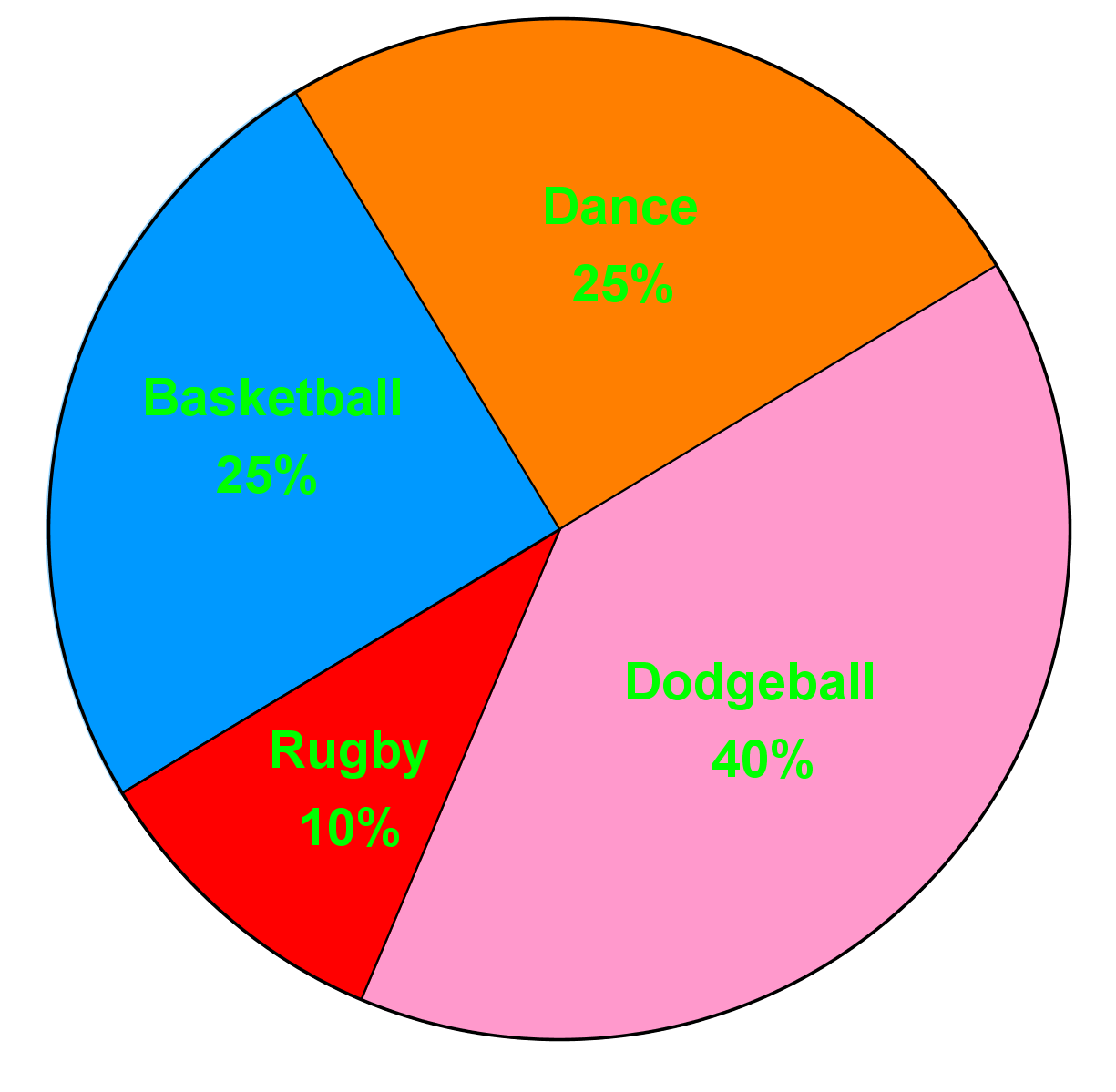
- The circle graph shows the types of activities that 200 students participated in during the school year.
- Compare the data in these two circle graphs.
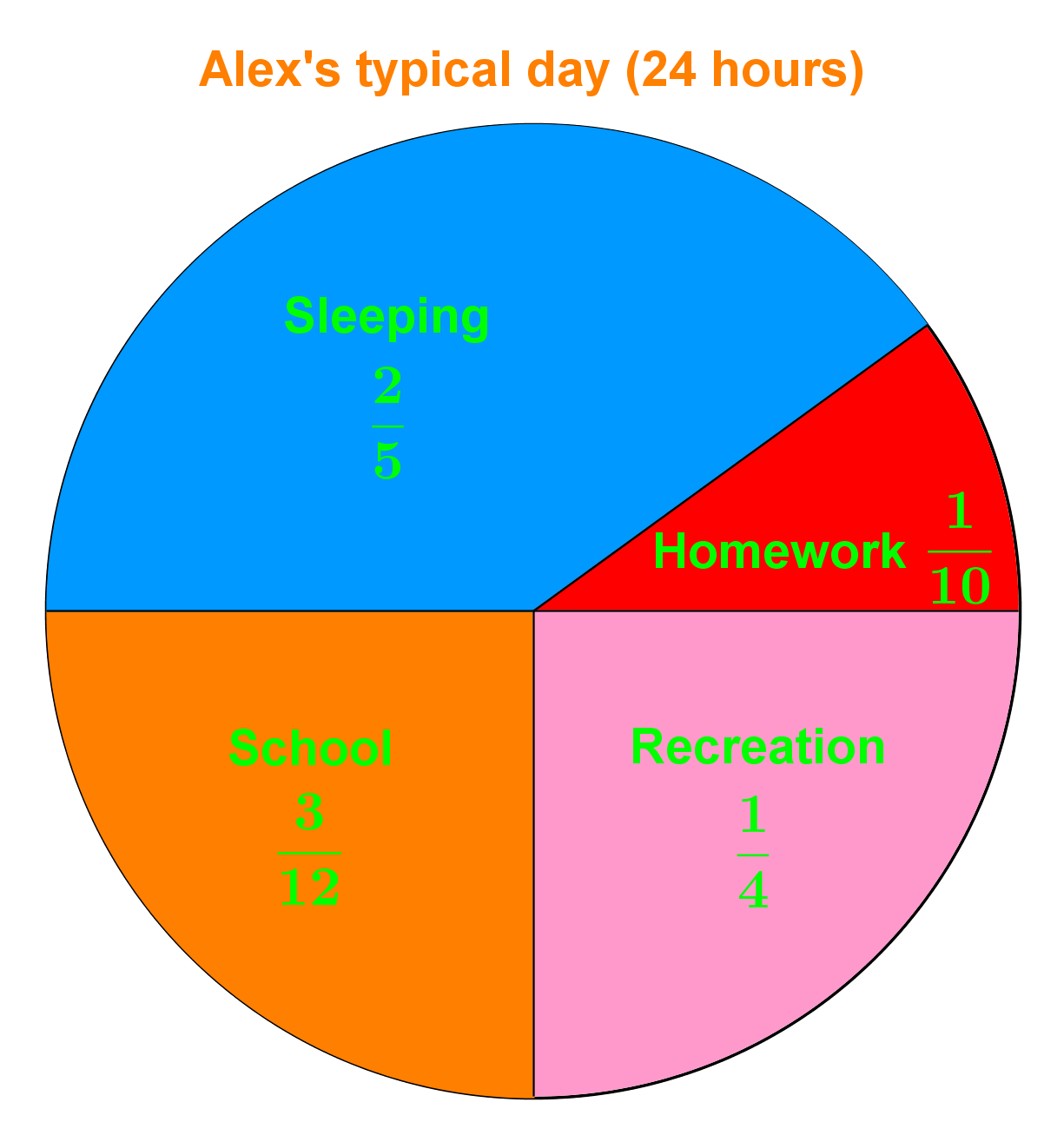
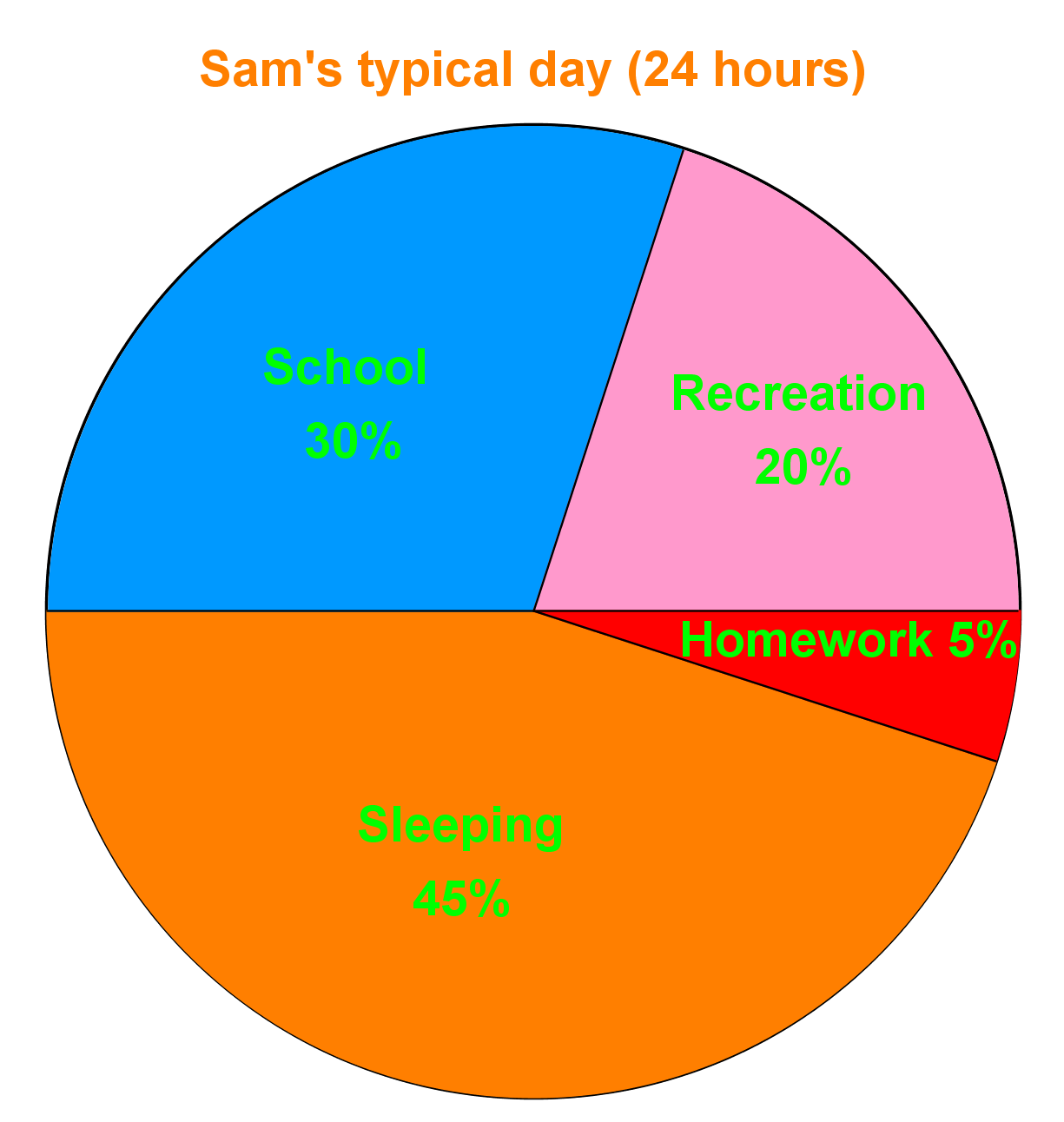
- Who spends more time sleeping in a typical day?
- How much longer does Alex spend on recreation activities than Sam in a typical day?
- Which activity do both Alex and Sam spend most of their time on?
- What is the difference between the measures of the central angles in the Recreation section of Alex and Sam's day?
- 30 grade 7 students were surveyed to determine their favorite type of pop.
- Could you use a circle graph to represent the following? Explain.






