Sign In
Sign Up
flag-sg

Gradient equation: m=y2−y1x2−x1m = \frac{y_2-y_1}{x_2- x_1}

0/1
Intros
Start Watching
Lessons
  1. Overview: Slopes of lines
    Overview: Slopes of lines
0/10
Examples
Start Watching
Lessons
  1. Determine the slope using the "rise over run" method
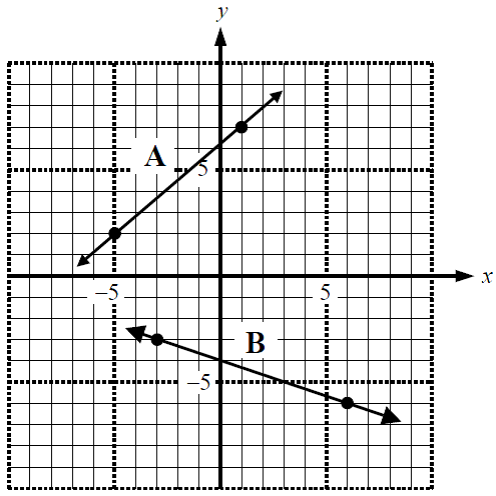
    Slope formula: m = y_2-y_1/x_2- x_1
    1. Line A
    2. Line B
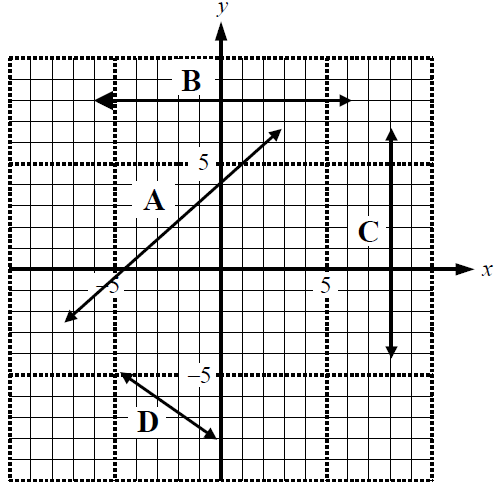
  2. Determine the slope based on the graph: positive, negative, zero, or undefined, and verify
    Slope formula: m = (y_2-y_1)/(x_2- x_1)
    1. Line A
    2. Line B
    3. Line C
    4. Line D
  3. Given two points of a line, determining the slope using m=(y2−y1)/(x2−x1)m = (y_2 - y_1) / (x_2 - x_1)
    1. (−2,7)(-2,7) and (6,−6)(6, -6)
    2. (53,67)(\frac{5}{3}, \frac{6}{7}) and (35,−83)(\frac{3}{5} , \frac{-8}{3})
  4. Arrange the slopes from flattest to steepest.
    1. −3,12,34,32-3,\frac{1}{2},\frac{3}{4},\frac{3}{2}
  5. Two isosceles triangles have the same height. The slopes of the sides of triangle A are double the slopes of the corresponding sides of triangle B. How do the lengths of their bases compare?