Vibration and energy

In this lesson, we will learn:

• Vibrating and oscillating systems
• Hooke’s law
• How to find the amount of energy stored in a vibrating system?

Notes:

• There are many examples of vibrating objects around us, an object attached to the end of spring, guitar strings, ruler held firmly at the end of the table.
• During vibration (oscillation) the object moves back and forth about a fixed position called “equilibrium position”.
• Let’s consider a mass vibrating at the end of a uniform spring.;

$\qquad \quad$(a) The mass is at its equilibrium position, there is no force exerted.



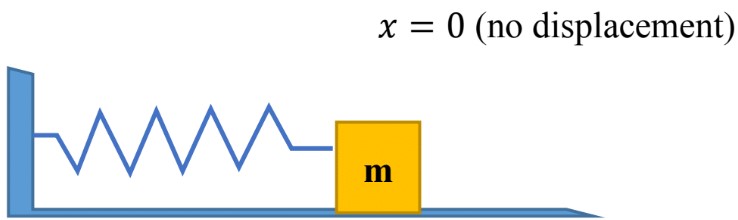
$\qquad \quad$(b) The mass is oscillating; the restoring force tries to pull the mass back to its equilibrium position.


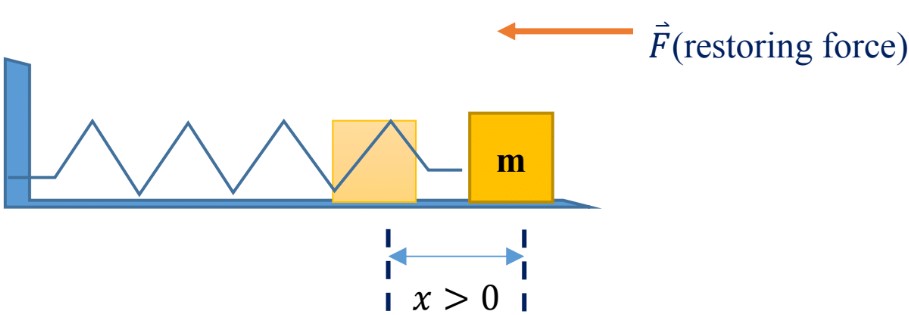
$\qquad \quad$(c) The mass is oscillating; the restoring force tries to push the mass back to its equilibrium position.


Hooke’s Law

• The magnitude of the restoring force is directly proportional to the displacement $x$;
  $F \propto x$
• The direction of the restoring force is always opposite to the displacement which is indicated by a minus sign in the equation.
  
  $F = - kx$ (Hooke’s Law)

$F$: Force exerted by the spring on the mass
$K$: Spring constant
$x$: Displacement

Energy

• As we know the mechanical energy of a system is the sum of kinetic and potential energies.
• In the case of the spring-mass system, the potential energy would be in the form of elastic potential energy in the spring which is calculated using the following equation;

$PE = \frac{1}{2}kx^{2}$

Therefore; the mechanical energy of the system is;

$E = \frac{1}{2}mv^{2} \, + \, \frac{1}{2}kx^{2}$
$\qquad \quad$(a) At the extreme points where the mass stops momentarily to change the direction; $v$ = 0, $x$ = $A$ (amplitude, maximum displacement)

$E = \frac{1}{2}kA^{2} \,$ (1)
$\qquad \quad$(b) At the equilibrium position the mass moves with maximum velocity;

$V = V_{max},x = 0$

$E = \frac{1}{2} mv^{2} _{max}$ (2)

From (1) and (2);

$\frac{1}{2}kA^{2} = \frac{1} {2}mv^{2} _{max} \quad \Rightarrow \quad V^{2} _{max} = (\frac{k}{m}) \, A^{2}$


$\qquad \quad$(c) At intermediate points, the energy is a part kinetic and part potential;

$\frac{1}{2}mv^{2} \, + \, \frac{1}{2}kx^{2} = \frac{1}{2}kA^{2}$

$\qquad$
• From the above equation we can find velocity as the function of position;

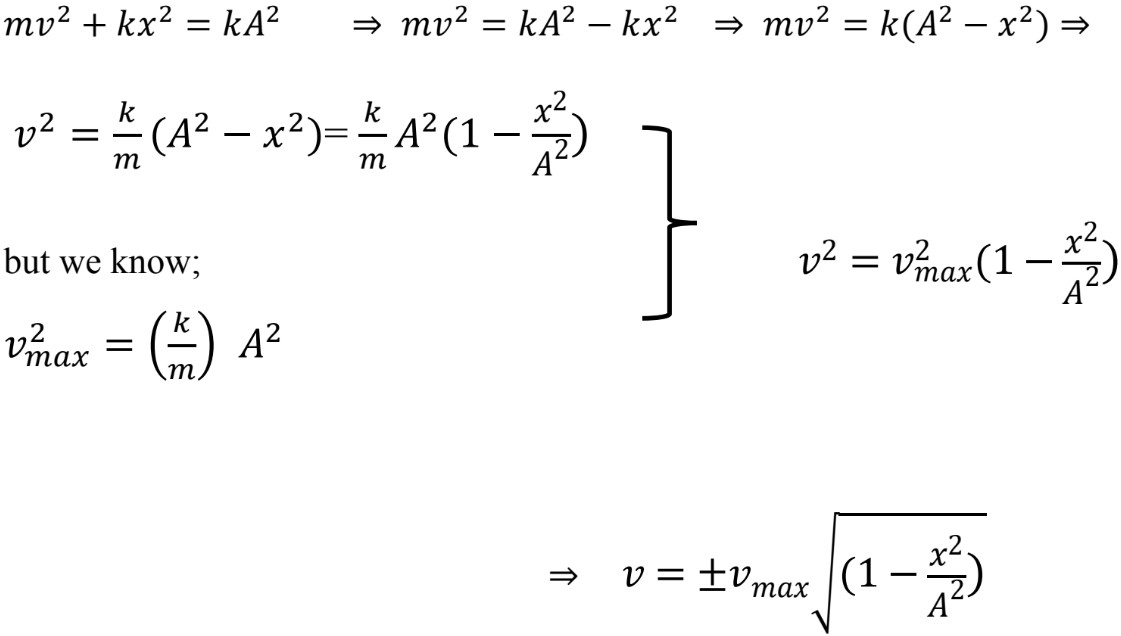
This gives the velocity of the object at any position.