Mechanical waves 

Everything You Need in One Place

Homework problems? Exam preparation? Trying to grasp a concept or just brushing up the basics? Our extensive help & practice library have got you covered.

Learn and Practice With Ease

Our proven video lessons ease you through problems quickly, and you get tonnes of friendly practice on questions that trip students up on tests and finals.

Instant and Unlimited Help

Our personalized learning platform enables you to instantly find the exact walkthrough to your specific type of question. Activate unlimited help now!

?
Intros
Lessons
  1. Mechanical Waves
  2. Introduction to periodic waves
  3. Velocity
?
Examples
Lessons
  1. A musical note of frequency 1.8 Hz is sounded on a day when the speed of sound in air is 320 m/s. What is the wavelength of this note in air?
    1. A train of waves travel through a rope at the rate of four waves per second. Find the period of the motion.
      1. The time taken for one complete cycle of a musical note is 2 × 10-4 s. Find the frequency of the sound.
        1. Radio waves are broadcasted at a frequency of120 MHz. Find the period of the electromagnetic waves producing thus type of Radio waves.
          1. A person shouts towards a vertical cliff 630m away. The echo of his voice is heard 6.00 s later.
            1. What is the speed of sound of the person's voice?
            2. The wavelength of the sound is 0.650m. What is its frequency?
            3. What is the period of the wave?
            1. In order to produce larger wavelength, do you need higher or lower frequency?
              1. Five pulses are generated every 0.200s in a pond. What is the speed of propagation of the wave if the wavelength of the surface wave in 2.40cm?
                1. A periodic longitudinal wave that has a frequency on 40.0 Hz travels along a coil spring. If the distance between two successive compressions in 0.600m, what is the speed of the water? 
                  Topic Notes
                  ?

                  In this lesson, we will learn:

                  • Wave properties
                  • The relation between wave speed, wave-length, and the frequency.
                  • Solving exercises relating variables.

                  Notes:

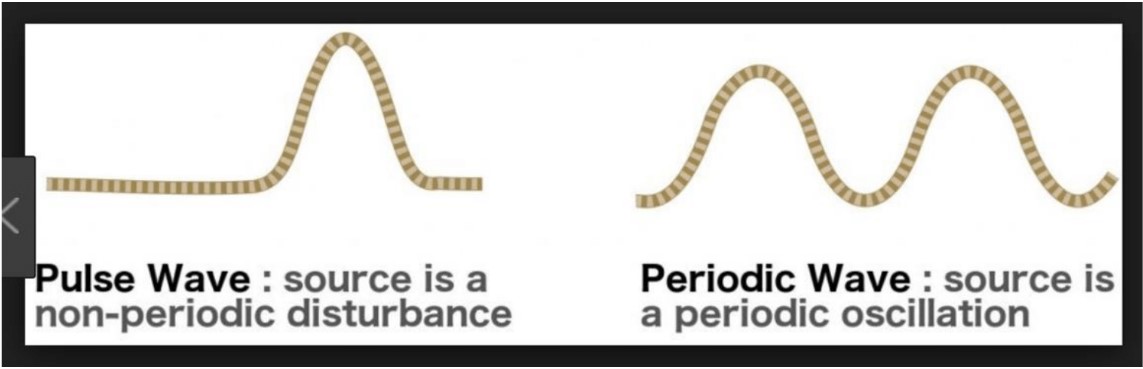
                  Periodic Waves
                  • Pulse: Single disturbance of a medium
                  • Periodic Waves: Continual disturbance of a medium.
                    Particles in the medium move in a simple harmonic motion.

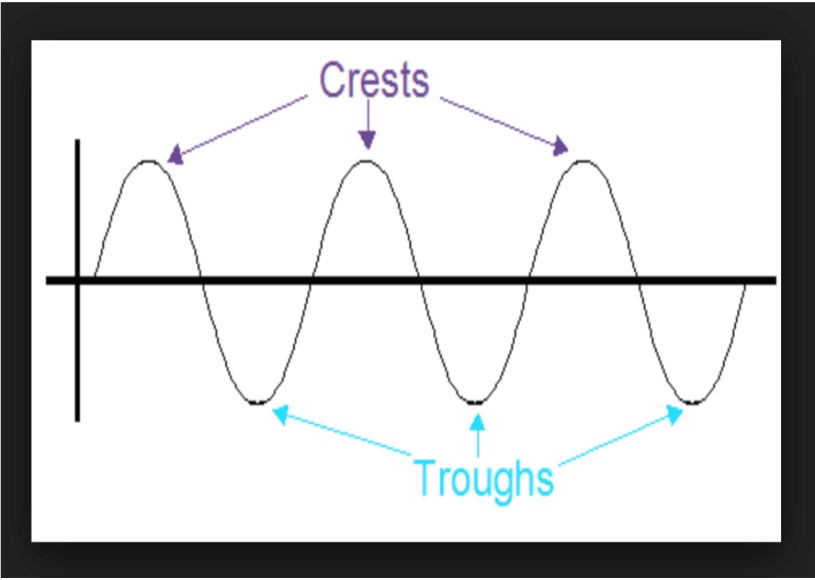
                  Mechanical Waves


                    Crest: The top of the wave is called the crest; maximum displacement.

                    (Note: in the case of longitudinal waves we use the term Compression, representing maximum pressure or maximum density)

                    Trough: The bottom part of the wave is called the trough; minimum displacement.

                    (Note: In the case of longitudinal waves the term Rarefaction is used, minimum pressure or minimum density)

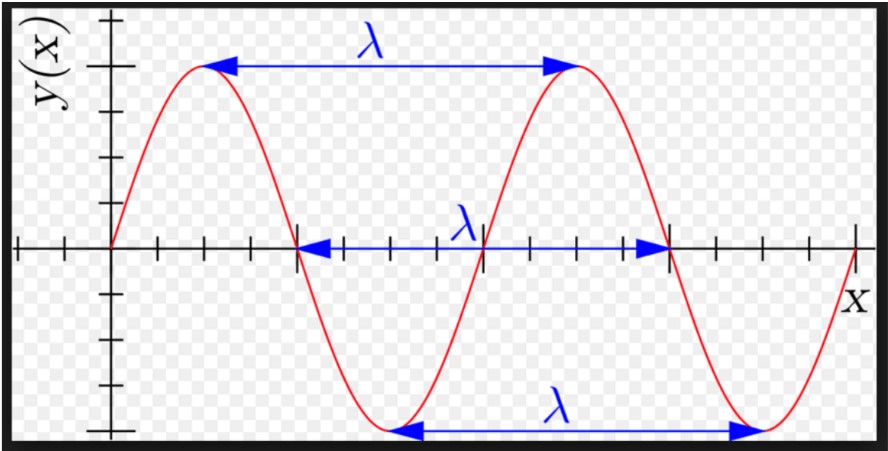
                  Mechanical Waves

                    Wavelength: The distance between two successive crests (troughs) called wavelength

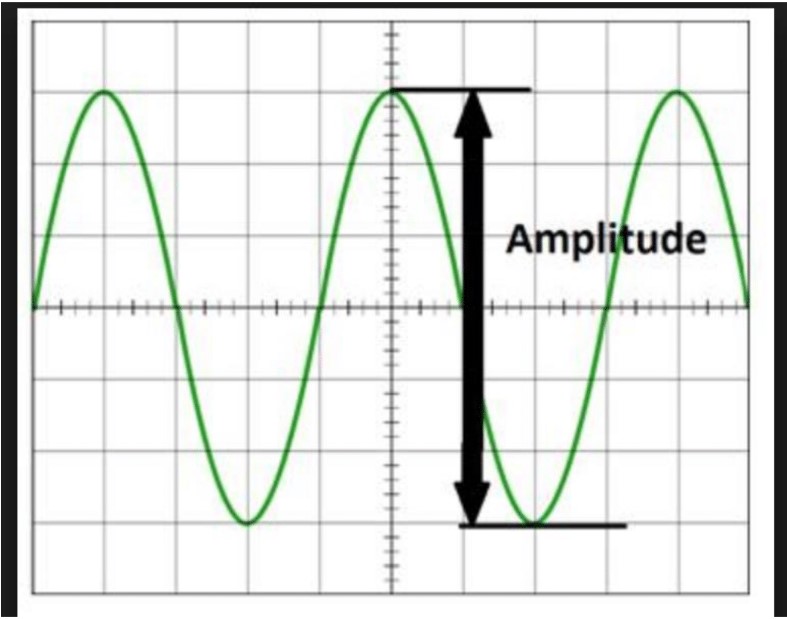
                  Mechanical Waves

                    Amplitude: The height of a crest or depth of a trough called amplitude.

                    A high energy wave is characterized by a high?amplitude

                    A low energy wave is characterized by a low?amplitude

                  Mechanical Waves

                    Period: The time between two consecutive crests (troughs) called the period. The period is the time taken for one wavelength to pass by a point.

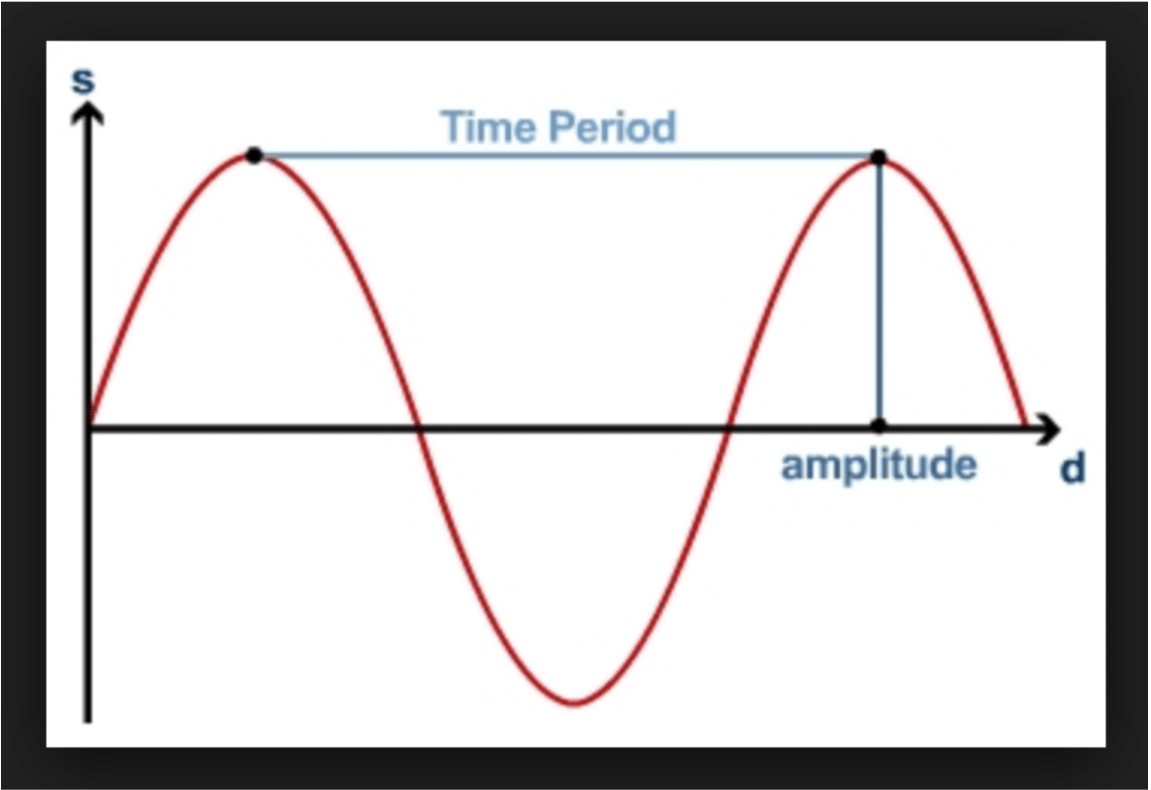
                  Mechanical Waves

                    Frequency: Number of waves passing through a fixed point in ONE second.


                    Table of terms and units of periodic motion

                    \quadVariable\quad

                    \quadSymbol \quad

                    \quadStandard Units \quad

                    Wavelength

                    λ \lambda (Lambda)

                    Meters (m)

                    Amplitude

                    A

                    Meters (m)

                    Period

                    T

                    Seconds(s)

                    Frequency

                    f

                    Hz (hertz) =waves/seconds

                    =1/seconds = (s-1 )

                    Velocity: How fast the pulse is moving though a medium; the speed of the wave depends on the medium thought which it travels.

                    Using the kinematic equation for constant velocity, the equation for speed (velocity) of the wave is given by:

                    △X=v.t ⇒v=△Xt \triangle X = v.t \, \Rightarrow \enspace v = \frac{\triangle X} {t}

                    Change in position (distance between two consecutive crests) of a wave is defines as its WAVELENGTH, therefore; 

                    △X=λ \triangle X = \lambda

                    Time taken between two consecutive crests is defined as the PERIOD of the motion, therefore; t=T t=T

                    v=△Xt=λT(1) v=λT v = \frac{\triangle X} {t} = \frac{\lambda}{T} \qquad (1) \, v = \frac{\lambda}{T}

                    Frequency is defined as number of waves per second, therefore; FREQUENCY is the reciprocal of the period,

                    (2) f=1T (2) \, f = \frac{1}{T}


                    From (1) & (2);

                    (3) v=λT=λ(1T)=λf  (3) \, v = \frac{\lambda}{T} = \lambda (\frac{1}{T}) = \lambda f \,