Magnetic Fields of Long Wires & Forces Between Them

Magnets and magnetic fields

Jump to:Notes Concept FAQs Prerequisites

Notes

In this lesson, we will learn:

Magnetic field due to a long straight wire
Magnetic field midway between two currents
Forces between two parallel wires

Notes:

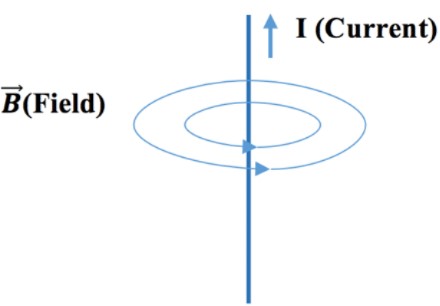
An electric current produces a magnetic field

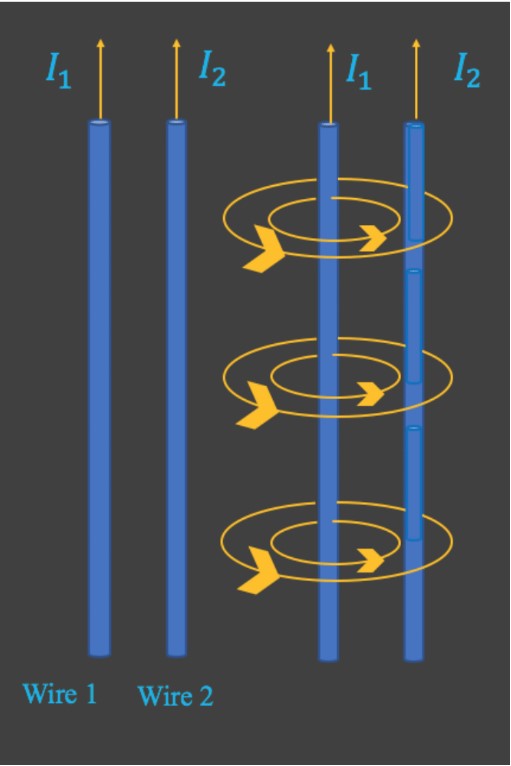
The magnetic field surrounding the electric current in a long straight wire is such that the field lines are circles with the wire at the center.
The field strength at a given point would be greater if the current flowing in the wire were greater; $B \propto I$
The filed strength would be less at points farther from the wire $B \propto \frac{1}{r}$ ;

\quad B \propto I

\quad B \propto \frac{1}{r} \qquad B \propto \frac{1}{r} \quad \Rightarrow \quad B =

\large \frac{\mu_{0}}{2 \pi} \frac{I}{r}

The value of the constant

\mu_{0}

, which is called the permeability of free space, is

\mu_{0}

= 4

\pi

× 10^-7

T.m/A

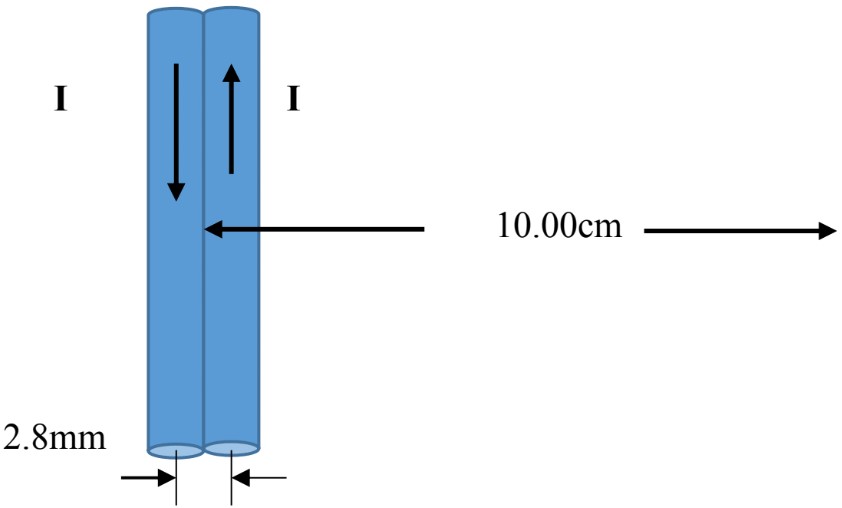
Magnetic field due to a long straight wire & force between two parallel wires

Magnetic Field Midway Between Two Currents
Two parallel wires 10.0cm apart carry currents in opposite directions. Current

I_{1}

= 5.0A is out of the page,

I_{2}

=7.0 A is into the page. Determine the magnitude and direction of the magnetic field halfway between the two wires.

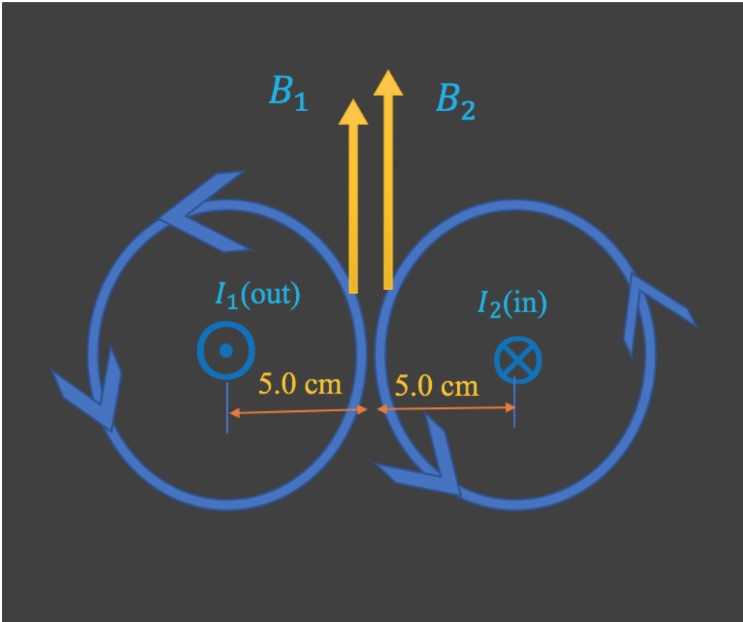
Magnetic field due to a long straight wire & force between two parallel wires

B_{1} =

\large \frac{\mu_{0} I_{1}} {2\pi r} = \frac{(4\pi \, \times \, 10^{-7} \, T\, . \, m/A) (5. 0A) } {2 \pi (0.050m) }

= 2.0 × 10^-5

T

B_{2} =

\large \frac{\mu_{0} I_{2}} {2\pi r} = \frac{(4\pi \, \times \, 10^{-7} \, T\, . \, m/A) (7. 0A) } {2 \pi (0.050m) }

= 2.8 × 10^-5

T

The total filed is up with the magnitude of

B = B_{1} +B_{2} = 4.8 \times 10^{-5} T

Forces Between Two Parallel Wires

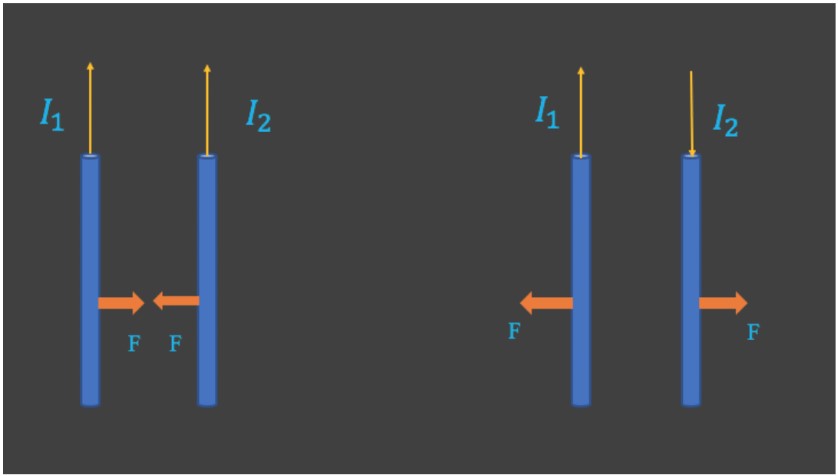
Consider two long parallel wires separated by a distance $d$ . They carry currents $I_{1}$ and $I_{2}$ , respectively. Each current produces a magnetic field that is felt by the other, so each must exert a force on the other.

Magnetic field $B_{1}$ produced by $I_{1}, \, B_{1} =$ $\large \frac{\mu_{0} I_{1}} {2 \pi d}$
The force $F_{2}$ is exerted by $B_{1}$ on a length of $I_{2}$ of wire 2, carrying current $I_{2}$ , $F_{2}$ = $I_{2}$ $B_{1}$ $I_{2}$
Substitute $B_{1}$ into $F_{2}$ formula to get the final equation;

F_{2} =

\large \frac{\mu_{0}} {2 \pi} \frac{I_{1} I_{2}} {d} I_{2}

Parallel currents in the same direction exert an attractive force on each other
Antiparallel currents (in opposite directions) exert a repulsive force on each other.

Concept

Introduction

The magnetic field due to a long straight wire and the force between two parallel wires are fundamental concepts in electromagnetism. Understanding these principles is crucial for grasping the behavior of electric currents and their interactions. The magnetic field around a long straight wire forms concentric circles, with its strength decreasing as distance from the wire increases. This field follows the right-hand rule, where the thumb points in the direction of current flow, and the fingers curl around the wire in the field's direction. When two parallel wires carry currents, they exert forces on each other due to their magnetic fields. These forces can be attractive or repulsive, depending on the current directions. The visual demonstration of magnetic fields provides a visual demonstration of these concepts, making it easier to comprehend the invisible magnetic fields and their effects. By exploring these electromagnetic phenomena, students can develop a solid foundation for more advanced topics in physics and electrical engineering.

FAQs

Here are some frequently asked questions about magnetic fields due to a long straight wire and force between two parallel wires:

What is the right-hand rule for determining the direction of a magnetic field around a wire?
The right-hand rule states that if you wrap your right hand around the wire with your thumb pointing in the direction of the current, your fingers will curl in the direction of the magnetic field lines. This rule helps visualize the circular nature of the magnetic field around a current-carrying wire.
How does the strength of a magnetic field change with distance from a long straight wire?
The strength of the magnetic field decreases inversely with the distance from the wire. This relationship is described by the equation B = μI / (2πr), where B is the magnetic field strength, μ is the permeability of free space, I is the current, and r is the distance from the wire.
What determines whether parallel current-carrying wires attract or repel each other?
The direction of the currents in the wires determines whether they attract or repel. If the currents flow in the same direction, the wires attract each other. If the currents flow in opposite directions, the wires repel each other. This is due to the interaction of their magnetic fields.
How is the force between two parallel current-carrying wires calculated?
The force per unit length between two parallel current-carrying wires is given by the equation F/L = (μII) / (2πd), where F is the force, L is the length of the wires, μ is the permeability of free space, I and I are the currents in each wire, and d is the distance between the wires.
What are some practical applications of the magnetic fields and forces between current-carrying wires?
Practical applications include electric motors, generators, transformers, electromagnetic rail guns, maglev trains, and particle accelerators. These concepts are also fundamental in defining the ampere (the SI unit of electric current) and in various precision measurement instruments used in scientific research and industry.

Prerequisites

Understanding the fundamental concepts that lay the groundwork for more advanced topics in physics is crucial for students aiming to master complex subjects like "Magnetic field due to a long straight wire & force between two parallel wires." One of the most essential prerequisite topics for this subject is electric currents produce magnetic fields. This foundational concept is pivotal in comprehending the intricate relationship between electricity and magnetism, which forms the basis of electromagnetism.

The principle that electric currents generate magnetic fields is a cornerstone in electromagnetic theory. When studying the magnetic field due to a long straight wire, students must first grasp how moving charges (electric current) create a magnetic field around the conductor. This understanding is crucial because it explains the origin of the magnetic field that surrounds a current-carrying wire, which is the primary focus of the main topic.

Moreover, the concept of vector addition of magnetic fields is particularly relevant when analyzing the force between two parallel wires. As each wire produces its own magnetic field, the interaction between these fields results in a force that can be attractive or repulsive, depending on the direction of the currents. Students who have a solid understanding of how to add magnetic field vectors will find it much easier to visualize and calculate these interactions.

The prerequisite topic also introduces students to the right-hand rule, a fundamental tool used to determine the direction of magnetic fields around current-carrying conductors. This rule is indispensable when working with long straight wires and parallel conductors, as it helps predict the direction of the magnetic field and the resulting forces between wires.

Furthermore, understanding how electric currents produce magnetic fields lays the groundwork for more advanced concepts such as Ampère's law and Biot-Savart law, which are often used to calculate the strength and direction of magnetic fields around various current-carrying configurations, including long straight wires.

By mastering this prerequisite topic, students will be better equipped to tackle the complexities of magnetic fields due to long straight wires and the forces between parallel conductors. They will have the necessary tools to visualize the magnetic field lines, understand their behavior, and calculate their effects on neighboring current-carrying wires. This foundational knowledge not only facilitates a deeper understanding of the main topic but also prepares students for more advanced studies in electromagnetism and its numerous applications in modern technology and engineering.

Magnetic Fields and Forces: Long Wires Demystified
Uncover the mysteries of magnetic fields around long wires and the forces between parallel conductors. Visualize complex concepts and build a strong foundation in electromagnetism for advanced physics studies.

Free to Join!

Easily See Your Progress

Make Use of Our Learning Aids

Last Viewed

Practice Accuracy

Suggested Tasks

Earn Achievements as You Learn

Create and Customize Your Avatar

Introduction

Magnetic Fields and Forces: Long Wires Demystified Uncover the mysteries of magnetic fields around long wires and the forces between parallel conductors. Visualize complex concepts and build a strong foundation in electromagnetism for advanced physics studies.

Easily See Your Progress

Make Use of Our Learning Aids

Last Viewed

Practice Accuracy

Suggested Tasks

Earn Achievements as You Learn

Create and Customize Your Avatar

Introduction

Become a member to get more!

Magnetic Fields and Forces: Long Wires Demystified
Uncover the mysteries of magnetic fields around long wires and the forces between parallel conductors. Visualize complex concepts and build a strong foundation in electromagnetism for advanced physics studies.