Faraday’s law
Intros
Examples
Lessons
- Four conductors of different lengths are moved through a uniform magnetic field at the same speed. Which conductor will induce the greatest emf?
- A part of a coil wire is placed in a uniform magnetic field as shown. Which two directions of motion would immediately induce an emf in the coil?
- 1 and 2
- 1 and 3
- 2 and 3
- 2 and 4
- The diagram below shows an aluminum ring and the current induced in it by the nearby magnet that is free to move along its central axis.
The magnet must be:- stationary
- moving to the left
- moving to the right
- spinning about its central axis.
- The diagram below shows two coils in a magnetic field.
An electric current can be induced in the coil oriented with its plane.- parallel to a constant magnetic field.
- parallel to a changing magnetic field.
- perpendicular to a constant magnetic field.
- perpendicular to a changing magnetic field.
- A metal block moves with a constant speed in a uniform magnetic field.
Which side of the block is positive?- JK
- KL
- LM
- MJ
Topic Notes
Introduction to Faraday's Law
Welcome to our exploration of Faraday's law, a fundamental principle in electromagnetism that revolutionized our understanding of electricity and magnetism. This law, discovered by Michael Faraday in 1831, explains the phenomenon of electromagnetic induction. It describes how a changing magnetic field can induce an electric current in a nearby conductor. To kick off our learning journey, I've prepared an introduction video that visually demonstrates this concept. This video is crucial as it breaks down the complex ideas into easily digestible parts, making Faraday's law more accessible. You'll see real-life applications and experiments that showcase the law in action. As we progress, we'll delve deeper into the mathematical formulations and practical implications of Faraday's law. Remember, this principle underpins much of our modern technology, from electric generators to transformers. So, let's get started and unravel the mysteries of electromagnetic induction together!
Faraday's Law of Induction
Introduction to Faraday's Law
Faraday's Law of Induction is a fundamental principle in electromagnetism that describes how a changing magnetic field can induce an electromotive force (EMF) in a conductor. This law forms the basis for many modern technologies, including electric generators and transformers.
The Concept of Magnetic Flux
To understand Faraday's Law, we must first grasp the concept of magnetic flux. Magnetic flux is a measure of the total magnetic field passing through a given area. It is denoted by the Greek letter Φ (phi) and is measured in Weber (Wb). The equation for magnetic flux is:
Φ = B * A * cos(θ)
Where:
- B is the magnetic field strength (in Tesla)
- A is the area of the loop
- θ (theta) is the angle between the magnetic field lines and the normal to the loop's surface
B Perpendicular and Its Significance
The concept of B perpendicular is crucial in understanding magnetic flux. It refers to the component of the magnetic field that is perpendicular to the surface area. When the magnetic field lines are perpendicular to the surface (θ = 0°), cos(θ) = 1, resulting in maximum flux. Conversely, when the field lines are parallel to the surface (θ = 90°), cos(θ) = 0, resulting in zero flux.
Faraday's Law and Induced EMF
Faraday's Law states that the induced EMF in a closed loop is equal to the negative rate of change of magnetic flux through the loop. Mathematically, it is expressed as:
ε = -dΦ/dt
Where:
- ε (epsilon) is the induced EMF
- dΦ/dt is the rate of change of magnetic flux
Factors Affecting Induced EMF
Several factors influence the magnitude of the induced EMF:
- Rate of Change of Magnetic Flux: A faster change in magnetic flux results in a larger induced EMF. This can be achieved by rapidly moving a magnet near a coil or quickly changing the current in a nearby conductor.
- Loop's Area: Increasing the area of the loop exposed to the changing magnetic field will increase the induced EMF. This is why transformers often use coils with many turns to maximize the effective area.
- Angle: The angle between the magnetic field lines and the normal to the loop's surface affects the induced EMF. Maximum EMF is induced when the field lines are perpendicular to the surface.
- Number of Turns: In a coil with multiple turns, the induced EMF is multiplied by the number of turns, as each turn experiences the changing flux.
Examples of Faraday's Law in Action
Example 1: Moving Loop
Imagine a rectangular loop of wire moving through a uniform magnetic field. As the loop enters or exits the field, the magnetic flux through it changes, inducing an EMF. The direction of the induced current can be determined using Lenz's Law, which states that the induced current will flow in a direction that opposes the change in magnetic flux.
Example 2: Transformer
In a transformer, the primary coil is connected to an AC source, creating a changing magnetic field. This changing field induces an EMF in the secondary coil. The ratio of the number of turns in each coil determines the voltage transformation.
Applications
Magnetic Field and Loop Orientation
The relationship between magnetic field lines and loop orientation is a fundamental concept in electromagnetism, playing a crucial role in understanding magnetic flux and its applications. This relationship is essential for various electromagnetic devices and phenomena, from simple electric generators to complex power generation systems.
Magnetic field lines are imaginary lines that represent the direction and strength of a magnetic field in space. They are typically depicted as continuous curves that emerge from the north pole of a magnet and enter the south pole. The density of these lines indicates the strength of the magnetic field, with closer lines representing a stronger field.
When we consider a loop of wire in a magnetic field, its orientation relative to the field lines significantly affects the magnetic flux through the loop. Magnetic flux is a measure of the total magnetic field passing through a given area. The orientation of the loop determines how much of the magnetic field passes through it, which in turn influences the induced electromagnetic effects.
Let's examine two extreme scenarios: when the loop is parallel and when it's perpendicular to the magnetic field lines.
Parallel Orientation
When the loop is oriented parallel to the magnetic field lines, the plane of the loop is perpendicular to the field lines. In this configuration, the maximum number of field lines pass through the loop's area. This orientation results in the maximum possible magnetic flux through the loop.
Mathematically, the magnetic flux (Φ) is given by the equation: Φ = BA cos θ, where B is the magnetic field strength, A is the area of the loop, and θ is the angle between the magnetic field lines and the normal to the loop's plane. In the parallel orientation, θ = 0°, and cos 0° = 1, so the flux is at its maximum value: Φ = BA.
Perpendicular Orientation
Conversely, when the loop is oriented perpendicular to the magnetic field lines, the plane of the loop is parallel to the field lines. In this configuration, no magnetic field lines pass through the loop's area. This orientation results in zero magnetic flux through the loop.
Using the same equation, Φ = BA cos θ, in the perpendicular orientation, θ = 90°, and cos 90° = 0, so the flux becomes zero: Φ = 0.
Intermediate Orientations
For any orientation between parallel and perpendicular, the magnetic flux will be somewhere between the maximum value and zero. The exact value depends on the angle θ between the field lines and the normal to the loop's plane. As the loop rotates from a parallel to a perpendicular orientation, the flux decreases according to the cosine function in the equation.
Implications and Applications
Understanding the relationship between magnetic field lines and loop orientation is crucial in many practical applications. For instance, in electric generators, the orientation of the loop (or coil) is deliberately changed to induce an alternating current. By rotating a loop in a magnetic field, the flux through the loop continuously changes, inducing an electromotive force (EMF) and generating electricity.
In transformers, the orientation of the primary and secondary coils relative to the magnetic field in the core is designed to maximize flux linkage and energy transfer. Similarly, in electric motors, the orientation of the rotor coils relative to the stator's magnetic field is carefully controlled to produce rotational motion.
The concept also extends to more advanced applications in physics and engineering. For example, in magnetic resonance imaging (MRI), the orientation of hydrogen atoms in the body relative to the applied magnetic field is crucial for generating detailed images. In particle accelerators, the orientation of magnetic fields is precisely controlled to guide charged particles along desired paths.
In conclusion, the relationship between magnetic field lines and loop orientation is a fundamental principle in electromagnetism. It determines the magnetic flux through a loop, which is essential for understanding and designing a wide range of electromagnetic devices and systems. By manipulating this relationship, we can harness the power of magnetism for numerous practical applications in technology and science.
Faraday's Law Equation and Applications
Faraday's Law of Electromagnetic Induction is a fundamental principle in electromagnetism, describing how a changing magnetic field induces an electromotive force (EMF) in a conductor. The complete Faraday's Law equation is expressed as:
ε = -N(dΦ/dt)
This induced EMF equation encapsulates the essence of electromagnetic induction. Let's break down each component:
- ε (epsilon) represents the induced electromotive force (EMF) in volts.
- N is the number of turns or loops in the conductor.
- dΦ/dt denotes the rate of change of magnetic flux over time.
- The negative sign (-) indicates the direction of the induced current, following Lenz's Law.
The significance of the negative sign in Faraday's Law is crucial. It represents Lenz's Law, which states that the induced current flows in a direction that opposes the change in magnetic flux that caused it. This opposition is nature's way of conserving energy and maintaining equilibrium.
The change in flux (dΦ/dt) is a key component of the equation. It represents how quickly the magnetic field lines are cutting through the conductor. Factors affecting this change include the strength of the magnetic field, the area of the loop, and the angle between the field and the loop.
When dealing with multiple loops, Faraday's Law applies cumulatively. The total induced EMF is the sum of the EMFs induced in each individual loop. This is why the number of turns (N) is multiplied by the rate of change of magnetic flux in the equation. More loops result in a stronger induced EMF, which is why transformers and electric generators often use coils with many turns.
Faraday's Law finds numerous applications in real-world scenarios:
- Electric Generators: These devices convert mechanical energy into electrical energy by rotating a coil in a magnetic field, inducing an EMF.
- Transformers: Used to step up or step down voltage in power distribution systems, transformers rely on Faraday's Law to transfer energy between coils.
- Induction Cooktops: These modern cooking appliances use rapidly changing magnetic fields to induce currents directly in the cookware, generating heat.
- Electric Guitar Pickups: The vibration of metal strings in a magnetic field induces a current in the pickup coils, converting mechanical vibrations into electrical signals.
- Electromagnetic Flow Meters: Used to measure the flow rate of conductive liquids in pipes by inducing a voltage proportional to the flow velocity.
- Wireless Charging: This technology uses electromagnetic induction to transfer energy between two coils, charging devices without direct electrical contact.
Understanding Faraday's Law is crucial for engineers and physicists working on various electromagnetic applications. It explains how we can generate electricity on a large scale, transform voltages for efficient power transmission, and create innovative devices that harness the power of electromagnetic induction.
In conclusion, Faraday's Law, represented by the equation ε = -N(dΦ/dt), is a cornerstone of electromagnetism. It quantifies how changing magnetic fields induce electromotive forces, taking into account the number of loops and the rate of flux change. The law's applications span from large-scale power generation to everyday consumer electronics, demonstrating its fundamental importance in modern technology and energy systems.
Methods of Changing Induced EMF
Induced electromotive force (EMF) is a fundamental concept in electromagnetism, and understanding the methods of changing it is crucial for various applications in physics and engineering. There are three primary methods of altering induced EMF: changing the magnetic field strength, modifying the loop's area, and adjusting the angle between the magnetic field and the loop. Each of these methods has unique implications for the induced current and magnetic field.
1. Changing the Magnetic Field Strength: The first method involves altering the strength of the magnetic field. According to Faraday's law of induction, the induced EMF is directly proportional to the rate of change of magnetic flux. By increasing or decreasing the magnetic field strength, we can significantly impact the induced EMF. For example, in a simple generator, rotating a magnet faster near a coil of wire will increase the rate of change of the magnetic field, resulting in a higher induced EMF. This principle is widely used in power generation, where the rotation speed of turbines affects the output voltage.
2. Changing the Loop's Area: The second method focuses on modifying the area of the loop or coil exposed to the magnetic field. The induced EMF is proportional to the area of the loop perpendicular to the magnetic field lines. By increasing the loop's area, we can capture more magnetic flux, leading to a higher induced EMF. Conversely, decreasing the area will reduce the induced EMF. This principle is applied in transformers, where the number of turns in the primary and secondary coils affects the voltage transformation. It's also relevant in the design of electric motors, where the size and shape of the armature coils influence the motor's efficiency.
3. Changing the Angle: The third method involves adjusting the angle between the magnetic field lines and the plane of the loop. The induced EMF is maximum when the magnetic field lines are perpendicular to the loop's plane and minimum (zero) when they are parallel. This relationship is described by the cosine of the angle between the field lines and the normal to the loop's plane. In practical applications, such as in AC generators, this principle is utilized by rotating a coil in a fixed magnetic field, causing a continuous change in the angle and thus producing an alternating current.
The implications of these changes on the induced current and magnetic field are significant. The induced current, according to Lenz's law, always flows in a direction that opposes the change in magnetic flux that caused it. This means that as we increase the induced EMF through any of the three methods, the induced current will also increase, creating its own magnetic field that opposes the original field. This opposition is the basis for electromagnetic braking systems and eddy current damping in various devices.
In the case of changing magnetic field strength, a rapidly changing field will induce stronger currents, which in turn create stronger opposing magnetic fields. This principle is used in induction heating, where rapidly alternating magnetic fields induce currents in conductive materials, causing them to heat up. When changing the loop's area, larger areas allow for greater induced currents, which can be beneficial in power generation but may also lead to increased energy losses in transformers if not properly managed.
The angle change method is particularly important in AC power generation and motors. As the angle between the magnetic field and the loop changes, the induced EMF varies sinusoidally, resulting in alternating current. This forms the basis of our AC power systems and the operation of synchronous motors. The continuous change in angle ensures a constant flow of induced current, which is essential for sustained power generation or motor operation.
Understanding and manipulating these three methods of changing induced EMF is crucial in the design and optimization of various electromagnetic devices. Engineers and physicists use these principles to develop more efficient generators, motors, transformers, and other electromagnetic equipment. By carefully controlling the magnetic field strength, loop area, and angle, they can fine-tune the performance of these devices to meet specific requirements in power generation, electric vehicles, and countless other applications.
In conclusion, the ability to change induced EMF through these three methods provides a powerful toolkit for controlling electromagnetic phenomena. Whether it's generating electricity, powering motors, or designing advanced sensors, the principles of changing magnetic field strength, loop area, and angle are at the heart of modern electromagnetic technology. As we continue to advance in fields like renewable energy and electric transportation, these fundamental concepts will remain crucial in driving innovation and efficiency in electromagnetic
Practical Applications and Experiments
Faraday's Law of Electromagnetic Induction is not just a theoretical concept; it's a principle that powers many of the technologies we use every day. Let's explore some exciting practical applications and fun experiments that bring this law to life!
One of the most common applications of Faraday's Law is in generators. These devices convert mechanical energy into electrical energy, powering our homes, businesses, and cities. When you flip a light switch, you're benefiting from Faraday's discovery! Generators work by rotating a coil of wire within a magnetic field, inducing an electric current. This principle is used in everything from massive power plants to portable generators for camping trips.
Transformers are another crucial application of Faraday's Law. These devices are essential for our power distribution systems, allowing electricity to be transmitted over long distances efficiently. Transformers use electromagnetic induction to step up or step down voltage levels, ensuring that the electricity reaching our homes is at a safe and usable level. Next time you see those large boxes on utility poles, remember that Faraday's Law is at work!
A more recent and innovative application is the induction cooktop. These sleek, modern cooking surfaces use electromagnetic induction to heat pots and pans directly, without heating the cooktop itself. When you place a magnetic cookware on an induction cooktop, it creates a magnetic field that induces electric currents in the pot, heating it rapidly and efficiently. It's a safer and more energy-efficient cooking method, all thanks to Faraday's Law!
Now, let's get hands-on with some simple experiments you can try at home or in the classroom to demonstrate Faraday's Law:
- Magnet and Coil Experiment: Wind a coil of wire around a cardboard tube and connect the ends to a sensitive galvanometer or multimeter. Move a strong magnet in and out of the tube quickly. You'll see the meter deflect, indicating an induced current!
- Homemade Generator: Attach several strong magnets to a rotating shaft (like a hand-crank or small motor). Place a coil of wire near the rotating magnets and connect it to an LED. As you turn the shaft, the changing magnetic field will induce a current, lighting up the LED!
- Electromagnetic Swing: Create a pendulum with a strong magnet attached to the end. Set up two coils of wire on either side of the pendulum's path. Connect each coil to an LED. As the magnet swings through the coils, it will induce a current, causing the LEDs to blink alternately!
These experiments are not only fun but also provide a tangible understanding of how changing magnetic fields can induce electric currents. They demonstrate the core principle behind generators, transformers, and induction cooktops. By exploring these practical applications and conducting hands-on experiments, you're following in the footsteps of Michael Faraday himself, who was a master of both theoretical understanding and practical demonstration.
So, the next time you use an electric appliance, ride in an electric vehicle, or cook on an induction stove, take a moment to appreciate the brilliant simplicity of Faraday's Law. It's a testament to how fundamental scientific principles can lead to world-changing technologies. Keep experimenting, stay curious, and who knows? You might just invent the next big application of electromagnetic induction!
Conclusion
Faraday's Law is a fundamental principle in electromagnetic induction, stating that a changing magnetic field induces an electromotive force in a conductor. This law is crucial for understanding the generation of electricity in power plants and the operation of transformers. The introduction video provides a clear visualization of this concept, making it easier to grasp the relationship between magnetic flux and induced current. The importance of Faraday's Law extends beyond theoretical physics, playing a vital role in modern technology and energy production. To deepen your understanding, we encourage you to explore further resources and conduct simple experiments at home. Try creating a basic generator or investigate the effects of changing magnetic fields on different materials. By engaging with these hands-on activities, you'll gain a more profound appreciation for the practical applications of Faraday's Law in our daily lives. Don't hesitate to seek out additional educational materials or join online forums to discuss your findings with fellow enthusiasts.
Faraday's Law
Faraday's Law of Induction is a fundamental principle in electromagnetism that describes how a change in magnetic flux can induce an electromotive force (EMF) in a loop of wire. This law is crucial for understanding how electric generators, transformers, and many other electrical devices operate.
Step 1: Understanding Faraday's Law
Faraday's Law states that the induced EMF in a loop is proportional to the rate of change of magnetic flux through the loop. The magnetic flux (ΦB) is defined as the product of the magnetic field (B), the area of the loop (A), and the cosine of the angle (θ) between the magnetic field and the normal to the loop's surface. Mathematically, it is expressed as:
ΦB = B × A = B × A × cos(θ)
Where B is the component of the magnetic field perpendicular to the loop's surface. The unit of magnetic flux is the Weber (Wb), which is equivalent to Tesla meter squared (T·m²).
Step 2: Factors Affecting Induced EMF
The induced EMF depends on several factors:
- The rate of change of magnetic flux (dΦB/dt).
- The area of the loop (A).
- The angle (θ) between the magnetic field and the normal to the loop's surface.
According to Faraday's Law, the induced EMF (ε) is given by:
ε = -dΦB/dt
The negative sign indicates the direction of the induced EMF, as described by Lenz's Law, which states that the induced EMF will always oppose the change in magnetic flux that caused it.
Step 3: Magnetic Flux Components
To understand the components of magnetic flux, consider a magnetic field (B) with two components: B (perpendicular to the loop) and B (parallel to the loop). In Faraday's Law, only the perpendicular component (B) contributes to the magnetic flux through the loop.
For example, if the magnetic field is perpendicular to the loop, the angle θ is 0 degrees, and cos(0) = 1, resulting in maximum magnetic flux. Conversely, if the magnetic field is parallel to the loop, the angle θ is 90 degrees, and cos(90) = 0, resulting in zero magnetic flux.
Step 4: Calculating Magnetic Flux
To calculate the magnetic flux (ΦB), use the formula:
ΦB = B × A × cos(θ)
Consider two scenarios:
- If the loop is parallel to the magnetic field (θ = 90°), the magnetic flux is zero because cos(90°) = 0.
- If the loop is perpendicular to the magnetic field (θ = 0°), the magnetic flux is maximum because cos(0°) = 1.
Step 5: Practical Applications
Faraday's Law has numerous practical applications, including:
- Electric generators: Convert mechanical energy into electrical energy by rotating a coil within a magnetic field, inducing an EMF.
- Transformers: Transfer electrical energy between circuits through electromagnetic induction, changing voltage levels.
- Induction cooktops: Use changing magnetic fields to induce currents in cookware, generating heat.
Understanding Faraday's Law is essential for designing and analyzing these devices, ensuring efficient and effective operation.
Step 6: Summary
In summary, Faraday's Law of Induction describes how a changing magnetic flux induces an EMF in a loop. The induced EMF depends on the rate of change of magnetic flux, the area of the loop, and the angle between the magnetic field and the loop's surface. By understanding and applying Faraday's Law, we can design and optimize various electrical devices and systems.
FAQs
-
What is Faraday's Law of Electromagnetic Induction?
Faraday's Law states that the induced electromotive force (EMF) in a closed loop is equal to the negative rate of change of magnetic flux through the loop. It's expressed mathematically as ε = -dΦ/dt, where ε is the induced EMF and dΦ/dt is the rate of change of magnetic flux.
-
How does changing the loop's area affect induced EMF?
Changing the loop's area affects the amount of magnetic flux passing through it. Increasing the area exposed to the magnetic field will increase the induced EMF, as more magnetic field lines can pass through the larger area. This principle is utilized in the design of electric generators and transformers to maximize efficiency.
-
What are some practical applications of Faraday's Law?
Faraday's Law has numerous practical applications, including:
- Electric generators for power production
- Transformers in power distribution systems
- Induction cooktops for efficient cooking
- Electromagnetic flow meters for measuring conductive liquid flow
- Wireless charging technology for electronic devices
-
How does the angle between magnetic field lines and the loop affect induced EMF?
The angle between magnetic field lines and the loop's normal affects the magnetic flux. Maximum EMF is induced when the field lines are perpendicular to the loop's surface (θ = 0°, cos θ = 1). When the field lines are parallel to the surface (θ = 90°, cos θ = 0), no EMF is induced. This relationship is described by the equation Φ = BA cos θ, where Φ is magnetic flux, B is magnetic field strength, A is loop area, and θ is the angle.
-
What is the significance of the negative sign in Faraday's Law equation?
The negative sign in Faraday's Law equation (ε = -dΦ/dt) represents Lenz's Law. It indicates that the induced current flows in a direction that opposes the change in magnetic flux that caused it. This opposition is nature's way of conserving energy and maintaining equilibrium. Understanding this concept is crucial for predicting the behavior of induced currents in various electromagnetic applications.
Prerequisite Topics for Understanding Faraday's Law
Faraday's law is a fundamental principle in electromagnetism, but to truly grasp its significance, it's crucial to have a solid understanding of several prerequisite topics. These foundational concepts provide the necessary context and background knowledge to fully appreciate the intricacies of Faraday's law.
One of the key prerequisites is understanding the rate of change. This mathematical concept is essential when dealing with Faraday's law, as it directly relates to the rate of change of magnetic flux. In Faraday's law, we're concerned with how quickly the magnetic field is changing, which is why a strong grasp of rate of change is crucial. It allows us to quantify and analyze the variations in magnetic flux over time, a cornerstone of electromagnetic induction.
Another vital prerequisite topic is the magnetic field due to a long straight wire & force between two parallel wires. This concept provides the foundation for understanding how magnetic fields behave and interact. When studying Faraday's law, we often deal with loops of wire in magnetic fields. Knowing how the angle between the magnetic field and the loop affects the induced current is crucial. This prerequisite topic helps build that understanding, making it easier to visualize and calculate the effects described by Faraday's law.
Lastly, familiarity with electric generators & counter EMF (Back EMF) is incredibly beneficial when delving into Faraday's law. Electric generators are practical applications of Faraday's law, demonstrating how changing magnetic fields can induce electrical currents. Understanding the principles behind generators provides real-world context for Faraday's law and helps illustrate its importance in modern technology. Additionally, the concept of counter EMF, or back EMF, is directly related to Lenz's law, which is often studied alongside Faraday's law and helps explain the direction of induced currents.
By mastering these prerequisite topics, students can approach Faraday's law with a more comprehensive understanding. The rate of change concept provides the mathematical tools, the understanding of magnetic fields offers the physical framework, and knowledge of electric generators gives practical context. Together, these prerequisites create a solid foundation upon which a deeper understanding of Faraday's law can be built, enabling students to better grasp its implications and applications in the field of electromagnetism.
Magnetic Field and Loop Orientation
The relationship between magnetic field lines and loop orientation is a fundamental concept in electromagnetism, playing a crucial role in understanding magnetic flux and its applications. This relationship is essential for various electromagnetic devices and phenomena, from simple electric generators to complex power generation systems.
Magnetic field lines are imaginary lines that represent the direction and strength of a magnetic field in space. They are typically depicted as continuous curves that emerge from the north pole of a magnet and enter the south pole. The density of these lines indicates the strength of the magnetic field, with closer lines representing a stronger field.
When we consider a loop of wire in a magnetic field, its orientation relative to the field lines significantly affects the magnetic flux through the loop. Magnetic flux is a measure of the total magnetic field passing through a given area. The orientation of the loop determines how much of the magnetic field passes through it, which in turn influences the induced electromagnetic effects.
Let's examine two extreme scenarios: when the loop is parallel and when it's perpendicular to the magnetic field lines.
Parallel Orientation
When the loop is oriented parallel to the magnetic field lines, the plane of the loop is perpendicular to the field lines. In this configuration, the maximum number of field lines pass through the loop's area. This orientation results in the maximum possible magnetic flux through the loop.
Mathematically, the magnetic flux (Φ) is given by the equation: Φ = BA cos θ, where B is the magnetic field strength, A is the area of the loop, and θ is the angle between the magnetic field lines and the normal to the loop's plane. In the parallel orientation, θ = 0°, and cos 0° = 1, so the flux is at its maximum value: Φ = BA.
Perpendicular Orientation
Conversely, when the loop is oriented perpendicular to the magnetic field lines, the plane of the loop is parallel to the field lines. In this configuration, no magnetic field lines pass through the loop's area. This orientation results in zero magnetic flux through the loop.
Using the same equation, Φ = BA cos θ, in the perpendicular orientation, θ = 90°, and cos 90° = 0, so the flux becomes zero: Φ = 0.
Intermediate Orientations
For any orientation between parallel and perpendicular, the magnetic flux will be somewhere between the maximum value and zero. The exact value depends on the angle θ between the field lines and the normal to the loop's plane. As the loop rotates from a parallel to a perpendicular orientation, the flux decreases according to the cosine function in the equation.
Implications and Applications
Understanding the relationship between magnetic field lines and loop orientation is crucial in many practical applications. For instance, in electric generators, the orientation of the loop (or coil) is deliberately changed to induce an alternating current. By rotating a loop in a magnetic field, the flux through the loop continuously changes, inducing an electromotive force (EMF) and generating electricity.
In transformers, the orientation of the primary and secondary coils relative to the magnetic field in the core is designed to maximize flux linkage and energy transfer. Similarly, in electric motors, the orientation of the rotor coils relative to the stator's magnetic field is carefully controlled to produce rotational motion.
The concept also extends to more advanced applications in physics and engineering. For example, in magnetic resonance imaging (MRI), the orientation of hydrogen atoms in the body relative to the applied magnetic field is crucial for generating detailed images. In particle accelerators, the orientation of magnetic fields is precisely controlled to guide charged particles along desired paths.
In conclusion, the relationship between magnetic field lines and loop orientation is a fundamental principle in electromagnetism. It determines the magnetic flux through a loop, which is essential for understanding and designing a wide range of electromagnetic devices and systems. By manipulating this relationship, we can harness the power of magnetism for numerous practical applications in technology and science.
Faraday's Law Equation and Applications
Faraday's Law of Electromagnetic Induction is a fundamental principle in electromagnetism, describing how a changing magnetic field induces an electromotive force (EMF) in a conductor. The complete Faraday's Law equation is expressed as:
ε = -N(dΦ/dt)
This induced EMF equation encapsulates the essence of electromagnetic induction. Let's break down each component:
- ε (epsilon) represents the induced electromotive force (EMF) in volts.
- N is the number of turns or loops in the conductor.
- dΦ/dt denotes the rate of change of magnetic flux over time.
- The negative sign (-) indicates the direction of the induced current, following Lenz's Law.
The significance of the negative sign in Faraday's Law is crucial. It represents Lenz's Law, which states that the induced current flows in a direction that opposes the change in magnetic flux that caused it. This opposition is nature's way of conserving energy and maintaining equilibrium.
The change in flux (dΦ/dt) is a key component of the equation. It represents how quickly the magnetic field lines are cutting through the conductor. Factors affecting this change include the strength of the magnetic field, the area of the loop, and the angle between the field and the loop.
When dealing with multiple loops, Faraday's Law applies cumulatively. The total induced EMF is the sum of the EMFs induced in each individual loop. This is why the number of turns (N) is multiplied by the rate of change of magnetic flux in the equation. More loops result in a stronger induced EMF, which is why transformers and electric generators often use coils with many turns.
Faraday's Law finds numerous applications in real-world scenarios:
- Electric Generators: These devices convert mechanical energy into electrical energy by rotating a coil in a magnetic field, inducing an EMF.
- Transformers: Used to step up or step down voltage in power distribution systems, transformers rely on Faraday's Law to transfer energy between coils.
- Induction Cooktops: These modern cooking appliances use rapidly changing magnetic fields to induce currents directly in the cookware, generating heat.
- Electric Guitar Pickups: The vibration of metal strings in a magnetic field induces a current in the pickup coils, converting mechanical vibrations into electrical signals.
- Electromagnetic Flow Meters: Used to measure the flow rate of conductive liquids in pipes by inducing a voltage proportional to the flow velocity.
- Wireless Charging: This technology uses electromagnetic induction to transfer energy between two coils, charging devices without direct electrical contact.
Understanding Faraday's Law is crucial for engineers and physicists working on various electromagnetic applications. It explains how we can generate electricity on a large scale, transform voltages for efficient power transmission, and create innovative devices that harness the power of electromagnetic induction.
In conclusion, Faraday's Law, represented by the equation ε = -N(dΦ/dt), is a cornerstone of electromagnetism. It quantifies how changing magnetic fields induce electromotive forces, taking into account the number of loops and the rate of flux change. The law's applications span from large-scale power generation to everyday consumer electronics, demonstrating its fundamental importance in modern technology and energy systems.
Methods of Changing Induced EMF
Induced electromotive force (EMF) is a fundamental concept in electromagnetism, and understanding the methods of changing it is crucial for various applications in physics and engineering. There are three primary methods of altering induced EMF: changing the magnetic field strength, modifying the loop's area, and adjusting the angle between the magnetic field and the loop. Each of these methods has unique implications for the induced current and magnetic field.
1. Changing the Magnetic Field Strength: The first method involves altering the strength of the magnetic field. According to Faraday's law of induction, the induced EMF is directly proportional to the rate of change of magnetic flux. By increasing or decreasing the magnetic field strength, we can significantly impact the induced EMF. For example, in a simple generator, rotating a magnet faster near a coil of wire will increase the rate of change of the magnetic field, resulting in a higher induced EMF. This principle is widely used in power generation, where the rotation speed of turbines affects the output voltage.
2. Changing the Loop's Area: The second method focuses on modifying the area of the loop or coil exposed to the magnetic field. The induced EMF is proportional to the area of the loop perpendicular to the magnetic field lines. By increasing the loop's area, we can capture more magnetic flux, leading to a higher induced EMF. Conversely, decreasing the area will reduce the induced EMF. This principle is applied in transformers, where the number of turns in the primary and secondary coils affects the voltage transformation. It's also relevant in the design of electric motors, where the size and shape of the armature coils influence the motor's efficiency.
3. Changing the Angle: The third method involves adjusting the angle between the magnetic field lines and the plane of the loop. The induced EMF is maximum when the magnetic field lines are perpendicular to the loop's plane and minimum (zero) when they are parallel. This relationship is described by the cosine of the angle between the field lines and the normal to the loop's plane. In practical applications, such as in AC generators, this principle is utilized by rotating a coil in a fixed magnetic field, causing a continuous change in the angle and thus producing an alternating current.
The implications of these changes on the induced current and magnetic field are significant. The induced current, according to Lenz's law, always flows in a direction that opposes the change in magnetic flux that caused it. This means that as we increase the induced EMF through any of the three methods, the induced current will also increase, creating its own magnetic field that opposes the original field. This opposition is the basis for electromagnetic braking systems and eddy current damping in various devices.
In the case of changing magnetic field strength, a rapidly changing field will induce stronger currents, which in turn create stronger opposing magnetic fields. This principle is used in induction heating, where rapidly alternating magnetic fields induce currents in conductive materials, causing them to heat up. When changing the loop's area, larger areas allow for greater induced currents, which can be beneficial in power generation but may also lead to increased energy losses in transformers if not properly managed.
The angle change method is particularly important in AC power generation and motors. As the angle between the magnetic field and the loop changes, the induced EMF varies sinusoidally, resulting in alternating current. This forms the basis of our AC power systems and the operation of synchronous motors. The continuous change in angle ensures a constant flow of induced current, which is essential for sustained power generation or motor operation.
Understanding and manipulating these three methods of changing induced EMF is crucial in the design and optimization of various electromagnetic devices. Engineers and physicists use these principles to develop more efficient generators, motors, transformers, and other electromagnetic equipment. By carefully controlling the magnetic field strength, loop area, and angle, they can fine-tune the performance of these devices to meet specific requirements in power generation, electric vehicles, and countless other applications.
In conclusion, the ability to change induced EMF through these three methods provides a powerful toolkit for controlling electromagnetic phenomena. Whether it's generating electricity, powering motors, or designing advanced sensors, the principles of changing magnetic field strength, loop area, and angle are at the heart of modern electromagnetic technology. As we continue to advance in fields like renewable energy and electric transportation, these fundamental concepts will remain crucial in driving innovation and efficiency in electromagnetic
Practical Applications and Experiments
Faraday's Law of Electromagnetic Induction is not just a theoretical concept; it's a principle that powers many of the technologies we use every day. Let's explore some exciting practical applications and fun experiments that bring this law to life!
One of the most common applications of Faraday's Law is in generators. These devices convert mechanical energy into electrical energy, powering our homes, businesses, and cities. When you flip a light switch, you're benefiting from Faraday's discovery! Generators work by rotating a coil of wire within a magnetic field, inducing an electric current. This principle is used in everything from massive power plants to portable generators for camping trips.
Transformers are another crucial application of Faraday's Law. These devices are essential for our power distribution systems, allowing electricity to be transmitted over long distances efficiently. Transformers use electromagnetic induction to step up or step down voltage levels, ensuring that the electricity reaching our homes is at a safe and usable level. Next time you see those large boxes on utility poles, remember that Faraday's Law is at work!
A more recent and innovative application is the induction cooktop. These sleek, modern cooking surfaces use electromagnetic induction to heat pots and pans directly, without heating the cooktop itself. When you place a magnetic cookware on an induction cooktop, it creates a magnetic field that induces electric currents in the pot, heating it rapidly and efficiently. It's a safer and more energy-efficient cooking method, all thanks to Faraday's Law!
Now, let's get hands-on with some simple experiments you can try at home or in the classroom to demonstrate Faraday's Law:
- Magnet and Coil Experiment: Wind a coil of wire around a cardboard tube and connect the ends to a sensitive galvanometer or multimeter. Move a strong magnet in and out of the tube quickly. You'll see the meter deflect, indicating an induced current!
- Homemade Generator: Attach several strong magnets to a rotating shaft (like a hand-crank or small motor). Place a coil of wire near the rotating magnets and connect it to an LED. As you turn the shaft, the changing magnetic field will induce a current, lighting up the LED!
- Electromagnetic Swing: Create a pendulum with a strong magnet attached to the end. Set up two coils of wire on either side of the pendulum's path. Connect each coil to an LED. As the magnet swings through the coils, it will induce a current, causing the LEDs to blink alternately!
These experiments are not only fun but also provide a tangible understanding of how changing magnetic fields can induce electric currents. They demonstrate the core principle behind generators, transformers, and induction cooktops. By exploring these practical applications and conducting hands-on experiments, you're following in the footsteps of Michael Faraday himself, who was a master of both theoretical understanding and practical demonstration.
So, the next time you use an electric appliance, ride in an electric vehicle, or cook on an induction stove, take a moment to appreciate the brilliant simplicity of Faraday's Law. It's a testament to how fundamental scientific principles can lead to world-changing technologies. Keep experimenting, stay curious, and who knows? You might just invent the next big application of electromagnetic induction!
Conclusion
Faraday's Law is a fundamental principle in electromagnetic induction, stating that a changing magnetic field induces an electromotive force in a conductor. This law is crucial for understanding the generation of electricity in power plants and the operation of transformers. The introduction video provides a clear visualization of this concept, making it easier to grasp the relationship between magnetic flux and induced current. The importance of Faraday's Law extends beyond theoretical physics, playing a vital role in modern technology and energy production. To deepen your understanding, we encourage you to explore further resources and conduct simple experiments at home. Try creating a basic generator or investigate the effects of changing magnetic fields on different materials. By engaging with these hands-on activities, you'll gain a more profound appreciation for the practical applications of Faraday's Law in our daily lives. Don't hesitate to seek out additional educational materials or join online forums to discuss your findings with fellow enthusiasts.
Faraday's Law
Faraday's Law of Induction is a fundamental principle in electromagnetism that describes how a change in magnetic flux can induce an electromotive force (EMF) in a loop of wire. This law is crucial for understanding how electric generators, transformers, and many other electrical devices operate.
Step 1: Understanding Faraday's Law
Faraday's Law states that the induced EMF in a loop is proportional to the rate of change of magnetic flux through the loop. The magnetic flux (ΦB) is defined as the product of the magnetic field (B), the area of the loop (A), and the cosine of the angle (θ) between the magnetic field and the normal to the loop's surface. Mathematically, it is expressed as:
ΦB = B × A = B × A × cos(θ)
Where B is the component of the magnetic field perpendicular to the loop's surface. The unit of magnetic flux is the Weber (Wb), which is equivalent to Tesla meter squared (T·m²).
Step 2: Factors Affecting Induced EMF
The induced EMF depends on several factors:
- The rate of change of magnetic flux (dΦB/dt).
- The area of the loop (A).
- The angle (θ) between the magnetic field and the normal to the loop's surface.
According to Faraday's Law, the induced EMF (ε) is given by:
ε = -dΦB/dt
The negative sign indicates the direction of the induced EMF, as described by Lenz's Law, which states that the induced EMF will always oppose the change in magnetic flux that caused it.
Step 3: Magnetic Flux Components
To understand the components of magnetic flux, consider a magnetic field (B) with two components: B (perpendicular to the loop) and B (parallel to the loop). In Faraday's Law, only the perpendicular component (B) contributes to the magnetic flux through the loop.
For example, if the magnetic field is perpendicular to the loop, the angle θ is 0 degrees, and cos(0) = 1, resulting in maximum magnetic flux. Conversely, if the magnetic field is parallel to the loop, the angle θ is 90 degrees, and cos(90) = 0, resulting in zero magnetic flux.
Step 4: Calculating Magnetic Flux
To calculate the magnetic flux (ΦB), use the formula:
ΦB = B × A × cos(θ)
Consider two scenarios:
- If the loop is parallel to the magnetic field (θ = 90°), the magnetic flux is zero because cos(90°) = 0.
- If the loop is perpendicular to the magnetic field (θ = 0°), the magnetic flux is maximum because cos(0°) = 1.
Step 5: Practical Applications
Faraday's Law has numerous practical applications, including:
- Electric generators: Convert mechanical energy into electrical energy by rotating a coil within a magnetic field, inducing an EMF.
- Transformers: Transfer electrical energy between circuits through electromagnetic induction, changing voltage levels.
- Induction cooktops: Use changing magnetic fields to induce currents in cookware, generating heat.
Understanding Faraday's Law is essential for designing and analyzing these devices, ensuring efficient and effective operation.
Step 6: Summary
In summary, Faraday's Law of Induction describes how a changing magnetic flux induces an EMF in a loop. The induced EMF depends on the rate of change of magnetic flux, the area of the loop, and the angle between the magnetic field and the loop's surface. By understanding and applying Faraday's Law, we can design and optimize various electrical devices and systems.
FAQs
-
What is Faraday's Law of Electromagnetic Induction?
Faraday's Law states that the induced electromotive force (EMF) in a closed loop is equal to the negative rate of change of magnetic flux through the loop. It's expressed mathematically as ε = -dΦ/dt, where ε is the induced EMF and dΦ/dt is the rate of change of magnetic flux.
-
How does changing the loop's area affect induced EMF?
Changing the loop's area affects the amount of magnetic flux passing through it. Increasing the area exposed to the magnetic field will increase the induced EMF, as more magnetic field lines can pass through the larger area. This principle is utilized in the design of electric generators and transformers to maximize efficiency.
-
What are some practical applications of Faraday's Law?
Faraday's Law has numerous practical applications, including:
- Electric generators for power production
- Transformers in power distribution systems
- Induction cooktops for efficient cooking
- Electromagnetic flow meters for measuring conductive liquid flow
- Wireless charging technology for electronic devices
-
How does the angle between magnetic field lines and the loop affect induced EMF?
The angle between magnetic field lines and the loop's normal affects the magnetic flux. Maximum EMF is induced when the field lines are perpendicular to the loop's surface (θ = 0°, cos θ = 1). When the field lines are parallel to the surface (θ = 90°, cos θ = 0), no EMF is induced. This relationship is described by the equation Φ = BA cos θ, where Φ is magnetic flux, B is magnetic field strength, A is loop area, and θ is the angle.
-
What is the significance of the negative sign in Faraday's Law equation?
The negative sign in Faraday's Law equation (ε = -dΦ/dt) represents Lenz's Law. It indicates that the induced current flows in a direction that opposes the change in magnetic flux that caused it. This opposition is nature's way of conserving energy and maintaining equilibrium. Understanding this concept is crucial for predicting the behavior of induced currents in various electromagnetic applications.
Prerequisite Topics for Understanding Faraday's Law
Faraday's law is a fundamental principle in electromagnetism, but to truly grasp its significance, it's crucial to have a solid understanding of several prerequisite topics. These foundational concepts provide the necessary context and background knowledge to fully appreciate the intricacies of Faraday's law.
One of the key prerequisites is understanding the rate of change. This mathematical concept is essential when dealing with Faraday's law, as it directly relates to the rate of change of magnetic flux. In Faraday's law, we're concerned with how quickly the magnetic field is changing, which is why a strong grasp of rate of change is crucial. It allows us to quantify and analyze the variations in magnetic flux over time, a cornerstone of electromagnetic induction.
Another vital prerequisite topic is the magnetic field due to a long straight wire & force between two parallel wires. This concept provides the foundation for understanding how magnetic fields behave and interact. When studying Faraday's law, we often deal with loops of wire in magnetic fields. Knowing how the angle between the magnetic field and the loop affects the induced current is crucial. This prerequisite topic helps build that understanding, making it easier to visualize and calculate the effects described by Faraday's law.
Lastly, familiarity with electric generators & counter EMF (Back EMF) is incredibly beneficial when delving into Faraday's law. Electric generators are practical applications of Faraday's law, demonstrating how changing magnetic fields can induce electrical currents. Understanding the principles behind generators provides real-world context for Faraday's law and helps illustrate its importance in modern technology. Additionally, the concept of counter EMF, or back EMF, is directly related to Lenz's law, which is often studied alongside Faraday's law and helps explain the direction of induced currents.
By mastering these prerequisite topics, students can approach Faraday's law with a more comprehensive understanding. The rate of change concept provides the mathematical tools, the understanding of magnetic fields offers the physical framework, and knowledge of electric generators gives practical context. Together, these prerequisites create a solid foundation upon which a deeper understanding of Faraday's law can be built, enabling students to better grasp its implications and applications in the field of electromagnetism.
In this lesson, we will learn:
- Faraday’s Law
- Faraday’s Law of Induction
- Different methods of inducing emf.
Notes:
Faraday’s Law
- According to Faraday the induced emf is proportional to the following factors:
- The rate of change of magnetic flux through the loop, .
- The loop’s area () and angle ().
Unit: tesla.meter2 = weber ()
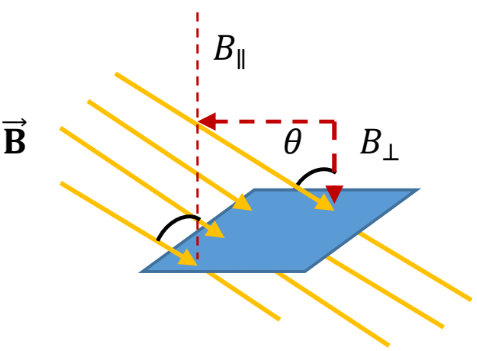
: is the component of the magnetic field perpendicular to the face of the loop.
: is the angle between magnetic field and a line perpendicular to the face of the loop.
Notes:
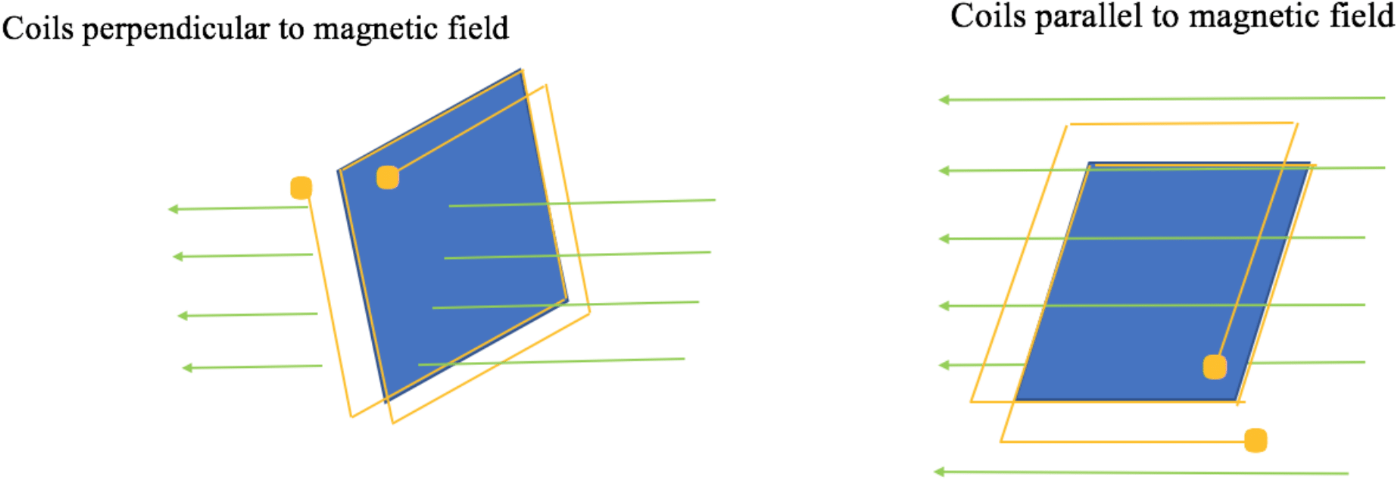
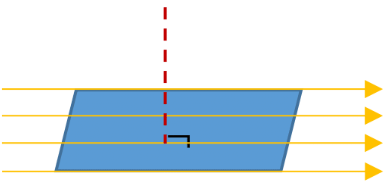
a. When the loop is parallel to , =90° and 0
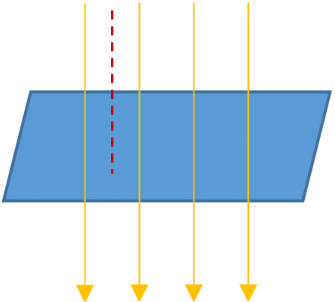
b. When the loop is perpendicular to , =0 and
- Number of line per unit area is proportional to the filed strength, therefore, is proportional the the total number of lines passing through the loop’s area
- When the loop is parallel to , no filed line will pass through the loop, =0
- When the loop is perpendicular to , maximum number of lines will pass through the loop, is maximum.
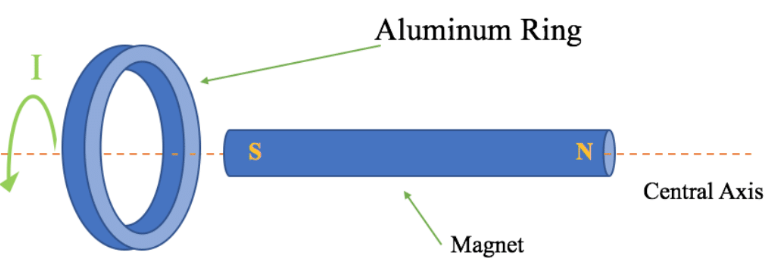
- The flux through the loop changes by the amount of over interval of time, therfore, the induced emf is calculated as follows;
if the loop contains N loops, the induced emf in each loop adds up;
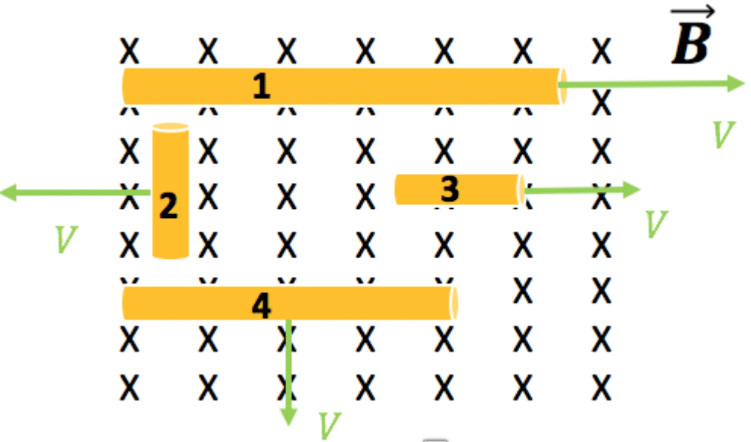
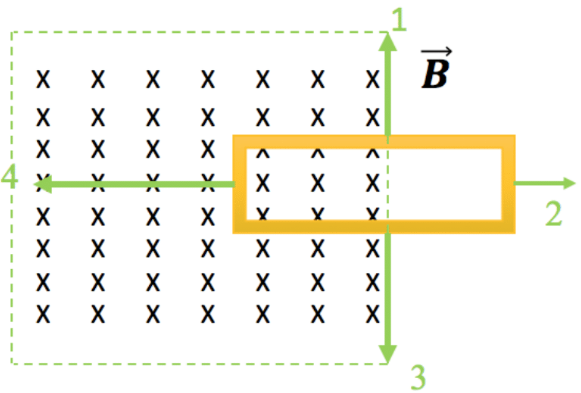
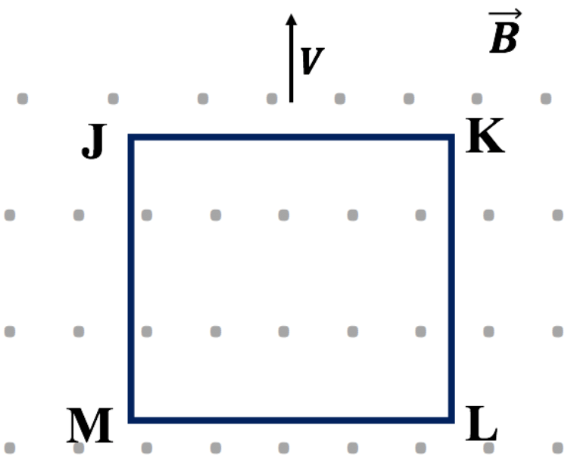
In general, there are three different ways to change the magnetic flux;
- Changing B
It could be done by changing the number of the loops, which in return changes the strength of the filed.
More number of loops larger magnetic field bigger flux - Changing A
The current can be induced by changing the area of the loop. As flux through the loop changes, the current is induced to maintain the the original flux. - Changing
- The current can be induced by rotating the coil in a magnetic field. The flux through the coil goes from maximum to zero.
Note: decreasing the area of the loop, induces a current, the induced current acts in a direction to increase the magnetic field in the original direction. Therefore, a magnetic field into the page is induced.
