Law of Conservation of energy
On a daily basis we talk about energy, we have the sense that energy moves us, energy makes the world work but, what is energy?
In simple terms energy can be defined as the capacity to do work, and as you already know, work is the result of a force applied through a distance resulting in a displacement, thus energy ends up being the capacity of something somewhere producing motion. So yeah, we need energy in our lives, funny enough, in the universe there is truly just two things that form everything else: matter and energy, how can that be? Let us continue with our lesson.
What is the law of conservation of energy
The law of conservation of energy states that energy cannot be created or destroyed, only transformed. This means that through time, the amount of energy existent remains the same it just has been converted from one form to the other depending on the cycles that it is passing through at any certain moment in time.The conservation of energy formula can be written simply as:
Meaning the initial energy is equal to the final energy in an isolated system, no matter the processes that this system develops and passes through over time.
It is important to note that this law, as we just mentioned above, is in reference of isolated systems, meaning that if we are to take a certain reference frame we could have disappearance of energy if this is not isolated. For example: Think of a pot with water on a stove, the stove is a maximum heat and the water is starting to boil. Remember that heat is energy, and so, if we take the pot only as our reference frame then energy is just coming out of nowhere and we could say is being created in the system, the same could be applied at the moment of time in which the water starts to boil and evaporates and goes away from the system. For this small reference frame we would be seeing how energy is being created into the system and then disappears from it, the reason is that this system is NOT isolated and so if we expand our reference frame to the whole kitchen we can clearly see that the energy is just passing through being released from the stove, to the heating water to then the vapor going into the air.
Now think of a truly isolated system, lets say any room which would be completely hermetically and temperature-wise isolated from the rest of the world. If you are inside, you will notice it doesn't change in temperature because heat is not being injected to the system. You could argue that if you rub your hands you will get them warmer, but that is just the energy inside your own body producing the effect, and so nothing is coming in and nothing is going out. If you were to make a fire inside this room you would need some kind of fuel for it to happen, and this is because fuel contains chemical potential energy and so, the energy was already inside the room, if you make the fire it would go from being stored in the fuel to be burning bright in a fire (still, dont do it, is an isolated room, a fire would consume your oxygen!). You cannot create or destroy energy in an isolated system, you can only use what you already have in the most effective and convenient way.
But if energy cannot be destroyed or created, only transformed? How did the energy come up to be in the first place?
Honest response: no one knows for sure since we cannot go back in time and observe what happened during the big bang, but we can observe how the energy contained in the universe is not changing, it has just transformed through its close to its 14 billion years of history. We can study the environment in the early universe thanks to deep space astronomical observations of the cosmic microwave background, we can also study the life-stages of stars and see how they are massive powerhouse units which collect energy during their formation and then release it to their surroundings during their lifetime, and we of course know how that energy released through nuclear fusion in stars such as our Sun, travels through the universe arriving to their planetary systems. For the case of our Solar System, the energy released (note here, NOT created, since the Sun itself gathered this energy and kept it as potential energy while forming its massive self, and then releases it through nuclear fusion when massive enough) from the Sun arrives to the surface of the Earth and thanks to our atmosphere it stays for a while to warm our environment, to trigger photosynthesis in plants during their metabolic processes, which will be then the same energy herbivores will consume as sugars from the plants, and then carnivores from the herbivores they hunt
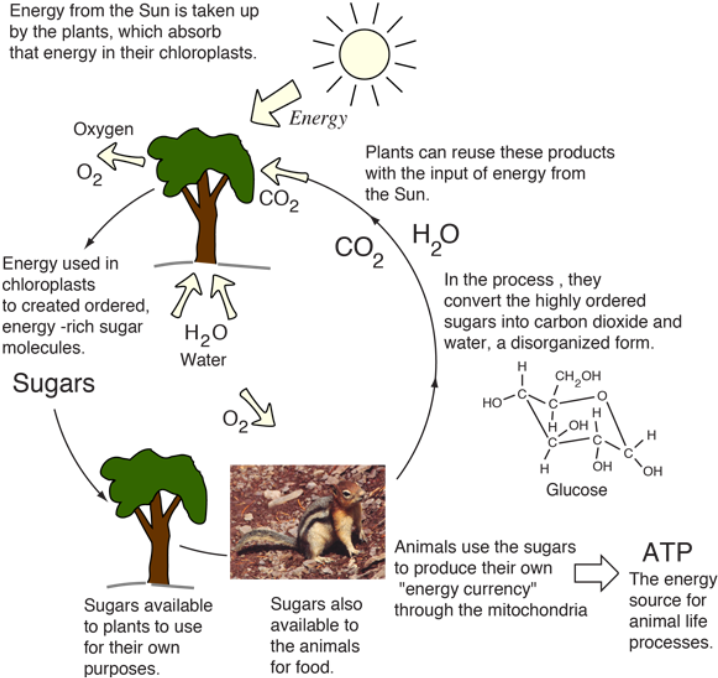
As you can see, energy passes through all of this cycle, and where will it end? Living organisms die and go back to the Earth and some day, approximately 5 billion years from now our Sun will release its outer layers and explode into a planetary nebula, expelling with it not only most of its contents but also destroying the Solar system and its planets leaving behind just a simple white dwarf star. At this point all of the elements released from the solar system, including everything that composes us, will start to slowly rain back down towards higher density areas within our galaxy and through time will at some point be part of a new star system which will probably contain a planetary system and even maybe a planet resembling our own, just like there probably was already before in order for us to be here.

Credit: NASA, ESA, and the Hubble SM4 ERO Team (Public Domain)
And this is all because the universe as a whole is taken as our immense isolated system. We cannot go outside and check if there is anything else (although there are many theories about it, but still, not even energy can make the relativistic trip out). So where did this mass-energy enormous system came from? We dont know, but it is closed and isolated and so, it remains unchanged on its amount of energy. In this and many other ways, the law of conservation of energy has been observed and proven for a long time. The same amount of energy that exists in the universe has always been here and will be until the end of the universe itself (if there is such thing at some point, we dont truly know). Go ahead and explore the world with the news eyes of this knowledge, what other cycles can you find around you where you can see energy being used and transformed from one stage to the next? Explore the world for a better understanding!
If you are interested on reading about the conservation of energy in the universe or the formation of the solar system, visit the links provided here of ask us about these topics.
Mechanical energy
After this interesting note, we will concentrate on mechanical energy for our lesson. In our everyday life we will see energy in different forms and one of the easiest to identify is mechanical energy. Mechanical energy is the energy of motion and position, explicitly, is the energy that describes and reigns simple objects positions, motions, displacements and everything in between.
Mechanical energy has two different components, each very important on itself which describe the state of an object in a reference plane. These two components are potential energy and kinetic energy, and so, mechanical energy can simply be defined as the addition of potential and kinetic energy.
Where E represents Energy (or total energy of the system), P is to denote the potential energy of the system and K is to denote the Kinetic energy.
Let us take a moment to explain each of the two mechanical energy types we can find so you are sure on how to work through problems later on. Let us start with the most obvious one: Kinetic energy.
Kinetic energy is the energy of motion, every time you see and object moving, it has kinetic energy. By now you have seen the physical definition of work, which is the application of a force resulting in a displacement of an object. We can relate this to kinetic energy since a displacement means that the object will move and so, it will have kinetic energy. Thus, kinetic energy ends up being the work that an object needs to do in order to change its state, for example, to change from rest to arrive at a certain speed (gains kinetic energy), to change from a certain constant speed motion to stop and go to rest (loses kinetic energy) or from going in a constant speed motion, accelerating or decelerating into another different speed (gaining or losing kinetic energy depending on the speeding up or down).
Mathematically, kinetic energy can be defined with the next simple formula:
Notice how kinetic energy has two important variables as factors on its equation: mass (m) and speed (v), thus, the kinetic energy of an object depends on its mass and the speed it is moving, if an object is not moving, its speed is zero and thus, it doesnt have kinetic energy (K=0).
Potential energy on the other hand is the energy of position and location. You may be wondering, how can an object contain energy if is not doing anything, well, in simple terms, energy can be stored too and so, that is probably why it has been called potential energy, since you have energy on a certain body with the potential to do something and ready to be used. Thus, objects at rest can have potential energy, it just depends on their position or location within a certain system and reference frame, in relation to other objects or any processes inside itself too.
A body can have different types of potential energies, it will all depend on the factors of the system you are exploring or studying. Since we are all living in one planet, Earth, one of the most important types of potential energy is gravitational potential energy (some people just call it gravitational energy too). In general, gravitational potential energy is just the potential energy of an object related to its location from another very massive object (or objects), since we will start by looking into cases where we study the behaviour of bodies (or any object) in everyday life, this means we will be looking into systems in which our reference frame is usually our world, our own planet Earth, and so, the gravitational potential energy we will be solving for is the potential energy which we have due our position in relation to the most massive object in our inertial reference frame: Earth.
Mathematically speaking, gravitational potential energy is defined as:
Let us explore the components of the equation for the potential energy of an object here on Earth: m is used to denote mass just like in the formula for kinetic energy, and so, the mechanical energy of a body always depends on its mass as you can see. The variable g means gravitational acceleration and this is because here on Earth, we are all subject to its gravitational field, so every object on this planet has a certain acceleration towards it, that is a constant value (since the mass of the planet is not changing, at least not on a substantial way) that you just need to remember: . And h is for height, in general this variable represents the distance between the body being studied and the very massive object in the system with it (for us Earth), so it could be represented with the letter d as in distance, for example. Using h was probably selected as the standard since, if we think on our distance to the Earth, it truly really means how high can we go from the surface of the planet, or the height we can obtain.
Knowing the meaning of each factor on the equation for gravitational potential energy allows us to clearly see how potential energy is all about location or position. Easily put, if an object is located high from the ground, it will have certain potential energy, the highest, the more potential energy within it. But if this same object is located in the ground, h=0 and so, it doesnt have any potential energy (P=0), because is already together with the massive object is being studied in relation with.
Note, gravitational potential energy in general is the potential energy of the location of bodies in relation to their location from one another, that means, we all have gravitational potential energy in respect to each and every other object or living body in our system (so you and your friends you and your desk, you and everything that has mass). This is because we have a mass, and so it all comes from gravitational forces! As long as something has a mass, they have a gravitational field, so you and every single one of us, or every animal, plant and object, has a gravitational field producing an acceleration towards each other due to it! Why dont we think on that? Realizing this is absolutely fantastic, but since we are very small masses all of these effects are completely negligible (you wont have object falling towards you in the way we all fall towards and stay in the surface of the Earth) and we can just go through our lives ignoring them. If we look at the gravitational potential energy we would have due to our own masses in relation to others (lets say you and a friend) the accelerations produced (the values for g in that system) would be so tiny that you wouldnt be able to come up with a meaningful amount of potential energy and so we just let it be.
Now that we know what potential and kinetic energy are, let us rewrite the formula for the total mechanical energy of a system:
Therefore, we can now come up with a conservation of mechanical energy formula by rewriting equation 1 in terms of the potential and kinetic energy definitions:
Where:
= mass
= acceleration due to gravity (9.8 )
= initial height
= initial speed
= final height
= final speed
So an object can have stored energy (potential energy) depending where it is located, internal processes or its position and this energy is ready to use. When the object starts moving this energy gets transformed into kinetic energy and so mechanical processes happen through a continuous cycle where energy gets transformed from one another depending on how the object behaves.
Of course this is in ideal cases where no external factors affect the system in question (so the system is isolated), but in real life we will have some energy in systems lost through heat due friction, air resistance and other factors we and every object has to interact with as long as they are in the atmosphere and surfaces of this world (and any other for that matter) so let us take a look at conservation of energy problems so we can see all of these factors in action.
Examples of Law of conservation of energy
We will explain the law of conservation of energy in terms of mechanical energy systems and solve a few exercises in the next section. To begin with, our first example will contain an ideal case where you will be shown how energy conservation works while lacking friction or air resistance, and then, we will finish by adding the effects of these important forces into our last two problems.
Example 1
A ball with mass 0.050 kg is thrown straight up into the air to an initial velocity of 5 m/s. What is the velocity of the ball when it reaches a height of 0.5 m? Assume no friction due to air resistance.
We identify each variable first:
= 0.050 kg
= acceleration due to gravity (9.8 )
= 0
= 5 m/s
= 0.5 m
= ?
Using the law of conservation in equation 6 to solve for the final velocity we have that:
substituting the variables for their values:
Now, as mentioned before, for the next two conservation of energy examples we will take into consideration the influence of air resistance and friction in our problems.
Example 2
= 0.2 kg
= 9.8 )
= 0
= 45 m/s
= ?
= 0
= 45J = 45 kg
Notice the final speed is zero, because at its highest point, the ball will be momentarily at rest before starting to fall back down. So we solve for the final height:
since the initial height and the final speed are zero, we can rewrite this as:
solving for the final height:
Therefore, the highest altitude the ball reaches is 80.357m.
Example 3
A 2.5 kg book initially at rest slides 20 m down an incline. Final velocity at the bottom of the incline is 8m/s. What is the force of friction acting on the book as it slides down the incline?
The diagram below shows the system we are solving for.
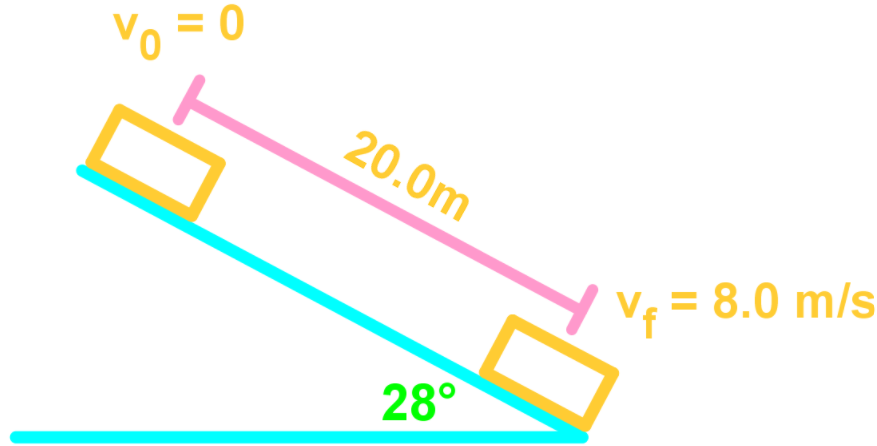
For this case, in the initial part the total mechanical energy of the system is comprised of the initial potential and initial kinetic energies, but at the end, some energy has been lost to friction, therefore, we define the law of conservation of energy with the next equation reflecting that.
Now let us expand on this for a little bit: At the beginning, the book is at rest and so it doesnt have kinetic energy. After the book has slided down the incline, it will be on the ground and so its height is zero, thus it doesnt have a final potential energy. Furthermore, do not forget that while the book was going down, it lost some energy due friction (which we have represented as , the work against friction). So the final mechanical energy (which is the sum of the final potential and final kinetic energy) is less than the initial mechanical energy since some of it was lost to friction, and thus, that is why we have to add it at the end. Therefore, our formula ends up being:
Now let us list the variables involved:
= 2.5 kg
= 9.8
= (20 m) sin (28°)
= 0 (at rest)
= 0
= 8 m/s
=
Notice the initial height, equal to the sine of the 28-degree angle times the 20 meters was found using right triangle trigonometry.
To solve this problem we need to remember that work is equal to the applied force times the distance of the displacement produced in the body. In this case, for the work against friction we have that is equal to the friction force times the displacement (the length of the incline which is the length the book is going to displace. And so, for this problem we will solve for the Force of friction:
Solving for
Thus, the force of friction acting on the book as it slides down the incline is equal to 7.5 N.
After solving these problems and talking about the conservation of energy throughout the universe, and in everything we do, you might want to take a look into the law of conservation of energy in chemical reactions reactions or even look at how there are other conservation laws very important in physics.
- Conservation of mechanical energy
- When to use conservation of energy to solve problems
Notes:
- Energy is a conserved quantity: it can change forms and be transferred from one place to another, but it cannot be created or destroyed. For a process where energy changes forms or gets transferred, we can say that total energy before the process is equal to total energy after, or
- Mechanical energy is energy that an object has due to its motion and position. It is the sum of the object's kinetic energy () and potential energy ().
- In real world processes, the mechanical energy an object possesses can be "lost" to other non-mechanical forms of energy. (The energy is lost in the sense that it is no longer in the object, but it is not destroyed since it gets transferred to the object's environment.)
- For example, a box that is sliding across the floor has a certain amount kinetic energy, but eventually will come to a stop due to friction. The friction between the box and the floor converts the kinetic energy of the box to heat energy. When the box comes to a stop, all of the kinetic energy it originally had has been converted to heat: the equation could be expressed as for this process
- Energy is always conserved, but a special case when solving problems is when an object doesn't lose or gain any mechanical energy to/from its environment during a process. In this case, rather than saying total energy of the object and its environment is conserved (which is always true), we can say the total energy of that particular object is conserved (which is only true sometimes). This means that the total mechanical energy it has before the process is the same as after, though the balance of kinetic and potential energy it has may change. The equation in these cases becomes . A common type of process where this is true is objects that move under the influence of gravity without any other external forces that cause mechanical energy to be lost as heat (such as friction or air resistance).
- For example, imagine a book balanced right at the edge of a bookshelf with 10 J of stored gravitational potential energy, but no kinetic energy. If the book falls, the 10 J of potential energy would be converted to kinetic energy over the course of the fall. If air resistance is negligible, all the potential energy is converted to kinetic energy and the book would have 10 J of kinetic energy the moment before it hits the ground. Additionally, conservation of energy means that at any point during the fall the total energy of the book is 10 J: the sum of the book's kinetic energy (from its current speed) and potential energy (from its remaining height above the ground) always adds to 10 J.
- Once the book hits the ground, the energy of the book is no longer conserved, but total energy is conserved (as it always is). The kinetic energy of the book is dissipated as 10 J of heat during the collision with the ground.
Kinetic Energy
kinetic energy, in joules (J)
mass, in kilgrams (kg)
velocity, in meters per second (m/s)
Gravitational Potential Energy
gravitational potential energy, in joules (J)
acceleration due to gravity, in meters per second squared (m/s2)
height, in meters (m)
Conservation of Energy
sum of all initial energy, in joules (J)
sum of all final energy, in joules (J)