Introduction to Parallel Lines and Transversals
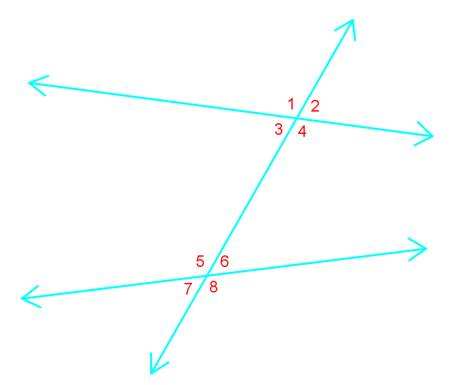
Welcome to our exploration of parallel lines and transversals! This fascinating topic is a cornerstone of geometry, and I'm excited to guide you through it. We'll start with a brief introduction video that sets the stage for our learning journey. This video is crucial as it visually demonstrates the key concepts we'll be discussing. Parallel lines, as you'll see, are lines that never intersect no matter how far they extend. A transversal is a line that crosses these parallel lines, creating interesting angle relationships. As we delve deeper, you'll discover how these angles relate to each other, forming patterns that are both beautiful and mathematically significant. Understanding these relationships is essential for solving more complex geometric problems. So, let's dive in and unravel the mysteries of parallel lines and transversals together! Remember, this knowledge will be a powerful tool in your mathematical toolkit.