Sign In
Sign Up
flag-ie

Linear Approximations and Tangent Planes: 3D Calculus Essentials
Dive into the world of 3D calculus with linear approximations and tangent planes. Master these crucial concepts for advanced problem-solving in mathematics, physics, and engineering.

  1. Intros0/2 watched
  1. 0/2
Now Playing:Linear approximations and tangent planes – Example 0a
Intros
0/2 watched
  1. Linear Approximations & Tangent Planes Overview:
  2. Linear Approximations & Tangent Planes Overview:
    Tangent Planes
    • A review of linear approximation
    • 2D = tangent lines, 3D = tangent planes
    • z=f(x0,y0)+fx(x0,y0)(x−x0)+fy(x0,y0)(y−y0)z=f(x_0,y_0)+f_x(x_0,y_0)(x-x_0)+f_y(x_0,y_0)(y-y_0)
    • An example
  3. Linear Approximations & Tangent Planes Overview:
    Linear Approximation with Multi-Variable Functions
    • Estimating a value of a 3D surface
    • Approximation for points "near" (x0,y0)(x_0,y_0),
    • An example
Linear approximations and tangent planes
Jump to:NotesConceptFAQsPrerequisites
Notes
Notes:

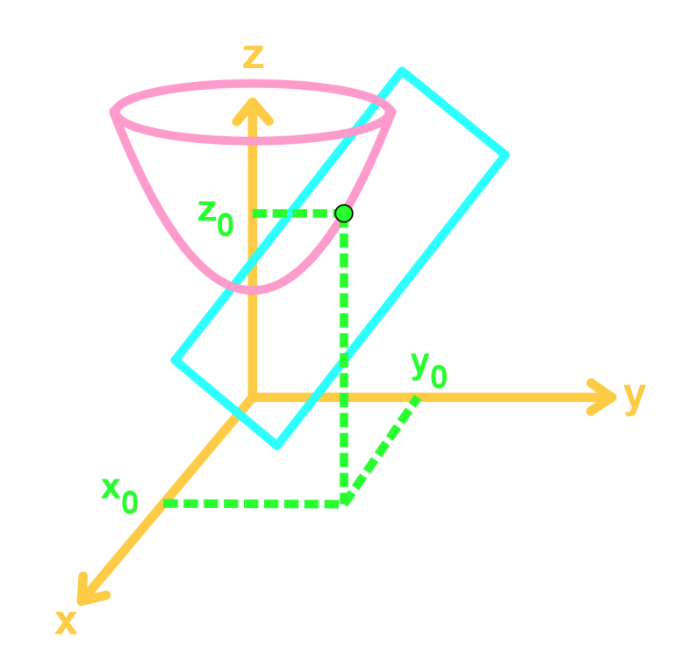
Tangent Planes
Recall that in Calc I, linear approximation is about finding a linear equation tangent to a curve at a point, and using it to estimate values of the curve "near" that point. The equation of the tangent line at point aa would be:
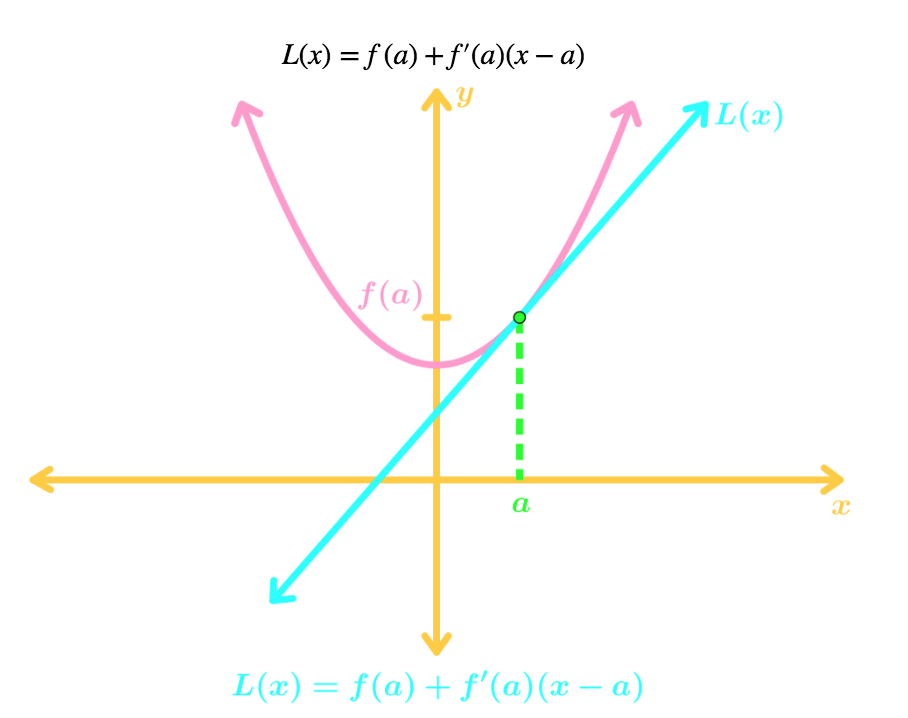
graph with tangent point
Calc III is similar, but now it is in 3D. Instead of 2D curves, we have 3D surfaces. Instead of tangent lines, we have tangent planes. The formula for the tangent plane at point (x0,y0)(x_0, y_0)is:

z=f(x0,y0)+fx(x0,y0)(x−x0)+fy(x0,y0)(y−y0)z=f(x_0,y_0)+f_x(x_0,y_0)(x-x_0)+f_y(x_0,y_0)(y-y_0)


Linear Approximation
Just like how we can estimate values of a 2D curve, can also estimate the value of a 3D surface near a point using linear approximation. We say that if we are at the point (x0,y0)(x_0,y_0), and we want to approximate a point near it (say (x,y)(x,y)), then

f(x,y)≈f(x0,y0)+fx(x0,y0)(x−x0)+fy(x0,y0)(y−y0)f(x,y) \approx f(x_0,y_0)+f_x(x_0,y_0)(x-x_0)+f_y(x_0,y_0)(y-y_0)

graph of linear approx
Concept

Introduction to Linear Approximations and Tangent Planes

Linear approximations and tangent planes are fundamental concepts in Calculus 3, extending the principles of linear approximation from Calculus 1 to multivariable functions. The introduction video serves as a crucial starting point, offering a visual representation of these complex ideas. In Calculus 1, linear approximation involves using tangent lines to estimate function values near a point. Calculus 3 expands this concept to three-dimensional space, where tangent planes approximate surfaces of multivariable functions. These planes provide a linear approximation of the function near a specific point, allowing for simplified analysis of complex surfaces. Understanding linear approximations and tangent planes is essential for grasping more advanced topics in multivariable calculus, such as directional derivatives and optimization problems. The video introduction helps students visualize these abstract concepts, making it easier to comprehend their applications in real-world scenarios. By building upon prior knowledge from Calculus 1, students can more effectively grasp these advanced mathematical tools and their significance in higher-level calculus.

FAQs

1. What is the difference between linear approximation in 2D and 3D?

Linear approximation in 2D uses tangent lines to approximate function values near a point, while in 3D, it uses tangent planes. In 2D, we work with a single variable and one derivative, whereas in 3D, we deal with two variables and partial derivatives. The 3D version provides a flat surface approximation of a more complex 3D surface.

2. How do you find the equation of a tangent plane?

To find the equation of a tangent plane: 1. Calculate the partial derivatives of the function with respect to x and y. 2. Evaluate these derivatives at the point of tangency. 3. Use the formula: z - z = fx(x, y)(x - x) + fy(x, y)(y - y), where (x, y, z) is the point of tangency and fx, fy are the partial derivatives.

3. What are the limitations of linear approximation in 3D?

Linear approximation in 3D is most accurate near the point of tangency and for small changes. It becomes less accurate as you move away from this point or when dealing with highly nonlinear functions. It may not work well for functions with rapid changes or discontinuities. Always compare approximated values with actual calculations to understand its limitations in specific applications.

4. How is linear approximation used in real-world applications?

Linear approximation is used in various fields: - Physics: Estimating orbital trajectories in celestial mechanics - Engineering: Analyzing structural deformations and circuit behavior - Computer Graphics: Calculating lighting and shading effects - Fluid Dynamics: Modeling airflow in preliminary aircraft designs These applications often involve simplifying complex systems for initial analysis or quick estimations.

5. What are some related topics to study after mastering linear approximations and tangent planes?

After mastering these concepts, consider studying: - Directional derivatives - Gradient vectors - Taylor series in multiple variables - Optimization in multivariable calculus - Lagrange multipliers These topics build upon the foundation of linear approximations and tangent planes, offering deeper insights into multivariable calculus and its applications.

Prerequisites

Understanding linear approximations and tangent planes is a crucial concept in calculus, but to fully grasp this topic, it's essential to have a solid foundation in several prerequisite areas. These fundamental concepts provide the building blocks for comprehending the more advanced ideas of linear approximations and tangent planes.

One of the most important prerequisites is the slope of tangent line. This concept is fundamental to understanding how linear approximations work, as they essentially use the tangent line to approximate a curve at a specific point. Mastering this topic will help you visualize how a tangent plane relates to a surface in three-dimensional space.

Another crucial prerequisite is the ability to work with the point-slope form of a line. This skill is invaluable when constructing linear approximations, as it allows you to easily create equations for tangent lines and planes using a point and a slope or normal vector.

While it may seem unrelated at first, estimating square roots is also a valuable skill. This concept helps develop your intuition for approximation, which is at the heart of linear approximations. Understanding how to estimate values will help you gauge the accuracy of your linear approximations.

As you delve deeper into the topic, you'll find that knowledge of the derivative of inverse trigonometric functions and the derivative of exponential functions becomes crucial. These advanced derivative techniques are often used when working with complex functions that require linear approximation.

Lastly, a solid understanding of the rate of change is essential. This concept is the foundation of derivatives, which are used to find the slopes of tangent lines and planes. Grasping how rates of change work will help you understand why linear approximations are effective and how they relate to the behavior of functions.

By mastering these prerequisite topics, you'll be well-prepared to tackle the complexities of linear approximations and tangent planes. Each of these concepts contributes to your overall understanding, providing the necessary tools and intuition to work with these advanced calculus ideas. Remember, a strong foundation in these basics will make your journey through more complex mathematical concepts smoother and more rewarding.