Sign In
Sign Up
flag-ie

Understanding the Multiplier Effect in Economics
Explore the multiplier effect, a key concept in economics that explains how initial changes in spending can lead to larger economic impacts. Learn its significance in shaping policies and predicting economic outcomes.

  1. Intros0/3 watched
  1. 0/3
Now Playing:The multiplier definitions – Example 0a
Intros
0/3 watched
  1. The Multiplier Definitions
    • Change in equilibrium and autonomous expenditure
    • Multiplier is always greater than 1
    • Why Multiplier> 1?
  2. Relationship Between the Multiplier & Slope of AE Curve
    • Formula for Change in Real GDP
    • Formula for Slope of AEAE curve
    • Algebraic Manipulation
    • Multiplier=11−Slope  of  AE  Curve Multiplier = \frac{1} {1- Slope\;of\;AE\;Curve}
Consumption & saving plans, and marginal propensity
Jump to:NotesConceptFAQsPrerequisites
Notes
The Multiplier Definitions

The AEAE curve can be shifted up? How? By the increase of autonomous expenditure.

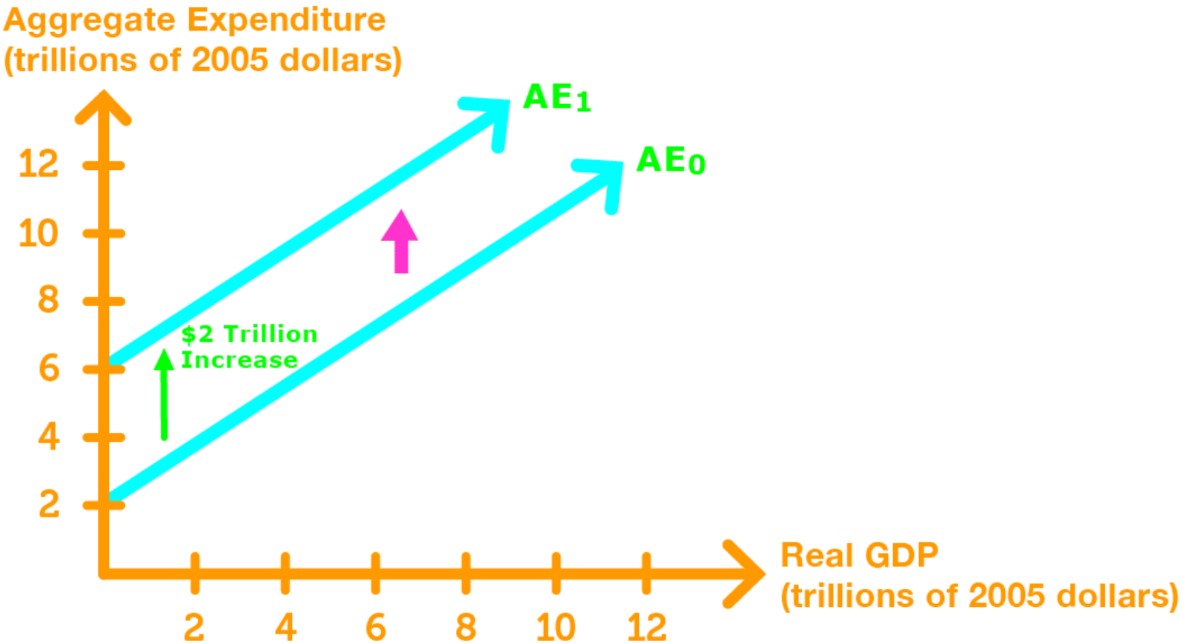
The Multiplier Definitions


If the AEAE curve shifts up, then the equilibrium expenditure changes. How can we find the new equilibrium?

We need to introduce a new concept.

Multiplier: shows the magnitude in how much equilibrium expenditure has changed in proportion with the change in autonomous expenditure.

In other words,

Multiplier=Δ  equilimrium  expenditureΔ  autonomous  expenditureMultiplier = \frac{\Delta \; equilimrium\;expenditure} {\Delta \; autonomous\;expenditure}

The multiplier is important because it helps us see the magnitude of the increase in the AEAE curve.

Note: The multiplier is always greater than 1. This is because the change in equilibrium expenditure is always bigger than the change in autonomous expenditure.

Suppose we have the following graph

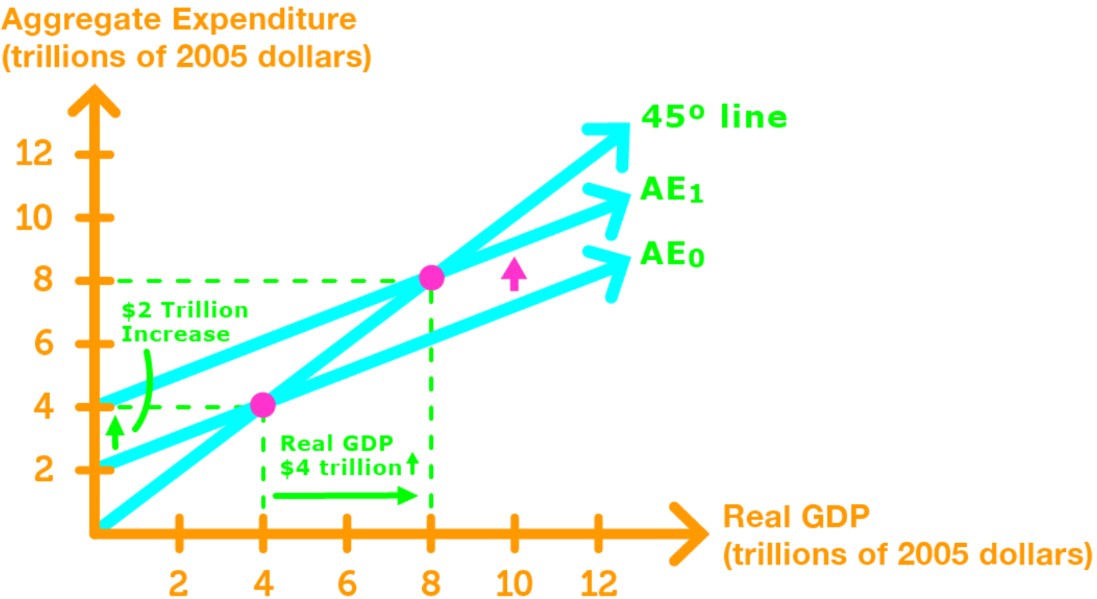
The Multiplier Definitions

Notice that:
  1. Equilibrium expenditure is at point AA

  2. The change in autonomous expenditure shifts the AEAE curve up by 2 trillion

  3. The new equilibrium expenditure is at point BB

  4. The change in equilibrium expenditure is 4 trillion

  5. Multiplier=Δ  equilimrium  expenditureΔ  autonomous  expenditure=42=Multiplier = \frac{\Delta \; equilimrium\;expenditure} {\Delta \; autonomous\;expenditure} = \frac{4} {2} = 2, which is greater than 1


Relationship Between the Multiplier & Slope of AECurve

If we know the slope of the AEAE curve, then we can also find the multiplier. How?

Recall that the change in real GDP is due to the changes in both induced expenditure and autonomous expenditure. In other words,

ΔY=ΔN+ΔA \Delta Y = \Delta N + \Delta A

Where:
ΔY \Delta Y = change in real GDP
ΔN \Delta N = change in induced expenditure
ΔA \Delta A = autonomous expenditure

We also know the slope of the AEAE curve as

Slope of AEAE Curve = ΔNΔY \frac{\Delta N} {\Delta Y}
ΔY ×\Delta Y \, \times Slope of AEAE Curve = ΔN \Delta N

Substituting this to the other equation gives

ΔY=ΔY ×\Delta Y = \Delta Y \, \times Slope of AEAE Curve + ΔA \Delta A
ΔY−ΔY ×\Delta Y - \Delta Y \, \times Slope of AEAE Curve = ΔA \Delta A
ΔY\Delta Y (1 - Slope of AEAE Curve) = ΔA\Delta A
ΔY=ΔA1−Slope  of  AE  Curve\Delta Y = \frac{\Delta A} {1- Slope\;of\;AE\;Curve}

Now dividing both sides by ΔA \Delta A gives us

ΔYΔA=11−Slope  of  AE  Curve=The  Multiplier\frac{\Delta Y} {\Delta A} = \frac{1} {1 - Slope\;of\;AE\;Curve} = The \;Multiplier

Example: If the slope of the AEAE curve is 0.5, what is the multiplier?

The  Multiplier=11−0.5=2The \;Multiplier = \frac{1} {1 - 0.5} = 2

Note: There are other versions of the formula for the multiplier.

Multiplier=11−MPC,Multiplier=1MPSMultiplier = \frac{1} {1 - MPC}, Multiplier = \frac{1} {MPS}


The Multiplier Applications

Imports and income taxes impacts the size of the multiplier. In fact, they make the multiplier smaller. How?

Recall that the multiplier is:

Multiplier=11−Slope  of  AE  Curve Multiplier = \frac{1} {1- Slope\;of\;AE\;Curve}

Notice that the smaller the slope of AE, the smaller the multiplier is. Using mathematical formulas, we can find that the slope of the AEAE curve is

Slope  of  AE  Curve=b(1−t)−mSlope\; of\; AE\; Curve = b(1-t)-m

Substituting this to our multiplier, we have

Multiplier=Multiplier = 11−[b(1−t)−m] \large \frac{1} {1 - [b(1-t)-m]}

Where:
bb = marginal propensity to consume
tt = marginal tax rate
mm = marginal propensity to import

Therefore, the multiplier can be small if:
  1. bb is small
  2. tt (marginal tax rate) is large
  3. mm (marginal propensity to import) is large

Example: Find the multiplier if b=0.5b=0.5, t=0t=0, m=0m=0. Then find the multiplier when b=0.5b=0.5, t=0.1t=0.1, m=0.2m=0.2. What difference do you see?

Multiplier=Multiplier = 11−[b(1−t)−m]=11−[0.5(1−0)−0]=10.5=2 \large \frac{1} {1 - [b(1-t)-m]} = \frac{1} {1 - [0.5 (1 - 0 ) -0 ] } =\frac{1} {0.5} = 2

Multiplier=Multiplier = 11−[b(1−t)−m]=11−[0.5(1−0)−0]=10.75=1.33‾ \large \frac{1} {1 - [b(1-t)-m]} = \frac{1} {1 - [0.5 (1 - 0 ) -0 ] } =\frac{1} {0.75}= 1.\overline{33}

The multiplier is smaller when there is marginal tax rate and marginal propensity to import.

Note: The smaller the multiplier is from import and income taxes, the less steep the slope of the AE curve is. This reduces the value of the multiplier, which makes the equilibrium expenditure lower.

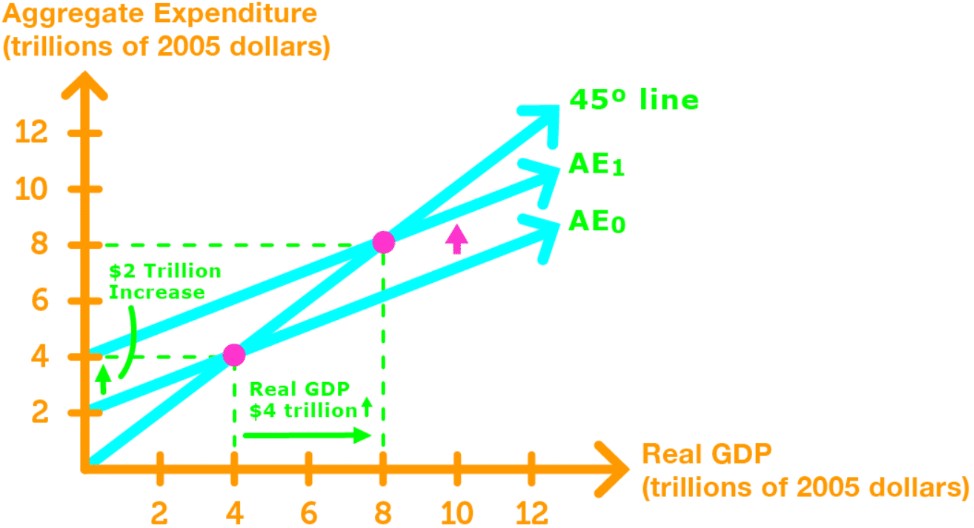
The Multiplier Definitions

The slope of the AEAE curve here is 12\frac{1}{2}, and the multiplier is 2.

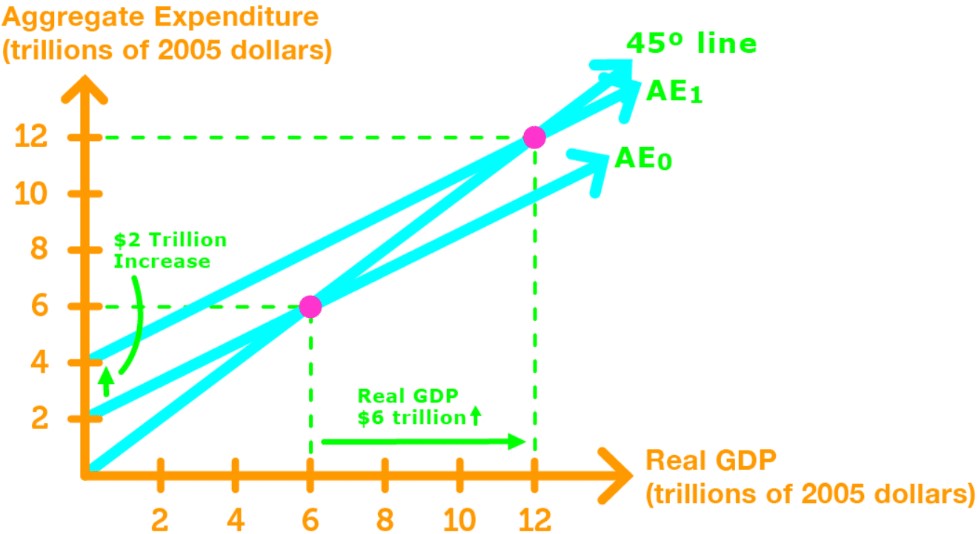
The Multiplier Definitions

The slope of the AEAE curve here is 23\frac{2}{3}, and the multiplier is 3.
Concept

Introduction to the Multiplier Effect in Economics

The multiplier effect is a fundamental concept in economics that explains how an initial change in spending can lead to a larger overall impact on the economy. Our introduction video provides a clear and concise explanation of this crucial economic principle. By watching this video, viewers will gain a solid understanding of how the multiplier works and its significance in economic analysis. The multiplier effect plays a vital role in shaping economic policies and predicting their outcomes. It demonstrates how government spending, investments, or changes in consumer behavior can have amplified effects on economic growth, employment, and overall economic activity. Understanding the multiplier is essential for policymakers, economists, and students alike, as it helps in evaluating the potential impacts of various economic interventions. This knowledge enables more informed decision-making and better forecasting of economic growth trends, making it an indispensable tool in the field of economics.

FAQs

Here are some frequently asked questions about the multiplier effect and related concepts:

  1. What is the multiplier effect in economics?

    The multiplier effect is an economic concept that describes how an initial change in spending can lead to a larger overall impact on the economy. It shows that the total increase in national income can be greater than the initial change in expenditure due to the ripple effect of spending throughout the economy.

  2. How is the multiplier calculated?

    The multiplier is calculated using the formula: Multiplier = 1 / (1 - MPC), where MPC is the Marginal Propensity to Consume. For example, if the MPC is 0.8, the multiplier would be 1 / (1 - 0.8) = 5, meaning a $1 increase in spending could lead to a $5 increase in national income.

  3. How do imports and taxes affect the multiplier?

    Imports and taxes reduce the multiplier effect. The Marginal Propensity to Import (MPI) and the Marginal Tax Rate (MTR) cause "leakages" from the circular flow of income, decreasing the overall impact of initial spending changes. The adjusted formula becomes: Multiplier = 1 / (1 - MPC + MPI + MTR).

  4. What is the relationship between the Aggregate Expenditure (AE) curve and the multiplier?

    The slope of the AE curve is directly related to the multiplier. A steeper AE curve indicates a higher Marginal Propensity to Consume (MPC), which results in a larger multiplier. Conversely, a flatter AE curve suggests a lower MPC and a smaller multiplier effect.

  5. How do policymakers use the multiplier concept in economic decision-making?

    Policymakers use the multiplier concept to estimate the potential impact of fiscal policies such as government spending or tax cuts. Understanding the multiplier helps them design more effective economic stimulus packages during recessions or plan for the broader economic effects of policy changes. However, they must also consider factors like time lags and potential crowding out effects when applying this concept.

Prerequisites

Understanding the foundations of economics is crucial when delving into more advanced concepts like "The multiplier definitions." One of the key prerequisite topics that plays a significant role in grasping the multiplier effect is market equilibrium. This fundamental concept serves as a cornerstone for comprehending how changes in various economic factors can have amplified effects on the overall economy.

Market equilibrium, which refers to the state where supply and demand are balanced, is essential for understanding the multiplier effect. When we talk about the multiplier definitions, we're essentially exploring how initial changes in spending can lead to larger changes in economic output. This relationship is intrinsically linked to the concept of equilibrium in the goods market.

To fully appreciate the multiplier effect, one must first grasp how markets reach equilibrium. In a state of market equilibrium, the quantity of goods supplied matches the quantity demanded at a specific price point. This balance is crucial because it sets the stage for understanding how external factors can disrupt this equilibrium and trigger a chain reaction of economic events.

The multiplier definitions build upon this foundation by explaining how an initial change in spending can lead to a more significant change in national income. For instance, when government spending increases, it doesn't just affect the immediate recipients of that spending. Instead, it creates a ripple effect throughout the economy, as those recipients then increase their own spending, and so on. This cascading effect is what we refer to as the multiplier.

By understanding market equilibrium, students can better visualize how this initial disruption to the equilibrium state can propagate through the economy. It helps in comprehending why a small change in one sector can lead to much larger changes in overall economic output. This relationship between initial change and final impact is at the heart of the multiplier definitions.

Moreover, the concept of equilibrium in the goods market is crucial for grasping how the multiplier effect can vary in different economic conditions. In a perfectly competitive market at equilibrium, the multiplier effect might work differently compared to a market with imperfections or one that is not at equilibrium. This understanding allows for a more nuanced analysis of economic policies and their potential impacts.

In conclusion, mastering the prerequisite topic of market equilibrium is essential for students aiming to fully comprehend the multiplier definitions. It provides the necessary context for understanding how economic changes propagate and amplify, forming the basis for more complex economic analyses and policy decisions. By building on this foundational knowledge, students can develop a more comprehensive understanding of macroeconomic principles and their real-world applications.