In an economic sense, firms in an oligopoly market play a game with each other to try to achieve economic profit.
Lets first try to investigate the features of a game, and then later see how it relates to Prisoners Dilemma and Duopoly.
There four common features in a game:
- Rules: laws that need to be followed.
- Strategies: possible actions for a player.
- Payoffs: the gains and loss of a player from his actions.
- Outcomes: the result gained from the action of all players.
Rules of Prisoners Dilemma, and Strategies
Background: Suppose two people are suspects of a crime, and now must become prisoners. We will call them prisoners A and B. The police do not know who committed the crime, so they force the prisoners to play a game to see if they stay silent or betray each other.
Note: Assume that the prisoners are not friends, and do not know each other.
Rule: Each prisoner is placed in an isolated room, and the prisoners cannot communicate with each other. Each prisoner is told that they are suspected of committing the crime, and are told the following
- If both do not accuse each other of committing the crime and stay silent, then both will be sentenced to jail for 3 years.
- If the prisoner accuses the other prisoner of committing the crime and the other stays silent, then the accuser is free, and the other prisoner is sentenced to jail for 10 years.
- If both prisoners accuse each other, then both will be sentenced to jail for 5 years.
Strategies: Prisoner A and B have two possible actions
- Stay silent to the crime.
- Accuse the other prisoner.
Outcomes: Since there are two prisoners and each have two strategies
- Both stay silent
- Prisoner A accuses, Prisoner B stays silent
- Prisoner A stays silent, Prisoner B accuses
- Both prisoners accuse each other
The Payoff Matrix, & Nash Equilibrium
A payoff matrix is a table that shows all the strategies each player can make, and lists the gains/losses of each player for every outcome.
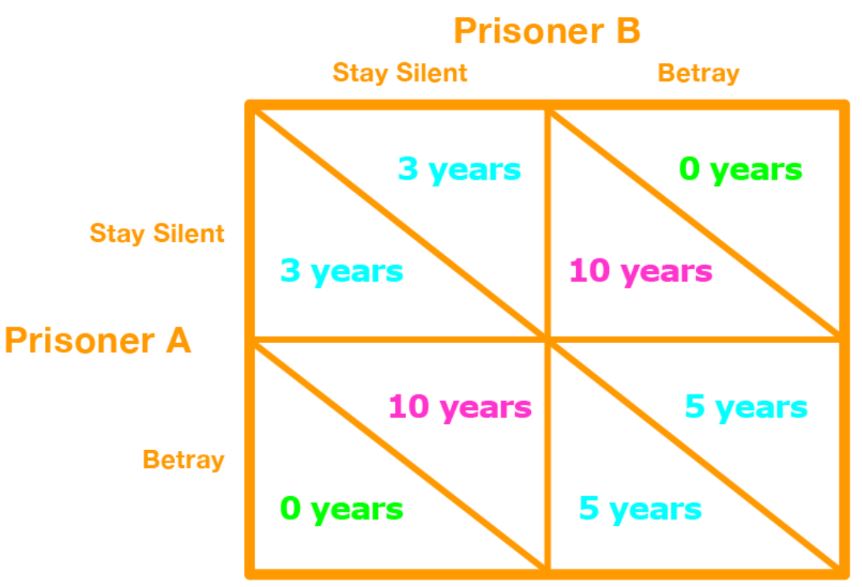
Nash Equilibrium: is the equilibrium where each players strategy is optimal when given the strategies of all other players.
Lets look at the optimal strategy for prisoner A.
- Case 1: Prisoner A assumes Prisoner B stays silent about the crime.
If prisoner A chooses.
1. Stay silent → 3 years in jail
2. Accuses → 0 years in jail
The best choice is to accuse prisoner B.
Case 2: Prisoner A assumes Prisoner B accuses.
- If prisoner A chooses.
1. Stay silent → 10 years in jail
2. Accuses → 5 years in jail
The best choice is to accuse prisoner B.
So, whether prisoner B stays silent or accuses, prisoner As best action is to accuse.
Lets look at the optimal strategy for prisoner B.
- Case 1: Prisoner B assumes Prisoner A stays silent about the crime.
If prisoner B chooses.
1. Stay silent → 3 years in jail
2. Accuses → 0 years in jail
The best choice is to accuse prisoner A.
Case 2: Prisoner B assumes Prisoner A accuses.
- If prisoner B chooses.
1. Stay silent → 10 years in jail
2. Accuses → 5 years in jail
The best choice is to accuse prisoner A.
So, whether prisoner A stays silent or accuses, prisoner Bs best action is to accuse.
Hence, the Nash equilibrium is for both prisoners accuse each other. This outcome will lead both prisoners to go to jail for 5 years.
Prisoners Dilemma in Duopoly
The same idea in prisoners dilemma holds for duopoly.
Collusive Agreement: an agreement between two firms to form a cartel and act as a monopoly.
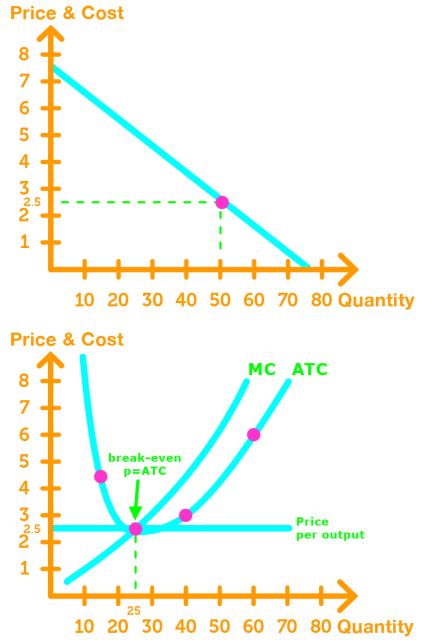
Suppose there is firm A and firm B, and the demand and costs for the product is below.
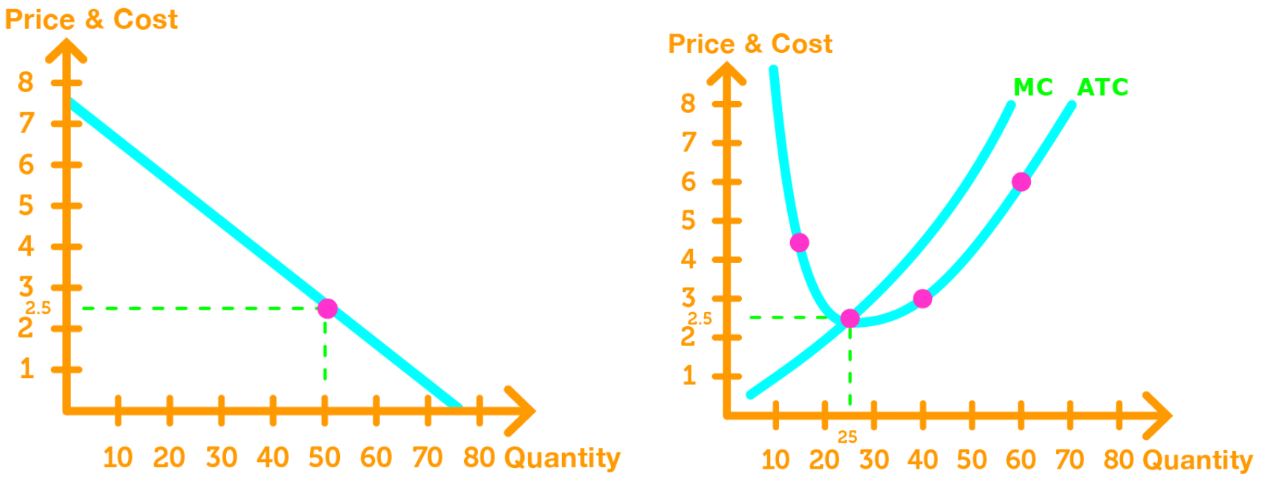
Strategies: Each firm can either
- Comply
- Cheat .
Outcomes:
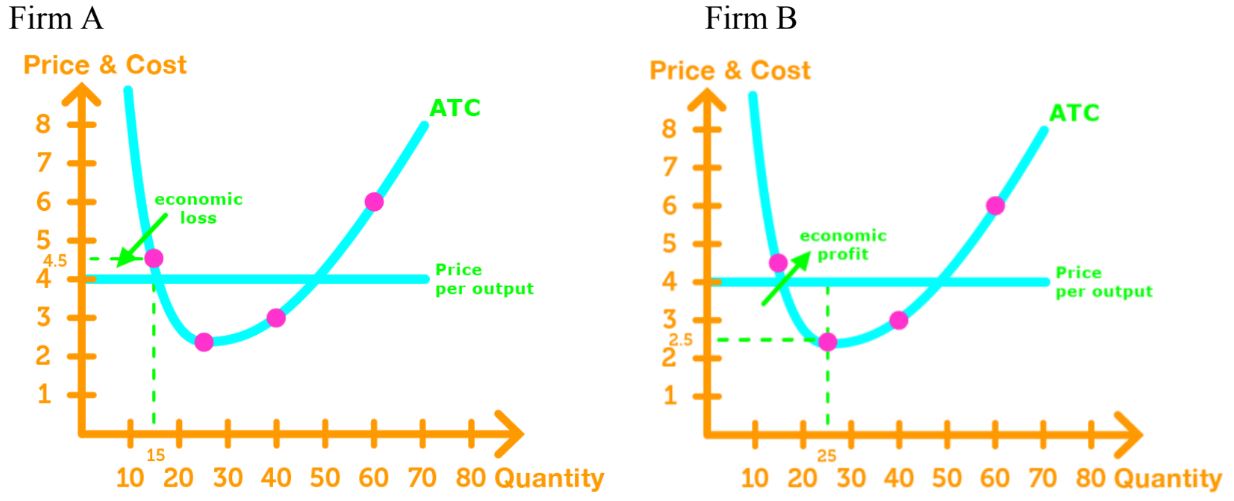
- Both firms Comply: then they enter a collusive agreement and act as a monopoly. Both firms produce an output of 15, and sell each outprice for $5.
- Firm A Complies, and Firm B Cheats: Then firm B produces 10 more than firm A, thus lowering the price to $4.
- Firm B Complies, and Firm A Cheats: Vice versa happens in outcome b.
- Both Firms Cheat: They break the collusive agreement and both firms will increase their output to 25, thus lowering the price to $2.50. Both firms will gain no profit.
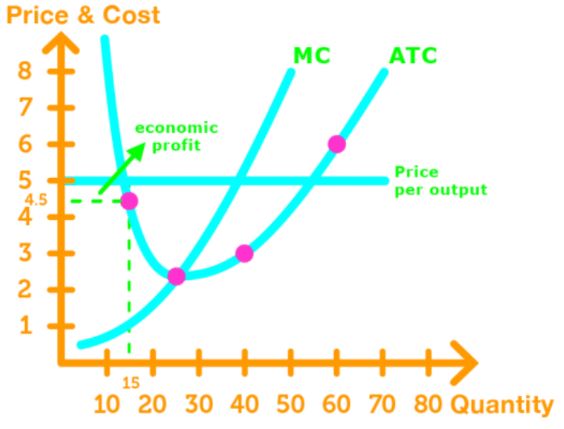
Result: Both firms gain an economic profit of $7.50
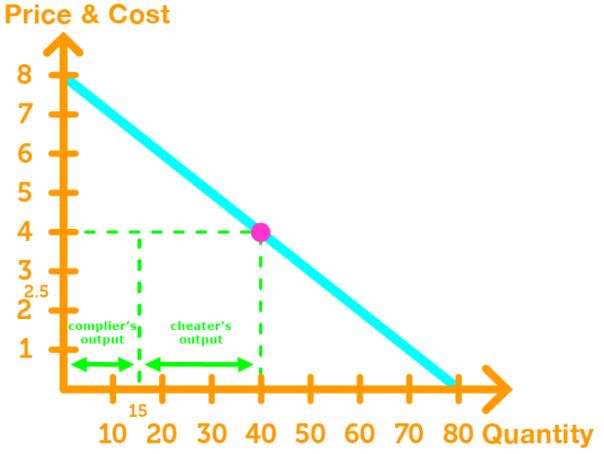
Result: Firm B gains an economic profit of $37.50, and Firm A incurs an economic loss of -$7.50.
Payoff: With the listed outcomes, we can create a payoff matrix.
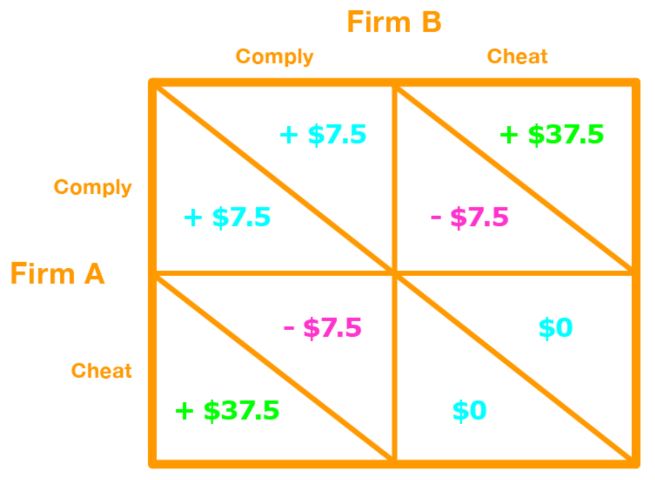
The Nash equilibrium will be for both firms to cheat.






