1. Home
  2. >Chemistry
  3. >Acid-Base Theory
Help

Buffer solutions

Get the most by viewing this topic in your current grade. Pick your course now.

?
Intros
Lessons
  1. What is a buffer solution?
  2. Definition and uses.
  3. Adding acid to buffer solution: pH change
  4. Adding base to buffer solution: pH change
  5. Worked example of pH change to buffer.
  6. Buffers and change in concentration.
  7. The buffer region of a titration curve.
?
Examples
Lessons
  1. Calculate the pH change that takes place when acid or base is added to buffer solutions.
    A 1L buffer solution is made from 0.4 mol ethanoic acid (CH3COOH) and 0.4 mol of its conjugate base, ethanoate (CH3COO-). The Ka of ethanoic acid is 1.4*10-5.
    What would be the pH change if 0.1 mol of H3O+ was added to this buffer?
    1. Show the effect of a buffer using a titration curve and find Ka of a weak acid in a buffer.
      Below is the titration curve of a weak acid by a strong base:
      1. At which point in the titration is a buffer effect occurring? Mark the region on the graph.
      2. pH1/2 during this titration was 3.82. Find the Ka of the weak acid in the buffer using the Henderson-Hasselbach equation.
        1. Calculate the concentration of solutions needed to prepare a buffer of a given pH.
          Ethanoic acid has a Ka of 1.4*10-5. What amounts are required to prepare a 100mL buffer with pH 4.25 using ethanoic acid, CH3COOH and sodium hydroxide, NaOH?

          1 Source for acidity constant (Ka) data: ATKINS, P. W., & DE PAULA, J. (2006). Atkins' Physical chemistry. Oxford, Oxford University Press.
        Topic Notes
        ?

        Introduction to Buffer Solutions

        Buffer solutions are essential in chemistry, playing a crucial role in maintaining stable pH levels in various systems. These remarkable mixtures resist changes in pH when small amounts of acids or bases are added, making them indispensable in many chemical and biological processes. Our introduction video provides a visual overview of buffer solutions and their significance. In this article, we'll delve deeper into what makes a buffer, exploring the key components that give these solutions their unique properties. We'll also examine what a buffer does, uncovering the mechanisms behind their ability to maintain pH stability. Additionally, we'll discuss the wide-ranging applications of buffer solutions in fields such as biochemistry, pharmaceuticals, and environmental science. Understanding buffer solutions is fundamental for anyone studying chemistry or working in related industries, as these powerful tools help control and optimize countless chemical reactions and processes.

        What is a Buffer Solution?

        Buffer solutions are essential mixtures in chemistry that help maintain a stable pH level when small amounts of acids or bases are added. These solutions are crucial in various biological and chemical processes where pH stability is paramount. But what is a buffer made of, and why are they so important?

        A buffer solution is typically composed of two key components: a weak acid or base and its conjugate pair. The weak acid or base acts as the primary buffering agent, while its salt (the conjugate) provides the counterbalance. This unique composition allows buffers to resist significant changes in pH when small amounts of strong acids or bases are introduced.

        The composition of buffers is carefully balanced to maintain pH stability. For an acidic buffer, you'll find a weak acid and its conjugate base. Conversely, a basic buffer contains a weak base and conjugate acid. This pairing is crucial for the buffer's ability to neutralize both acids and bases effectively.

        Let's explore some common buffer solution examples to better understand their composition and function:

        • Acetic acid/sodium acetate buffer: This is a classic example of an acidic buffer. It consists of acetic acid (CH3COOH) and its salt, sodium acetate (CH3COONa).
        • Carbonic acid/bicarbonate buffer: Found in blood, this buffer helps maintain the body's pH. It's made up of carbonic acid (H2CO3) and bicarbonate ions (HCO3-).
        • Ammonia/ammonium chloride buffer: This basic buffer comprises ammonia (NH3) and its salt, ammonium chloride (NH4Cl).
        • Phosphate buffer: Widely used in biological research, this buffer contains dihydrogen phosphate (H2PO4-) and monohydrogen phosphate (HPO4^2-).

        These buffered solution examples demonstrate the versatility of buffers in various applications, from laboratory experiments to natural biological systems.

        The importance of buffers in maintaining pH stability cannot be overstated. They play crucial roles in:

        1. Biological systems: Our blood, for instance, uses the carbonic acid/bicarbonate buffer to keep pH within a narrow range, essential for proper enzyme function and overall health.
        2. Industrial processes: Many manufacturing processes require specific pH levels for optimal results, and buffers help maintain these conditions.
        3. Environmental science: Natural water bodies use carbonate buffers to resist pH changes, protecting aquatic life from sudden acidification.
        4. Laboratory work: Buffers are indispensable in creating stable environments for chemical reactions and analyses.

        Understanding what makes up a buffer is key to appreciating their function. The weak acid or base component can donate or accept protons, while the conjugate pair acts as a reservoir, ready to neutralize incoming acids or bases. This dynamic interplay allows buffers to maintain pH stability even when challenged by additional acids or bases.

        In conclusion, buffer solutions are remarkable mixtures that play a vital role in countless chemical and biological processes. Their unique composition of weak acids or bases and their conjugate pairs allows them to maintain pH stability in the face of chemical challenges. From the human body to industrial applications, buffers are the unsung heroes of pH regulation, ensuring that critical processes can occur within optimal pH ranges.

        How Do Buffers Work?

        Buffers are remarkable chemical systems that play a crucial role in maintaining stable pH levels in various biological and chemical processes. Understanding how buffers resist changes in pH is essential for many scientific applications. This article will explore the mechanism behind buffer action, discuss Le Chatelier's principle in relation to buffers, and provide examples of how buffers respond to acid and base additions.

        At its core, a buffer is a solution that contains a weak acid and its conjugate base, or a weak base and conjugate acid. This unique composition allows buffers to resist changes in pH when small amounts of acids or bases are added. The key to understanding how buffers work lies in their ability to neutralize both acids and bases through a series of chemical reactions.

        When a small amount of acid is added to a buffer solution, the conjugate base component of the buffer reacts with the incoming hydrogen ions (H+), effectively neutralizing them. Conversely, when a small amount of base is added, the weak acid component of the buffer donates protons to neutralize the hydroxide ions (OH-). This dual action is what gives buffers their remarkable ability to maintain a relatively constant pH.

        Le Chatelier's principle plays a significant role in understanding buffer behavior. This principle states that when a system at equilibrium is disturbed, it will shift to counteract the change and establish a new equilibrium. In the context of buffers, this means that when an acid or base is added, the buffer system will shift its equilibrium to minimize the change in pH.

        Let's consider a specific example to illustrate how buffers resist pH change. Take an acetic acid-acetate buffer system, represented by the following equation:

        CH3COOH CH3COO- + H+

        When a small amount of acid (H+) is added to this buffer, the equilibrium shifts to the left according to Le Chatelier's principle. The acetate ions (CH3COO-) react with the added H+ ions to form more acetic acid (CH3COOH). This reaction consumes the excess H+ ions, preventing a significant drop in pH.

        Conversely, if a small amount of base (OH-) is added, the acetic acid component of the buffer reacts with the OH- ions to produce water and acetate ions. This reaction can be represented as:

        CH3COOH + OH- CH3COO- + H2O

        This process consumes the excess OH- ions, preventing a significant increase in pH. The buffer equations demonstrate how the system responds to both acidic and basic disturbances, maintaining a relatively stable pH.

        The significance of buffer equations extends beyond their ability to show chemical reactions. These equations allow scientists to calculate the pH of buffer solutions using the Henderson-Hasselbalch equation:

        pH = pKa + log([A-] / [HA])

        Where pKa is the negative logarithm of the acid dissociation constant, [A-] is the concentration of the conjugate base, and [HA] is the concentration of the weak acid. This equation is crucial for preparing buffers with specific pH values and understanding how the ratio of acid to conjugate base affects the buffer's pH.

        It's important to note that while buffers are effective at resisting pH changes, they have limitations. Buffers have a finite capacity to neutralize acids or bases. Once this capacity is exceeded, the buffer becomes overwhelmed, and significant pH changes can occur. This concept is known as buffer capacity and is an essential consideration when working with buffer systems.

        In biological systems, buffers play a vital role in maintaining the pH of blood and other bodily fluids within narrow ranges. For example, the bicarbonate buffer system in blood helps maintain a pH between 7.35 and 7.45, which is crucial for proper enzyme function.

        Buffer Capacity and pH Calculations

        Buffer capacity is a crucial concept in chemistry that refers to the ability of a buffer solution to resist changes in pH when small amounts of acid or base are added. A buffer solution consists of a weak acid and its conjugate base or a weak base and its conjugate acid. The buffer capacity is essential in maintaining a stable pH in various biological and chemical systems.

        To calculate the pH of a buffer solution, chemists use the Henderson-Hasselbalch equation. This equation relates the pH of a buffer solution to the pKa of the weak acid and the concentrations of the acid and its conjugate base. The Henderson-Hasselbalch equation is expressed as:

        pH = pKa + log([A-] / [HA])

        Where:

        • pH is the negative logarithm of the hydrogen ion concentration
        • pKa is the negative logarithm of the acid dissociation constant
        • [A-] is the concentration of the conjugate base
        • [HA] is the concentration of the weak acid

        Let's walk through some buffer equation examples to illustrate how to use the Henderson-Hasselbalch equation:

        Example 1: Calculate the pH of a buffer solution containing 0.1 M acetic acid (CH3COOH) and 0.1 M sodium acetate (CH3COONa). The pKa of acetic acid is 4.76.

        Step 1: Identify the components of the equation

        • pKa = 4.76
        • [A-] = [CH3COO-] = 0.1 M
        • [HA] = [CH3COOH] = 0.1 M

        Step 2: Apply the Henderson-Hasselbalch equation

        pH = 4.76 + log(0.1 / 0.1) = 4.76 + log(1) = 4.76

        Example 2: Calculate the pH of a buffer solution containing 0.2 M ammonia (NH3) and 0.1 M ammonium chloride (NH4Cl). The pKa of ammonium ion is 9.25.

        Step 1: Identify the components of the equation

        • pKa = 9.25
        • [A-] = [NH3] = 0.2 M
        • [HA] = [NH4+] = 0.1 M

        Step 2: Apply the Henderson-Hasselbalch equation

        pH = 9.25 + log(0.2 / 0.1) = 9.25 + log(2) = 9.55

        While buffer systems are incredibly useful in maintaining stable pH levels, they do have limitations:

        1. Limited range: Buffers are most effective within ±1 pH unit of their pKa value.
        2. Finite capacity: Buffers can only neutralize a limited amount of added acid or base before becoming overwhelmed.
        3. Temperature sensitivity: The pKa of weak acids and bases can change with temperature, affecting buffer performance.
        4. Ionic strength effects: High ionic strength can alter the activity coefficients of species in solution, impacting buffer behavior.
        5. Dilution effects: Diluting a buffer solution can change its pH and reduce its buffering capacity.

        Understanding buffer capacity and how to calculate the pH of buffer solutions using the Henderson-Hasselbalch equation is essential for many applications in chemistry, biochemistry, and environmental science. By mastering these concepts and practicing buffer equation examples, researchers and students can better predict

        Applications of Buffer Solutions

        Buffer solutions play a crucial role in various fields of science and industry, maintaining stable pH levels in diverse environments. These solutions resist changes in pH when small amounts of acids or bases are added, making them invaluable in chemistry, biology, and industrial processes. Understanding the applications of buffer solutions is essential for researchers, healthcare professionals, and industrial chemists alike.

        In chemistry laboratories, buffer solutions are indispensable for maintaining consistent pH levels during experiments and analyses. They are used in techniques such as spectrophotometry, chromatography, and electrophoresis to ensure accurate results. For instance, in gel electrophoresis, a common method for separating DNA fragments, buffers maintain a stable pH to prevent damage to the DNA molecules and ensure proper migration through the gel.

        Biological buffers are of paramount importance in living systems. The human body, for example, relies on several buffer systems to maintain a narrow pH range essential for survival. Blood pH regulation is a prime example of biological buffers in action. The bicarbonate buffer system, consisting of carbonic acid and bicarbonate ions, works tirelessly to keep blood pH within the critical range of 7.35 to 7.45. This tight regulation is vital for proper enzyme function, oxygen transport, and overall cellular health.

        In addition to blood, other biological fluids such as saliva and urine also contain buffer systems. These buffers help protect teeth from acid erosion and maintain optimal conditions for digestive processes. The importance of buffers extends to cellular biology, where intracellular pH regulation is crucial for proper protein folding, enzyme activity, and cellular metabolism.

        Industrial applications of buffer solutions are diverse and widespread. In the food and beverage industry, buffers are used to control the pH of products, ensuring consistency in taste, texture, and shelf life. For example, citrate buffers are commonly used in soft drinks to balance acidity and enhance flavor. In the pharmaceutical industry, buffers play a critical role in drug formulation and stability. They help maintain the pH of medications, which is essential for drug efficacy and safety.

        Water treatment facilities utilize buffer solutions to adjust and stabilize the pH of drinking water and wastewater. This process is crucial for preventing corrosion in pipes and ensuring the effectiveness of disinfection processes. In agriculture, buffer solutions are used in soil analysis and treatment, helping to optimize soil pH for crop growth and nutrient availability.

        The cosmetics industry also relies heavily on buffer solutions. Many personal care products, such as shampoos, lotions, and creams, incorporate buffers to maintain a pH that is compatible with skin and hair. This not only ensures product effectiveness but also helps prevent irritation and maintain skin health.

        In biotechnology and molecular biology, buffer solutions are essential for various laboratory techniques. PCR (Polymerase Chain Reaction), a method used to amplify DNA, requires specific buffer conditions to function optimally. Similarly, buffers are crucial in protein purification processes, enzyme assays, and cell culture media, where maintaining a stable pH is vital for biological activity and cell viability.

        The development of new buffer solutions continues to be an active area of research, particularly in the field of biological buffers. Scientists are constantly seeking to create buffers that mimic physiological conditions more closely, leading to improved in vitro studies and more accurate drug testing models.

        In conclusion, buffer solutions are indispensable in chemistry, biology, and industry. Their ability to maintain stable pH levels makes them crucial for a wide range of applications, from regulating blood pH in living organisms to ensuring product quality in manufacturing processes. As our understanding of pH-dependent processes grows, the importance and applications of buffer solutions are likely to expand further, continuing to play a vital role in scientific research and industrial innovation.

        Buffer Solutions in Titrations

        Buffer regions play a crucial role in acid-base titrations, forming an essential part of the titration process. These regions are characterized by their ability to resist significant changes in pH when small amounts of acid or base are added. Understanding how buffer regions form and their significance is key to mastering acid-base chemistry.

        During the titration of a weak acid with a strong base, or a weak base with a strong acid, a buffer region forms around the midpoint of the titration. This occurs because, at this stage, there are roughly equal concentrations of the weak acid (or base) and its conjugate base (or acid) present in the solution. The presence of both species allows the solution to neutralize small additions of either H+ or OH- ions, maintaining a relatively stable pH.

        A critical point in the titration process is the half-equivalence point. This occurs when exactly half of the weak acid or base has been neutralized. The half-equivalence point is significant for several reasons:

        • At this point, the concentration of the weak acid or base is equal to the concentration of its conjugate.
        • The pH at the half-equivalence point is numerically equal to the pKa of the weak acid (or pKb of the weak base).
        • The buffer capacity is at its maximum, providing the greatest resistance to pH changes.

        Understanding the half-equivalence point is crucial for determining the pKa of a weak acid using titration data. To find the pKa, one can simply read the pH at the half-equivalence point directly from the titration curve. Alternatively, if given the volume of base added at the equivalence point, one can calculate the volume at the half-equivalence point (which is half of this value) and then find the corresponding pH.

        The HCOO- ion (formate ion) serves as an excellent example to illustrate the concept of conjugate bases in buffer solutions. HCOO- is the conjugate base of formic acid (HCOOH). In answer to the question "is HCOO- an acid or base?", it's important to note that HCOO- can act as both, depending on the context:

        • As a conjugate base, HCOO- can accept a proton, acting as a base.
        • In water, HCOO- can also act as a weak base, accepting a proton from H2O to form HCOOH and OH-.

        In a buffer solution containing HCOOH and HCOO-, the formate ion plays a crucial role in maintaining pH stability. When a small amount of strong acid is added, HCOO- can accept protons, converting to HCOOH and minimizing the pH change. Conversely, when a small amount of strong base is added, HCOOH can donate protons, forming HCOO- and again resisting significant pH changes.

        The buffer region in a titration involving HCOOH and HCOO- would be most effective when the concentrations of HCOOH and HCOO- are approximately equal, which occurs at the half-equivalence point. At this point, the pH of the solution would be equal to the pKa of formic acid (approximately 3.75).

        Understanding buffer regions, half-equivalence points, and the behavior of conjugate acid-base pairs like HCOOH/HCOO- is essential for various applications in chemistry, biochemistry, and environmental science. These concepts are fundamental in maintaining pH stability in biological systems, optimizing industrial processes, and analyzing environmental samples. By mastering these principles, one can effectively predict and control pH changes in various chemical and biological systems, making this knowledge invaluable in both theoretical and practical applications of acid-base chemistry.

        Conclusion

        Buffer solutions play a crucial role in maintaining pH stability in various chemical and biological systems. These solutions consist of a weak acid and its conjugate base, or a weak base and its conjugate acid, working together to resist changes in pH when small amounts of acids or bases are added. Understanding buffer solutions is essential in chemistry, biochemistry, and environmental science. They maintain optimal conditions for enzymatic reactions, preserve the integrity of biological samples, and regulate pH in natural water systems. The ability of buffer solutions to maintain chemical equilibrium is vital in many industrial processes and laboratory experiments. By reviewing the introduction video, you'll gain a visual understanding of how buffer solutions function and their importance in various applications. Mastering the concepts of buffer solutions will enhance your comprehension of chemical equilibria and pH regulation, providing a solid foundation for advanced studies in chemistry and related fields.

        Buffer Solutions: Definition and Uses

        What is a buffer solution? Definition and uses.

        Step 1: Introduction to Buffer Solutions

        Buffer solutions are a fundamental concept in chemistry, particularly in the study of acids and bases. They play a crucial role in maintaining the pH levels in various chemical and biological systems. Understanding what a buffer solution is and how it functions is essential for anyone studying chemistry or related fields.

        Step 2: Definition of a Buffer Solution

        A buffer solution is defined as a solution of a weak acid or base in equilibrium with its conjugate pair. This equilibrium allows the solution to resist changes in pH when small amounts of strong acid or base are added. The key components of a buffer solution are the weak acid or base and its conjugate pair, both of which must be present in substantial quantities to effectively resist pH changes.

        Step 3: How Buffer Solutions Work

        The ability of a buffer solution to resist pH changes is due to the equilibrium between the weak acid or base and its conjugate pair. When a strong acid or base is added to the buffer solution, the equilibrium shifts to counteract the change in pH. This process is known as buffering. For example, if a strong acid is added, the conjugate base will react with the added hydrogen ions to form the weak acid, thereby minimizing the change in pH.

        Step 4: Components of a Buffer Solution

        To create a buffer solution, you need a weak acid or base and a large amount of its conjugate pair. For instance, a common buffer solution might consist of methanoic acid (HCOOH) and its conjugate base, methanoate (HCOO-). These components are typically mixed in equal quantities to ensure effective buffering. The conjugate base is often added in the form of a salt, such as sodium methanoate (NaHCOO), which dissociates in water to provide the conjugate base.

        Step 5: Example of a Buffer Solution

        An example of a buffer solution is one made from methanoic acid (HCOOH) and sodium methanoate (NaHCOO). When dissolved in water, sodium methanoate dissociates to form methanoate ions (HCOO-), which then participate in the buffering action. This buffer solution can resist changes in pH when small amounts of strong acid or base are added, making it useful in various chemical and biological applications.

        Step 6: Uses of Buffer Solutions

        Buffer solutions have a wide range of applications. They are used in biological systems to maintain the pH of blood and other bodily fluids. In industrial processes, buffer solutions are used to control the pH of chemical reactions. They are also essential in laboratory settings for experiments that require a stable pH environment. Additionally, buffer solutions are used in pharmaceuticals to ensure the stability and efficacy of medications.

        Step 7: Importance of Buffer Solutions

        The importance of buffer solutions cannot be overstated. They are crucial for maintaining the proper functioning of biological systems, ensuring the accuracy of chemical reactions, and providing stability in various industrial and laboratory processes. Without buffer solutions, many chemical and biological processes would be disrupted by changes in pH, leading to potentially harmful consequences.

        Step 8: Conclusion

        In conclusion, buffer solutions are essential for maintaining stable pH levels in various chemical and biological systems. They consist of a weak acid or base and its conjugate pair, which work together to resist changes in pH. Buffer solutions have numerous applications, from biological systems to industrial processes, making them a vital component in many fields of study and practice.

        FAQs

        Here are some frequently asked questions about buffer solutions:

        1. What makes a buffer solution?

        A buffer solution is made of a weak acid and its conjugate base, or a weak base and its conjugate acid. For example, an acetic acid-acetate buffer contains both acetic acid (CH3COOH) and sodium acetate (CH3COONa).

        2. Why do buffers resist pH change?

        Buffers resist pH change because they contain both an acid and a base component. When a small amount of acid is added, the base component neutralizes it. Similarly, when a small amount of base is added, the acid component neutralizes it, maintaining a relatively stable pH.

        3. What is the main purpose of a buffer?

        The main purpose of a buffer is to maintain a stable pH in a solution when small amounts of acids or bases are added. This is crucial in many biological and chemical processes where pH stability is essential for optimal function.

        4. How do buffers maintain pH in blood?

        Blood contains several buffer systems, with the bicarbonate buffer being the most important. It consists of carbonic acid (H2CO3) and bicarbonate ions (HCO3-). This system can neutralize both acids and bases to keep blood pH within the narrow range of 7.35 to 7.45.

        5. What is an example of a buffer solution in real life?

        A common real-life example of a buffer solution is human blood. Other examples include saliva, which helps protect teeth from acid erosion, and many commercial products like shampoos and pharmaceuticals that require stable pH for effectiveness and safety.

        Prerequisite Topics

        Understanding buffer solutions is a crucial concept in chemistry, but to fully grasp its intricacies, it's essential to have a solid foundation in certain prerequisite topics. One of the most important prerequisites for comprehending buffer solutions is the acid dissociation constant. This fundamental concept plays a pivotal role in understanding how buffer solutions function and maintain their pH stability.

        The acid dissociation constant, often denoted as Ka, is a quantitative measure of the strength of an acid in solution. It represents the extent to which an acid dissociates into its constituent ions in water. This concept is crucial for buffer solutions because it directly relates to the equilibrium between the weak acid or base and its conjugate pair in the buffer system.

        When studying buffer solutions, you'll find that the acid dissociation constant is used to calculate the pH of the buffer and predict its behavior when small amounts of acids or bases are added. The relationship between the acid dissociation constant and the buffer capacity is particularly important, as it determines how effectively the buffer can resist changes in pH.

        Moreover, understanding the acid dissociation constant helps in selecting appropriate weak acids or bases for creating buffer solutions. The pKa value, which is derived from the acid dissociation constant, is used to determine the optimal pH range in which a particular buffer system will be most effective.

        In the context of buffer solutions, the acid dissociation constant also plays a role in explaining why certain combinations of weak acids or bases and their conjugate pairs make effective buffers. It helps in understanding the equilibrium shifts that occur when small amounts of strong acids or bases are added to the buffer solution.

        Furthermore, the concept of the acid dissociation constant is essential for deriving and understanding the Henderson-Hasselbalch equation, which is a fundamental tool for calculating the pH of buffer solutions. This equation directly incorporates the pKa value and the concentrations of the acid-base conjugate pair, demonstrating the intimate connection between the acid dissociation constant and buffer behavior.

        In conclusion, a thorough understanding of the acid dissociation constant is indispensable for mastering the concept of buffer solutions. It provides the necessary foundation for comprehending the chemical equilibria involved, predicting buffer behavior, and applying this knowledge to real-world applications in chemistry and biochemistry. By grasping this prerequisite topic, students will be better equipped to tackle the complexities of buffer solutions and their vital role in maintaining pH stability in various chemical and biological systems.

        In this lesson, we will learn:

        • To recall the definition of a buffer solution and its components.
        • How to predict the shift in equilibrium when acid or base is added to a buffer solution.
        • How to calculate the pH of a buffer after the addition of strong acid or base.
        • How to calculate the Ka of a weak acid from the buffer region of a titration.
        • How to find the quantities needed to make buffer solutions of a given pH.

        Notes:

        • A buffer solution is a solution of a weak acid or base with its conjugate pair that can resist changes in pH when a small amount of strong acid or base is added.
          Buffer solutions are made by mixing a weak acid or base with a large amount of its conjugate pair, even so that there is equal amounts of both.

        • Above all, in chemistry buffers are known as mixtures that resist chemical changes made to them. Outside of chemistry, a buffer is something that acts a barrier or shield to another action or object, like buffers on a train track that cushion trains against their own movement, making them stop when they arrive at a terminus station. In chemistry, a buffer solution cushions and halts a chemical change being made in a few ways.

        • Buffers resist changes in pH by keeping H3O+ concentration nearly constant; it’s able to do this as the solution contains the conjugate acid/base AND its pair in equilibrium. This is seen with weak acids and weak bases.
          • For example, a buffer solution of methanoic acid (HCOOH) and methanoate (HCOO-), its conjugate base. There is an equal amount of conjugate acid and base.
            The weak acid is in equilibrium with its conjugate base (methanoate, HCOO-) in the equation:

            H2O + HCOOH(aq) ⇌\rightleftharpoons HCOO-(aq) + H3O+(aq)

            • If a strong acid such as nitric acid is added, the extra H3O+ ions it produces will react with the methanoate (HCOO-) and produce more HCOOH – the reverse reaction above will occur.

              HCOO-(aq) + H3O+(aq) → H2O + HCOOH(aq)

              This removes the H3O+ that was originally added and returns the pH to near its original value.
            • If a strong base such as sodium hydroxide is added, the extra OH- ions will react with some H3O+ ions in solution to produce water.

              OH-(aq) + H3O+(aq) → 2H2O(l)


              This excess water will shift the equilibrium to the right, producing more HCOO- and H3O+ - the forward reaction in the equilibrium, which will return the pH back to near its original value.

              H2O + HCOOH(aq) → HCOO-(aq) + H3O+(aq)


            Because it’s an equilibrium, we give this an equilibrium constant expression. This is just an acid dissociating into its conjugate base so it is a Ka expression:

            Ka=[HCOO−][H3O+][HCOOH] K_a = \frac{[HCOO^-][H_3O^+]}{[HCOOH]}
        • WORKED EXAMPLE:
          • In a buffer made of 0.5 mol methanoic acid (HCOOH) and 0.5 mol methanoate (HCOO-) in 1L water, 0.1 mol H3O+ is added.
            Look at the equilibrium again:

            H2O + HCOOH(aq) ⇌\rightleftharpoons HCOO-(aq) + H3O+(aq)


            With 0.1 mol H3O+ added, 0.1 mol HCOO- will react with this ‘disturbance’ to the equilibrium, which shifts to the left to oppose the change. This changes the equilibrium amounts of moles:

            Moles of acid (HCOOH)

            Moles of base (HCOO-)

            Before

            0.5

            0.5

            Change

            +0.1

            -0.1

            After

            0.6

            0.4



            This change to the acid and base quantities will affect [H3O+] in this buffer solution. When a buffer has equal amounts of conjugate acid and base, the terms cancel out and [H3O+] will be equal to Ka, which makes pH = pKa: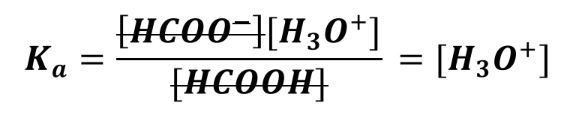
            buffer solutions weak acid or base ph = pka

            When the amounts are unequal, a constant will be in front of [H3O+].
            In our example, after 0.1 mol of acid was added:

            ′After′ Ka=[HCOO−][H3O+][HCOOH]=0.40.6[H3O+]'After' \,K_a = \frac{[HCOO^-][H_3O^+]}{[HCOOH]} = \frac{0.4}{0.6} [H_3O^+]


            With these expressions, we can easily work out [H3O+] and then the pH of the buffer before and after the acid was added, using the Ka of the acid.1
            With Ka (HCOOH) = 1.8*10-4, we work out the pH before the acid was added: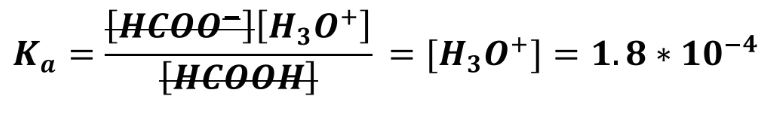

            buffer solutions weak acid or base ph = pka

            ′Before′ pH=−log[1.8∗10−4]=3.74'Before' \, pH = -log[1.8* 10^{-4}] = 3.74

            We re-arrange the Ka expression to solve for [H3O+] after the acid is added:

            Ka=[HCOO−][H3O+][HCOOH] K_a = \frac{[HCOO^-][H_3O^+]}{[HCOOH]} \enspace →[H3O+]=Ka[HCOOH][HCOO−] \enspace [H_3O^+] = K_{a}\frac{[HCOOH]}{[HCOO^-]}


            ′After′ [H3O+]=0.60.4Ka=2.7∗10−4'After' \, [H_{3}O^+] = \frac{0.6}{0.4}K_a = 2.7 * 10^{-4}

            ′After′ pH=−log[2.7∗10−4]=3.57'After' \,pH = -log[2.7 * 10^{-4}] = 3.57


            This shows the effect of a buffer solution. 0.1 mol acid was added to the buffer, and the pH change was from 3.74 to 3.57, only 0.16 units. Consider that 0.1 mol H3O+ added to 1L pure water (pH 7) would give a pH of 1, a change of 6 units!

          • Buffer solutions can resist changes in pH because of the equilibrium between the weak acid/base and its conjugate pair. The equilibrium will simply shift to remove or produce more H3O+ to oppose the change made when you added strong acid or base! See the diagram below, where a buffer is made with equal amounts of conjugate acid and base.
        • The pH of a buffer is also unaffected by dilution. This is unlike acids or bases on their own; diluting a strong acid solution tenfold will decrease H3O+ concentration tenfold, and increase pH by 1. However, Buffers do not change pH when diluted because the conjugate acid and conjugate base in them are diluted equally.
          For example, take a buffer solution with equal amounts of methanoic acid, HCOOH, and methanoate, HCOO-. The Ka expression would be:

          Ka=[HCOO−][H3O+][HCOOH]where[HCOOH]=[HCOO−] K_a = \frac{[HCOO^-][H_3O^+]}{[HCOOH]} \enspace where \enspace [HCOOH] = [HCOO^-]


          If the buffer was 1M HCOOH and 1M HCOO-, a tenfold dilution would decrease the concentration of both to 0.1M. If conjugate acid and base are equimolar, they will cancel out anyway: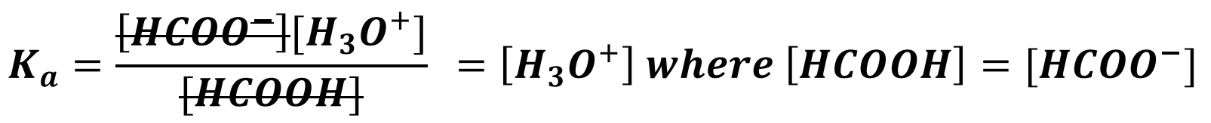

          buffer solutions weak acid or base ph = pka

          The above equation shows that in an equimolar buffer (where acid and base concentrations are equal), Ka is equal to H3O+ therefore pKa = pH.
          Even in a buffer where acid and base concentration are not equal, dilution does not change the [acid]/[base] concentration ratio. For example, 1M acid with 0.6M base diluted tenfold will
          Both of these scenarios the pH of buffers are unaffected by dilution because both acid and base are diluted equally!

        • The effect that adding strong base/acid to a weak acid/base buffer solution, when plotted on a curve, resembles a titration curve – the middle section where pH changes slowly is known as the ‘buffer region’ where the buffer equilibrium is established. The exact middle of this buffer region represents the pKa of the buffer, which is equal to the pH when there is a 1:1 conjugate acid:base ratio in the solution.

        • The effect of a buffer is seen when a weak acid is being titrated by a strong base, in the initial part called the buffer region. See the image below:

          During the beginning of the titration, while base is being added, there is only a very small increase in pH. This is because as the OH- base is being added, most of it is being reacted away by the H+ from the weak acid.
          The weak acid’s Ka equilibrium, responding to a drop in [H+] now shifts to produce more H+, which will need to be reacted away by more OH- from the strong base!
          To find the pH in a weak acid-strong base titration, chemists use the Henderson-Hasselbach equation:

          pH=pKa+log([Base][Acid])\large pH = pK_a + log(\frac{[Base]}{[Acid]})


          This equation is very useful to find the Ka of an unknown weak acid using the pH1/2, which is the pH when half of the volume of base at equivalence point has been added.
          At pH1/2, you have added half as much base as there originally was acid.
          Because half of the acid has been neutralised, you now have equal amounts of base and acid in your mixture. This makes the Henderson-Hasselbach equation a lot simpler: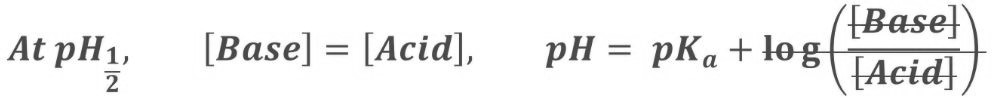

          buffer solutions weak acid or base ph = pka

          Therefore, at pH1/2:

          pH=pKa\large pH = pK_a


          Use the antilog (10-x) from this to find Ka.

        • The Henderson-Hasselbach equation can also be used to find the quantities needed to prepare buffers with a specific pH.
          Using the equation, you can solve for a ratio of acid to base that is required for the buffer to give a specific pH.
          This can be used for concentration, multiplied by your volume to find the number of moles you will need to originally add to create the buffer.