Sign In
Sign Up
flag-us

Area of Polar Curves: Mastering Calculations and Techniques
Unlock the power of polar curve area calculations with our in-depth guide. Learn essential formulas, tackle complex problems, and enhance your mathematical skills for academic success.

  1. Intros0/1 watched
  2. Examples0/4 watched
  1. 0/1
  2. 0/4
Now Playing:Area of polar curves– Example 0
Intros
0/1 watched
  1. Area of Polar Curve Overview
Examples
0/4 watched
  1. Area Enclosed with One Polar Equation
    Find the area of the inner loop of r=1+2sin⁡θr=1+2\sin\theta.
    Defining curves with parametric equations
    Jump to:NotesConceptFAQsPrerequisitesRelated
    Notes
    In this section, we will learn how to find the area of polar curves. For polar curves, we do not really find the area under the curve, but rather the area of where the angle covers in the curve. Note that not only can we find the area of one polar equation, but we can also find the area between two polar equations. It is important to always draw the curves out so that you can locate the area you are integrating, and write the integral correctly. We will first examine a generalized formula in finding areas of polar curves. Then we will apply this to finding areas with one polar curve and then two polar curves. Make sure you know your trigonometric identities very well before tackling these questions.
    To find the area of a single polar equation, we use the following formula:
    A=∫αβ12r2dθA=\int_{\alpha}^{\beta}\frac{1}{2}r^2d\theta
    where α\alpha is the starting angle and β\beta is the ending angle.

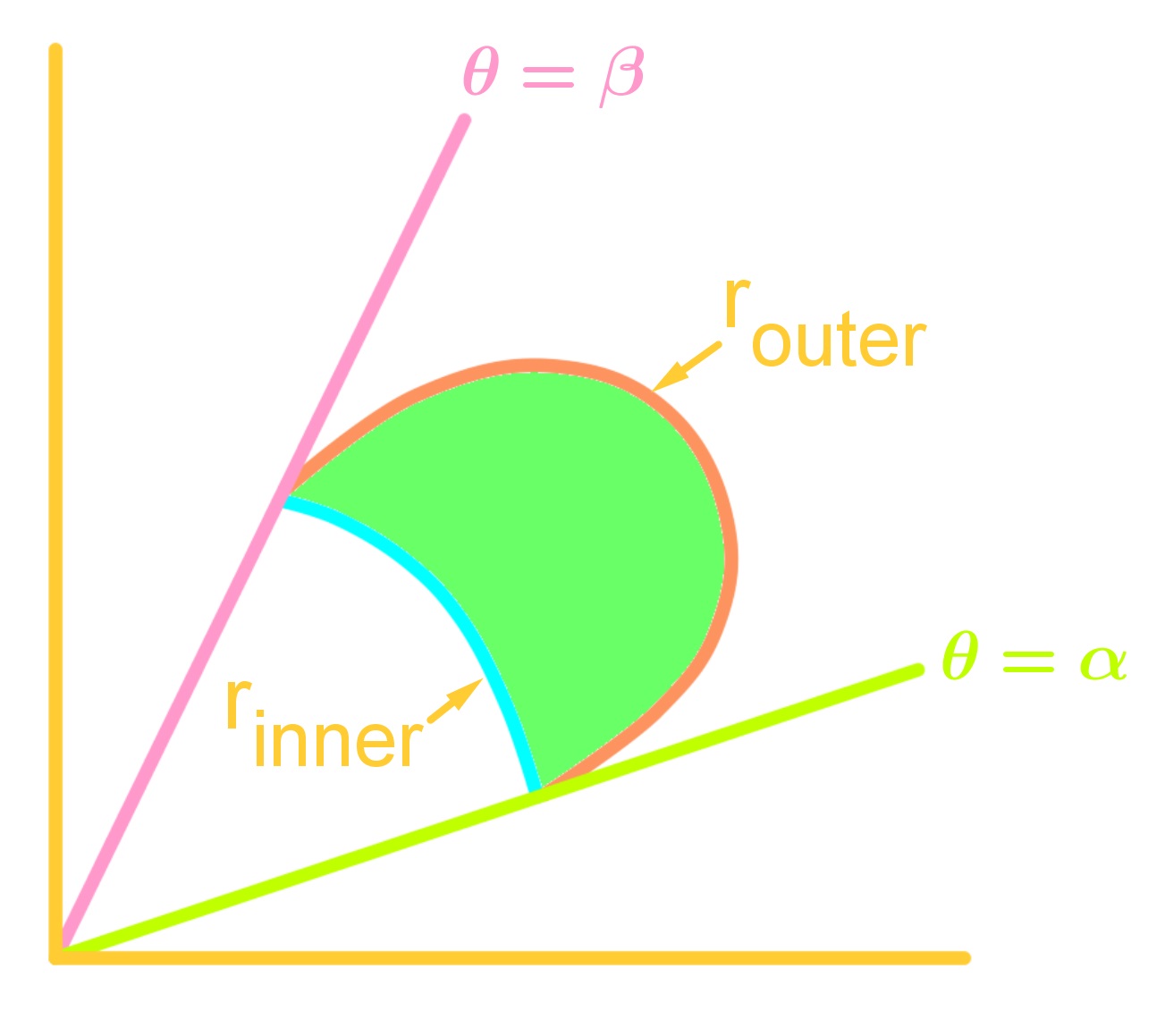
    To find the area that is enclosed by two polar equations like in the picture below, we use the formula:
    A=∫αβ12(r2  outer−r2  inner)dθA=\int_{\alpha}^{\beta}\frac{1}{2}(r^{2}\;_{outer}-r^{2}\;_{inner})d\theta
    where routerr_{outer} is the outer part of the first polar equation, and rinnerr_{inner} is the inner part of the second polar equation.
    area of polar curves
    Concept

    Introduction to Area of Polar Curves

    Welcome to the fascinating world of polar curves and their areas. This section delves into the intriguing concept of finding the area enclosed by polar curves, a crucial topic in advanced mathematics. We begin with an introductory video that provides a visual foundation for understanding polar curves and their unique properties. This video is essential for grasping the fundamental concepts before diving deeper into calculations. As we progress, we'll explore the methods for calculating the area of polar curves, focusing on both single equations and the more complex scenarios involving areas between two polar equations. The polar area formula will be a key tool in our calculations, allowing us to determine the precise area enclosed by these curves. Whether you're a student or an enthusiast, mastering polar curves will enhance your mathematical skills and provide insights into various real-world applications of polar curves, from engineering to physics.

    FAQs

    Here are some frequently asked questions about the area of polar curves:

    1. How do you find the area of a polar curve?

    To find the area of a polar curve, use the formula A = (1/2) r^2 dθ, where r is the polar function and θ is the angle. Integrate this from the starting angle α to the ending angle β. For a complete curve, typically integrate from 0 to 2π.

    2. What is the formula for the area between two polar curves?

    The formula for the area between two polar curves is A = (1/2) (r_outer^2 - r_inner^2) dθ, where r_outer is the function for the outer curve and r_inner is the function for the inner curve. Integrate from the starting angle α to the ending angle β where the curves intersect.

    3. How do you determine the limits of integration for polar area problems?

    To determine the limits of integration, find the points where the curves intersect by setting the equations equal to each other and solving for θ. These intersection points will typically be your α and β values. For a single curve, consider its period or symmetry to determine appropriate limits.

    4. What are some common challenges when solving polar area problems?

    Common challenges include graphing polar curves accurately, identifying symmetry, dealing with complex trigonometric expressions, setting up integrals correctly, and avoiding mistakes like forgetting to square r in the area formula or misinterpreting negative r values.

    5. How can I improve my skills in calculating polar curve areas?

    To improve your skills, practice regularly with various problem types, visualize curves before calculations, double-check your work (especially integral limits), use technology to verify results when possible, and review trigonometric identities. Consistent practice and patience are key to mastering this topic.

    Prerequisites

    When delving into the fascinating world of polar curves and their areas, it's crucial to have a solid foundation in several key mathematical concepts. Understanding these prerequisite topics will not only make learning about the area of polar curves easier but also provide a deeper appreciation for the interconnectedness of mathematical ideas.

    First and foremost, a strong grasp of polar coordinates is essential. This coordinate system, which uses distance from a central point and an angle to describe position, forms the basis for working with polar curves. Familiarity with angle bounds in polar coordinates will help you visualize and analyze these curves more effectively.

    Additionally, knowledge of trigonometric functions plays a vital role in understanding polar curves. These functions are integral to describing the shapes and behaviors of polar curves, and their properties are frequently used in calculations involving areas.

    Building on this, proficiency in trigonometric identities is crucial. These identities often come into play when simplifying complex expressions or solving integrals related to polar curve areas. Being able to manipulate and apply these identities efficiently can significantly streamline your calculations.

    Another important aspect to consider is the arc length of polar curves. While this might seem tangential, understanding arc length calculations can provide valuable insights into the nature of polar curves and their areas. Concepts like symmetry in polar curves, which are explored in arc length studies, can be applied to area calculations as well.

    Lastly, don't underestimate the importance of basic algebraic skills, particularly those related to solving equations. When working with polar curves, you'll often encounter equations that need to be solved or manipulated. The ability to handle these equations confidently will be invaluable in your study of polar curve areas.

    By mastering these prerequisite topics, you'll be well-equipped to tackle the complexities of calculating areas of polar curves. Each concept builds upon the others, creating a robust framework for understanding this advanced mathematical topic. Remember, mathematics is a cumulative discipline, and investing time in these foundational areas will pay dividends as you progress to more complex subjects like the area of polar curves.