Sign In
Sign Up
flag-us

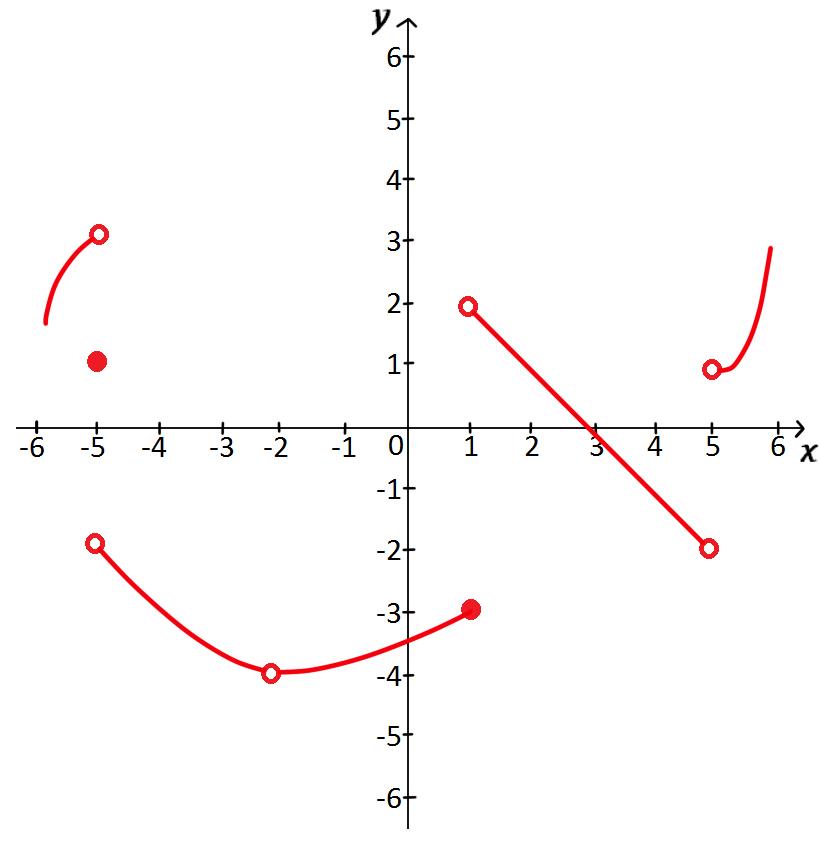
Finding limits from graphs

Get the most by viewing this topic in your current grade. Pick your course now.

Now Playing:Finding limits from graphs – Example 1a
Examples
  1. For the function f whose graph is shown, state the following:
    Finding limits from graphs
    1. lim⁡x→−5−f(x)\lim_{x \to -5^-} f(x)
      lim⁡x→−5+f(x)\lim_{x \to -5^+} f(x)
      lim⁡x→−5f(x)\lim_{x \to -5} f(x)
      f(−5)f(-5)

    2. lim⁡x→−2−f(x)\lim_{x \to -2^-} f(x)
      lim⁡x→−2+f(x)\lim_{x \to -2^+} f(x)
      lim⁡x→−2f(x)\lim_{x \to -2} f(x)
      f(−2)f(-2)

    3. lim⁡x→1−f(x)\lim_{x \to 1^-} f(x)
      lim⁡x→1+f(x)\lim_{x \to 1^+} f(x)
      lim⁡x→1f(x)\lim_{x \to 1} f(x)
      f(1)f(1)

    4. lim⁡x→4−f(x)\lim_{x \to 4^-} f(x)
      lim⁡x→4+f(x)\lim_{x \to 4^+} f(x)
      lim⁡x→4f(x)\lim_{x \to 4} f(x)
      f(4)f(4)

    5. lim⁡x→5−f(x)\lim_{x \to 5^-} f(x)
      lim⁡x→5+f(x)\lim_{x \to 5^+} f(x)
      lim⁡x→5f(x)\lim_{x \to 5} f(x)
      f(5)f(5)

Introduction to Calculus - Limits
Jump to:Notes
Notes
Limit is an important instrument that helps us understand ideas in the realm of Calculus. In this section, we will learn how to find the limit of a function graphically using one-sided limits and two-sided limits.
DEFINITION:
left-hand limit: lim⁡x→a−f(x)=L\lim_{x \to a^-} f(x) = L
We say "the limit of f(x), as x approaches a from the negative direction, equals L".
It means that the value of f(x) becomes closer and closer to L as x approaches a from the left, but x is not equal to a.

DEFINITION:
right-hand limit: lim⁡x→a+f(x)=L\lim_{x \to a^+} f(x) = L
We say "the limit of f(x), as x approaches a from the positive direction, equals L".
It means that the value of f(x) becomes closer and closer to L as x approaches a from the right, but x is not equal to a.

DEFINITION:
lim⁡x→af(x)=L\lim_{x \to a} f(x) = L if and only if lim⁡x→a+f(x)=L\lim_{x \to a^+} f(x) = L and lim⁡x→a−f(x)=L\lim_{x \to a^-} f(x) = L