Length contraction & relativistic momentum, mass and energy

In this lesson, we will learn:

• How to find the length contraction?
• How to calculate the relativistic momentum?
• The relation between total energy, kinetic energy and rest energy
• Relativistic Addition of Velocities

Notes:

In addition to the time interval difference in diverse frames of references, lengths and distances also vary from one frame to another.

Let’s consider a spaceship travelling at very high speed from Earth to another planet, and two different frame of references to calculate the length contraction.

1. Observer on earth


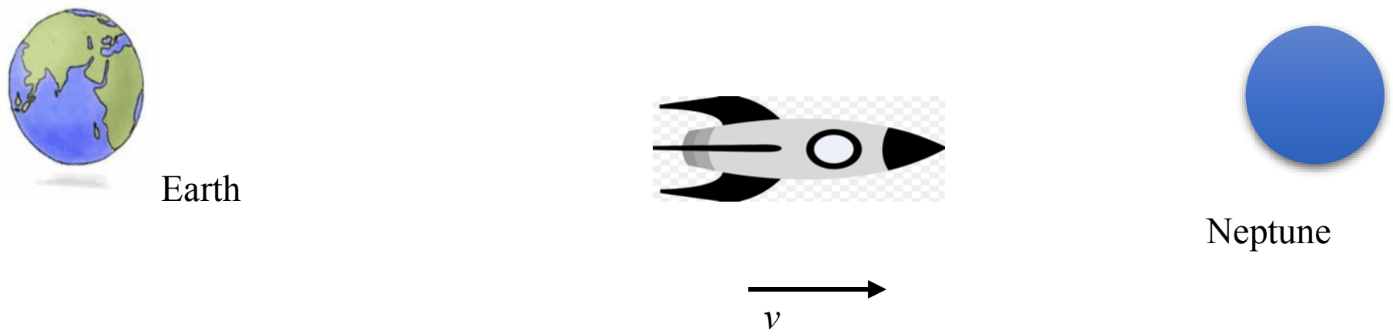
$L_{0}$: Distance between plants as measured by the observer (proper length)
$\triangle t$: Time required for the trip measured form Earth

$\triangle t = \frac{L_{0}}{v}$

2. Observer in the spaceship

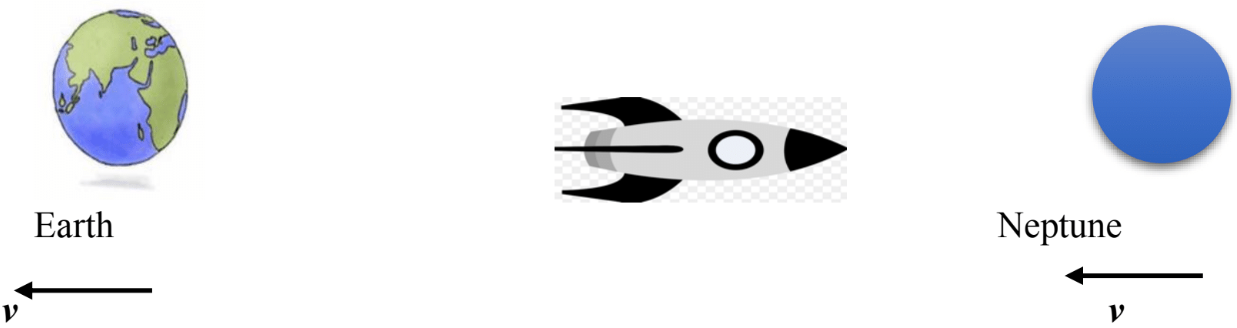
The time interval for the observer in the spacecraft is shorter, since the spacecraft is moving. So the time for the trip according to the time dilation equation would be;

$\triangle t_{0} = \triangle t \sqrt{(1 - v^{2} / c^{2} }$

$L$: the distance between the plants as viewed by the spacecraft observer.

$L = v\triangle t_{0} = v \triangle t\sqrt{(1 - v^{2} / c^{2})} = L_{0}\sqrt {(1 - v^{2} / c^{2})}$



$\gamma =$ $\large\frac{1}{\sqrt{(1 - v^{2} / c^{2}) }}$$\quad \Rightarrow \quad L = \frac{L_{0}} {\gamma}$

therefore; the length of the object is measured to be shorter when it is moving relative to the observer than when it is at rest. (length Contraction)

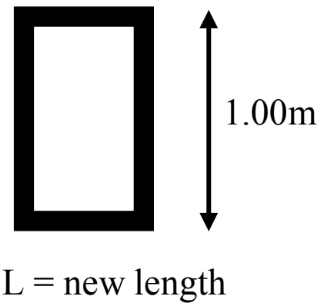
Note: the length contraction occurs only along the direction of the motion. If the object is moving along $x$-axis, the length is shortened but the height stays the same.

For example, if there is a rectangular painting (1.00m by 1.50m) hung on the wall of a spaceship which is moving at a speed of 0.8c, contraction occurs along the length only.



$L = L_{0}\sqrt {(1 - v^{2} / c^{2}) } =$ (1.50$m$) $\sqrt{(1 - (0.8c)^{2} / c^{2})} =$ 0.9$m$



Relativistic Momentum

In non-relative situation momentum is equal to $p = m_{0}v$ (classical momentum),
In relativistic situation the rest mass ($m_{0}$) increases with the speed and is called relativistic mass ($m_{rel}$)

$m_{rel} =$ $\large \frac{m_{0}} {\sqrt{(1 - v^{2} / c^{2})} }$ $= \gamma \,m_{0}$

therefore, the relativistic momentum can be calculated using the following equation

$p = m_{rel} v = \gamma \, m_{0} v =$ $\large \frac{m_{0} v} {\sqrt{(1 - v^{2} / c{2})} }$

Mass and Energy

The total amount of energy possessed in this case is the total kinetic energy and the rest energy, $E_{total} = E_{k} + E_{0}$

The rest energy by Einstein is defined as $E_{0} = m_{0} c^{2}$

The total energy is also calculated by Einstein’s equation but the mass should be

the relativistic mass $E_{total} =m_{rel} \, c^{2} =$ $\large \frac{m_{0} \,c^{2}} {\sqrt{(1 - v^{2} /c^{2}) }}$

$E_{k} = E_{total} \,- \, E_{0} =$ $\large \frac{m_{0} \, c^{2}} {\sqrt{(1 - v^{2} / c^{2}) }}$ $- \,m_{0} \,c^{2} = m_{0} c^{2}$$\large (\frac{ 1 } { \sqrt{(1 - v^{2} / c^{2})} } - 1)$

$E_{k} = m_{0}c^{2}$ $\large (\frac{1}{\sqrt{(1 - v^{2} / c^{2}})} - 1)$

Relativistic Addition of Velocities
Relative Velocities do not add simply, as in classical mechanics ($v$ << $c$)


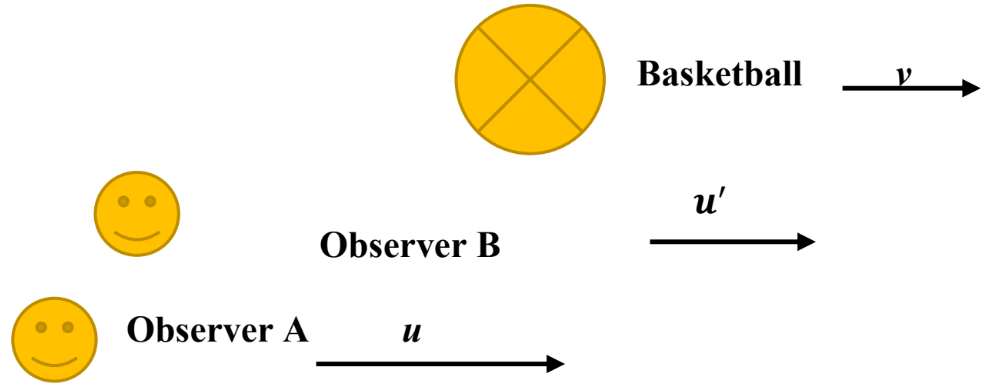
$u = \frac{v \, + \, u^{\prime} }{1 \, + \,v \,u^{\prime} / c^{2}} \qquad$ ($u^{\prime}$ and $v$ are in the same direction)