Refraction and Snell’s law

In this lesson, we will learn:

• Meaning of refractive index
• Snell’s law
• Calculating speed of light and angle of refraction in different media

• When light travels from one medium to another, the light is refracted: the direction of the light changes. This change in direction is caused by differences in the speed of light as it travels through different media:
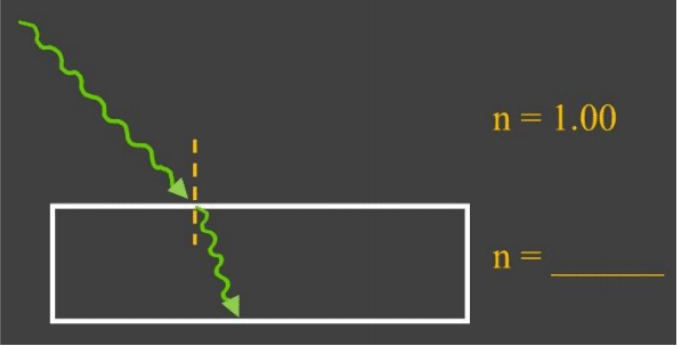
• When light travels from a medium where it moves faster to a medium where it moves slower, the light is refracted towards the normal.
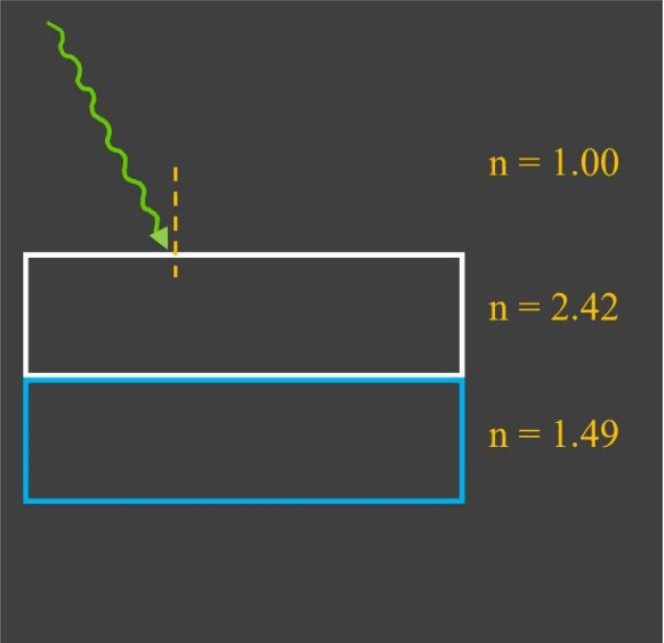
• When light travels from a medium where it moves slower to a medium where it moves faster, the light is refracted away from the normal.
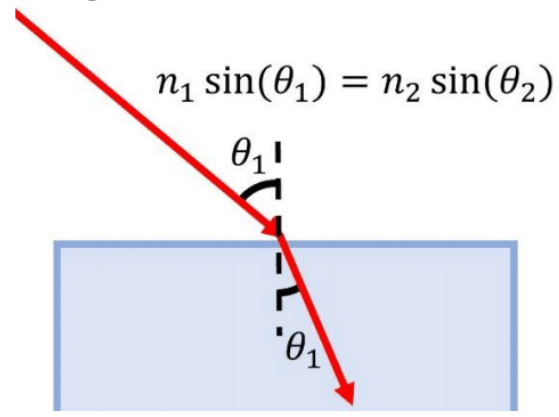
• Refractive index (n) is a unitless quantity associated with a medium that describes how much that medium refracts lights (i.e. a material possessing a larger refractive index indicates a greater change in the direction of light). When light passes from one medium to another, Snell’s law relates the refractive indices of the two media to the angle of incidence and angle of refraction of the light:
In the above diagram, $\theta$₁ is the angle of incidence and $\theta$₂ is the angle of refraction. These angles are measured from the ray of light to the normal, a line that is perpendicular to the surface of the new medium.
• The refractive index is also related to the speed of light inside the medium by the following expression:

n = $\large \frac{c}{v}$
We can see in the above expression that n is inversely proportional to v (i.e. light travels slower in media with larger n). Therefore, the above relationships between v and the direction light bends in can be restated in terms of n:
• When light travels from a medium with lower n (faster) to a medium with higher n (slower), the light is refracted towards the normal.
• When light travels from a medium with higher n (slower) to a medium with lower n (faster), the light is refracted away from the normal.