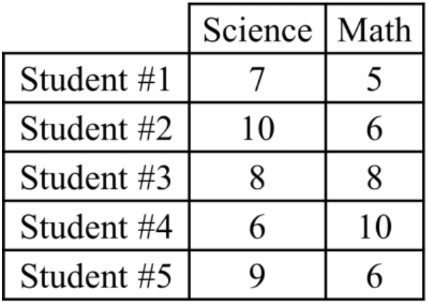
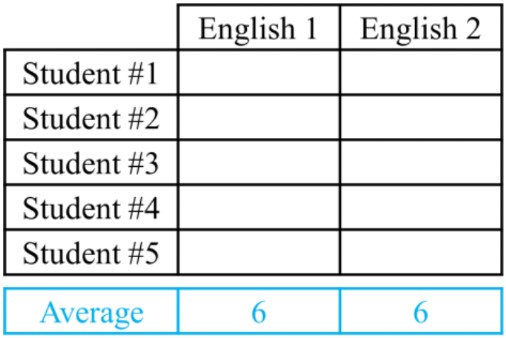
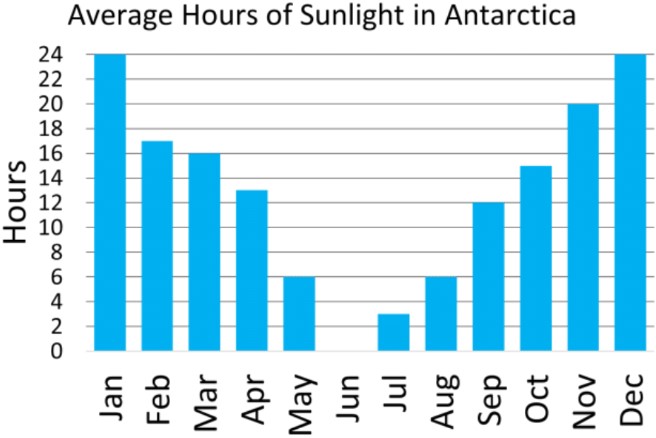
In this lesson, we will learn:
- How to calculate the four types of measures of central tendency for a data set: mean, median, mode, and range
Notes:
- Measures of central tendency refer to types of calculations done with data set numbers. A single number will describe the most common (central; middle) behaviors of the information you have collected.
- When doing experiments or surveys, the steps are to:
- (1) gather data
- (2) organize data
- (3) analyze data
- Analyzing data means that we want to make sense of all the information that we've gathered. What does the data tell us? What can we learn from it?
- There are four measures of central tendency: mean, median, mode and range.
- Range is the difference between the highest and lowest number
- It represents how far the data set is spread (how much it varies)
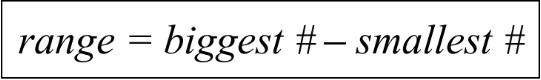
- Median is the number listed in the middle of the data set (in order)
- A quick way to find the approximate center of the data
- To find the median, first list all numbers from least to greatest. Then count the number of data points (is there a total number that is odd or even?)
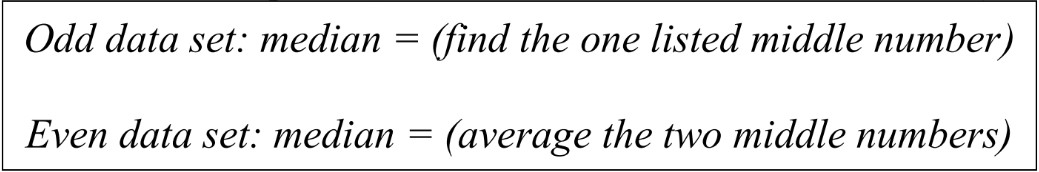
- Mode is the most common data value; the one that shows up the most frequently
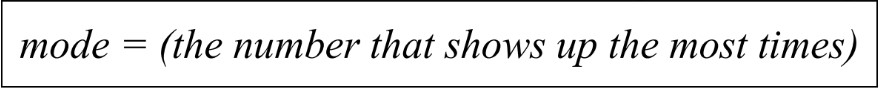
- Mean (also called average) is the sum of all data points divided by the number of data points
- This is a better way to find the true middle of the data set; it spreads all the values across all data points, giving a better idea of what is happening overall