Implicit Differentiation: Unlocking Complex Mathematical Relationships Discover the power of implicit differentiation to solve challenging equations, find derivatives of complex functions, and apply this essential calculus technique to real-world scenarios in physics and engineering.
- The graph shows a circle centred at the origin with a radius of 5.
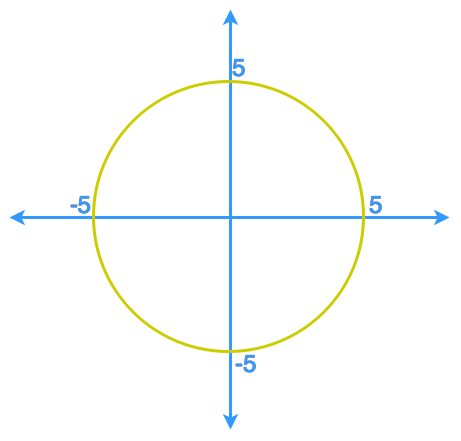
a) Define the circle implicitly by a relation between x and y .
b) Define the circle by expressing y explicitly in terms of x .
c) Use the method of "explicit differentiation" to find the slope of the tangent line to the circle at the point (4, -3).
d) Use the method of "implicit differentiation" to find the slope of the tangent line to the circle at the point (4, -3). - 3y4+5x2y3−x6=2x−9y+1
Use implicit differentiation to find: dxdy
Free to Join!
Easily See Your Progress
 We track the progress you've made on a topic so you know what you've done. From the course view you can easily see what topics have what and the progress you've made on them. Fill the rings to completely master that section or mouse over the icon to see more details.
We track the progress you've made on a topic so you know what you've done. From the course view you can easily see what topics have what and the progress you've made on them. Fill the rings to completely master that section or mouse over the icon to see more details.Make Use of Our Learning Aids
Earn Achievements as You Learn
 Make the most of your time as you use StudyPug to help you achieve your goals. Earn fun little badges the more you watch, practice, and use our service.
Make the most of your time as you use StudyPug to help you achieve your goals. Earn fun little badges the more you watch, practice, and use our service.Create and Customize Your Avatar
 Play with our fun little avatar builder to create and customize your own avatar on StudyPug. Choose your face, eye colour, hair colour and style, and background. Unlock more options the more you use StudyPug.
Play with our fun little avatar builder to create and customize your own avatar on StudyPug. Choose your face, eye colour, hair colour and style, and background. Unlock more options the more you use StudyPug.
Introduction to Implicit Differentiation
Implicit differentiation is a powerful technique in calculus that allows us to find derivatives of functions that are not easily expressed in explicit form. The introduction video provides a crucial foundation for understanding this concept, demonstrating its application in real-world scenarios. Unlike explicit functions, where y is directly expressed in terms of x, implicit functions define a relationship between variables without isolating one variable. This distinction is key to grasping the need for implicit differentiation. Explicit functions, such as y = x^2, clearly show y as a function of x. In contrast, implicit functions, like x^2 + y^2 = 1, express a relationship between variables without solving for y explicitly. Implicit differentiation becomes essential when dealing with these complex equations, enabling us to find derivatives and solve problems that would be challenging or impossible using traditional differentiation methods. This technique expands our ability to analyze and work with a wider range of mathematical relationships.
Q1: What is implicit differentiation and why is it important?
A1: Implicit differentiation is a technique used to find derivatives of functions that are not explicitly defined in terms of one variable. It's important because it allows us to handle complex equations where y cannot be easily isolated, such as conic sections or equations involving trigonometric, logarithmic, or exponential functions. This method expands our ability to analyze a wider range of mathematical relationships and solve problems in various fields like physics and engineering.
Q2: How does implicit differentiation differ from explicit differentiation?
A2: Explicit differentiation involves finding the derivative of a function where y is clearly expressed in terms of x (e.g., y = x^2). Implicit differentiation, on the other hand, is used when the relationship between x and y is given by an equation where y is not isolated (e.g., x^2 + y^2 = 25). In implicit differentiation, we treat y as a function of x and apply the chain rule, differentiating both sides of the equation with respect to x.
Q3: What are the key steps in performing implicit differentiation?
A3: The key steps are: 1) Identify the implicit relation. 2) Differentiate both sides of the equation with respect to x, treating y as a function of x. 3) Apply the chain rule to terms containing y, multiplying by dy/dx. 4) Group terms containing dy/dx on one side of the equation. 5) Solve for dy/dx, expressing it in terms of x and y.
Q4: What are some common mistakes to avoid in implicit differentiation?
A4: Common mistakes include forgetting to use the chain rule when differentiating y terms, incorrectly applying the product or quotient rule, neglecting to differentiate both sides of the equation, mishandling constants, and failing to isolate dy/dx at the end of the process. It's crucial to treat y as a function of x throughout the differentiation process and consistently apply dy/dx to each y term.
Q5: Where is implicit differentiation applied in real-world scenarios?
A5: Implicit differentiation has numerous real-world applications. In physics, it's used to analyze motion in constrained systems and planetary orbits. Engineers use it in thermodynamics for understanding heat transfer and in electrical engineering for analyzing non-linear circuits. It's also applied in economics for analyzing utility functions, in computer graphics for creating realistic simulations, and in medical imaging for improving image reconstruction algorithms.
Understanding implicit differentiation requires a solid foundation in several key mathematical concepts. One of the most fundamental is the relationship between two variables. This concept is crucial because implicit differentiation often deals with equations where variables are not explicitly solved for one another.
Building on this, students should be comfortable with solving linear equations with variables on both sides. This skill is essential when rearranging complex equations during the implicit differentiation process. Additionally, familiarity with composite functions is vital, as many implicit functions involve nested relationships between variables.
As we delve deeper into calculus, the power rule becomes indispensable. This rule forms the basis for differentiating many functions and is frequently applied in implicit differentiation. Closely related is the chain rule, which is crucial when dealing with composite functions in implicit form.
Understanding the derivative of exponential functions is another key prerequisite. Many implicit equations contain exponential terms, and knowing how to differentiate these is essential. Similarly, the power of a product rule and the power of a quotient rule are important algebraic concepts that translate directly to their calculus counterparts in differentiation.
Finally, grasping the concept of the slope and equation of tangent line is crucial. Implicit differentiation often involves finding the slope of a curve at a specific point, which is fundamentally what a derivative represents.
By mastering these prerequisite topics, students will be well-prepared to tackle the challenges of implicit differentiation. Each concept builds upon the others, creating a strong foundation for understanding this advanced calculus technique. Remember, implicit differentiation is not just about applying a set of rules, but about understanding how these underlying principles work together to solve complex mathematical problems.



