Introduction
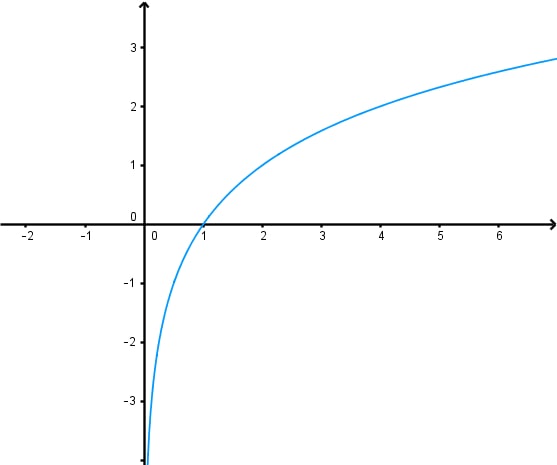
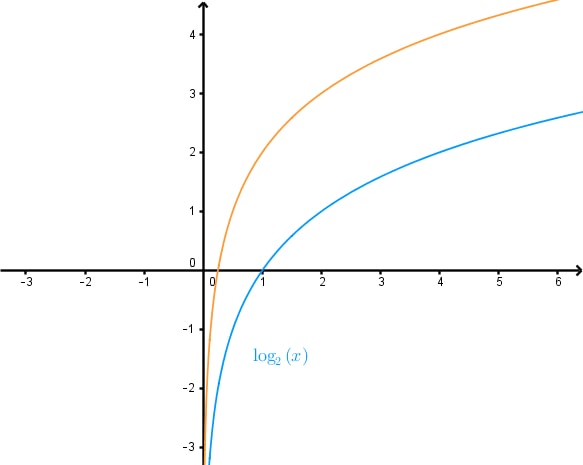
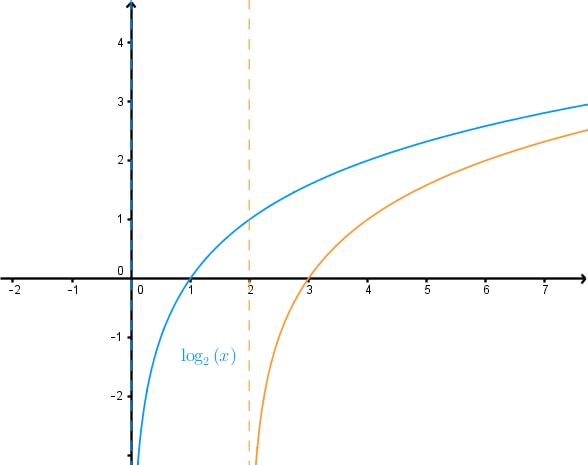
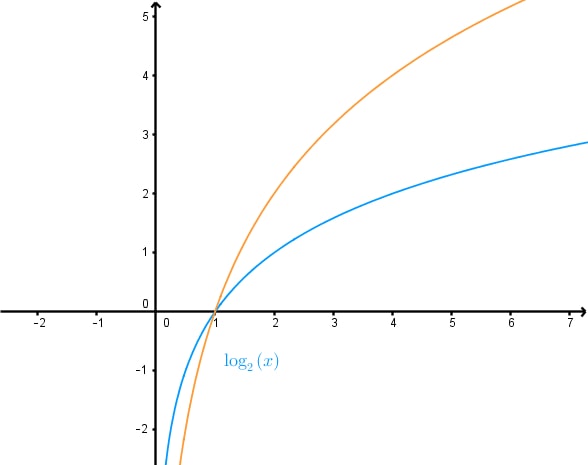
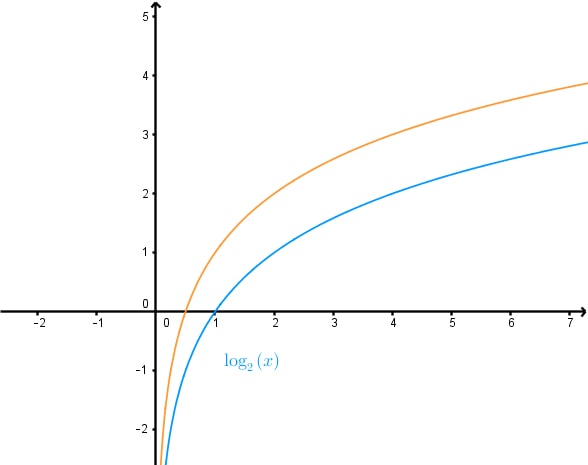
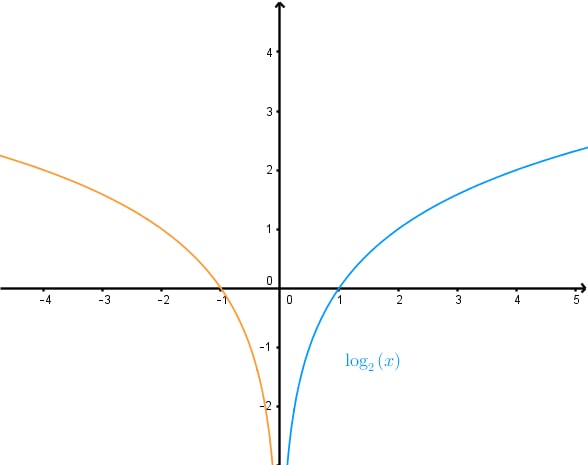
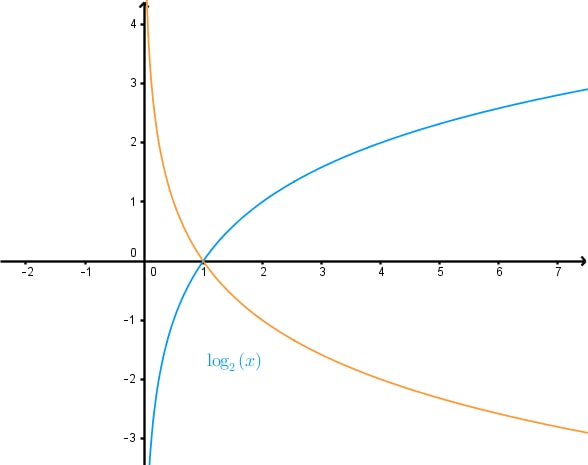
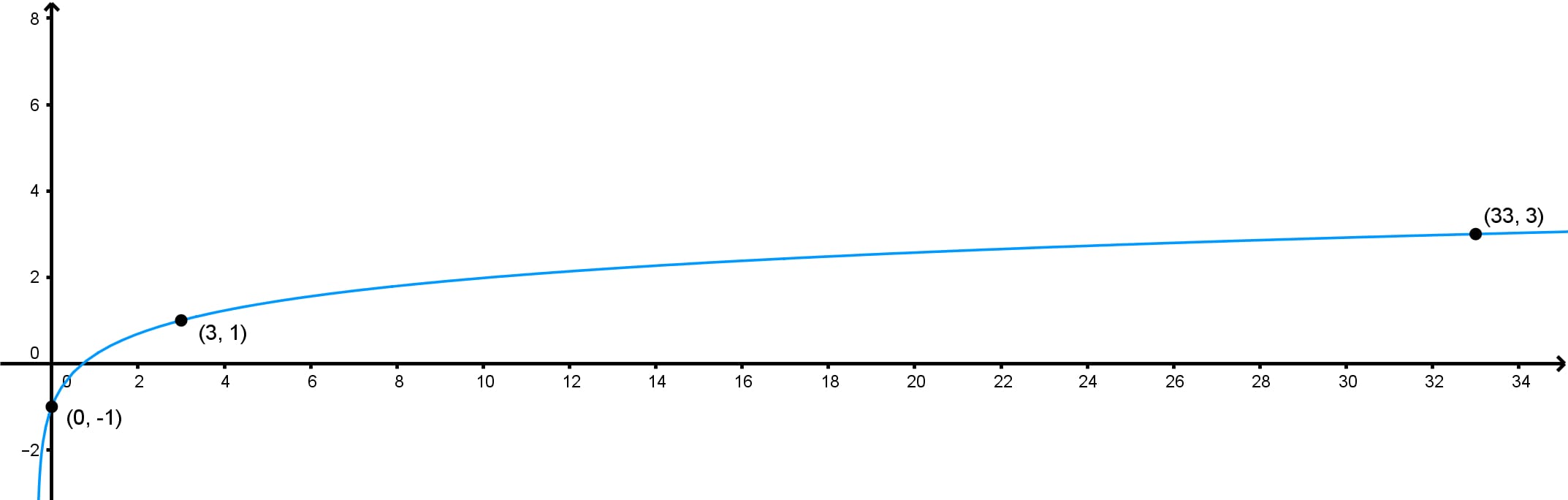
Finding a logarithmic function from its graph is a crucial skill in mathematics. This topic explores the process of identifying key features and using them to construct the equation of a logarithmic function. The introduction video serves as an essential starting point, providing a visual and conceptual foundation for understanding logarithmic graphs characteristics. By watching this video, students can grasp the fundamental characteristics of logarithmic functions, such as their shape, asymptotes, and intercepts. Logarithmic graphs characteristics play a significant role in various fields, including science, engineering, and economics. They are particularly useful for modeling phenomena that exhibit exponential decay modeling. Mastering the ability to interpret and derive logarithmic functions from graphs enhances problem-solving skills and deepens mathematical understanding. This knowledge is invaluable for advanced mathematical concepts and real-world applications, making it a cornerstone of mathematical education.