Sign In
Sign Up
flag-us

Mastering Isotopes: Key Concepts in Chemistry
Dive into the world of isotopes! Understand their definition, unique properties, and wide-ranging applications in science. From carbon dating to medical diagnostics, discover the importance of isotopes in chemistry and beyond.

  1. Intros0/6 watched
  2. Examples0/3 watched
  1. 0/6
  2. 0/3
Now Playing:Isotopes – Example 0a
Intros
0/6 watched
  1. Introduction to isotopes
  2. Introduction to isotopes
    What is an isotope?
  3. Introduction to isotopes
    Why does Cl have atomic mass of 35.5?
Examples
0/3 watched
  1. Use the number of particles to identify elements, and use elements to determine number of particles.
    Complete the table below. When writing the chemical symbol, write the mass number, and charge on the particle with it.
    identify elements, and use elements to determine number of particles
    Introduction to chemical formulae
    Jump to:NotesConceptFAQsPrerequisites
    Notes
    In this lesson, we will learn:
    • The definition of an isotope and their difference in properties.
    • The definition of relative atomic mass and relative isotopic mass.
    • How isotopes come to affect the relative atomic mass of an element.
    • How to calculate relative mass of samples using relative abundance.
    • The method used to detect different isotopes and some of their uses.

    Notes:
    • So far, we have ignored the fact that many elements in the Periodic Table have decimal numbers in their relative atomic mass.
      • If atomic mass is a measure of the number of protons and neutrons in an atom, how is it possible to have atomic mass that isn't a whole number? Remember, you cannot have half a proton or half a neutron in an atom!

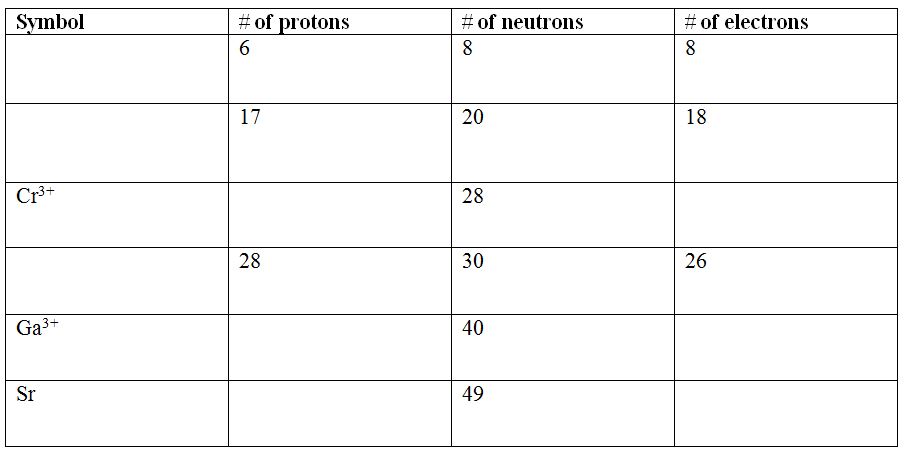
    • An isotope is an atom of an element with the same number of protons but a different number of neutrons. This gives an equal proton number (so by definition it's the same element) but a different mass number. The discovery of isotopes refuted Dalton’s claim of atoms that all atoms of an element were identical – this is not true!

    • Isotopes are why the periodic table contains decimals for many elements’ relative masses. Relative atomic mass for an element is an average value account for the masses and relative abundance of each isotope of an element.

    • “Relative” when talking about the mass of any atom or molecule, means relative to an atom of carbon-12. The mass of any isotope or atomic sample is defined as compared to the carbon-12 (12C) isotope:
      • The relative isotopic mass is the mass of an isotope relative to 1/12 of the mass of a 12C atom.
      • The relative atomic mass is the mass of any atomic sample relative to 1/12 of the mass of a 12C atom.

    • Any given element (defined by the proton number!) might have atoms with different numbers of neutrons. This element's range of atoms with different numbers of neutrons in them are its' isotopes.
      • For example, hydrogen atoms have only 1 proton, and can only have one proton.
        • Hydrogen atoms with zero neutrons are called Hydrogen-1. This is by far the most common isotope of hydrogen we observe. About 99.98% of hydrogen atoms are hydrogen-1.
        • Hydrogen atoms with one neutron are called Hydrogen-2 or deuterium. This only makes up about 0.02% of any sample of hydrogen atoms.
      • Another example: Carbon atoms have 6 protons in their nucleus and can only have 6 protons.
        • The most common isotope of carbon atoms is carbon-12, which has 6 protons and 6 neutrons in the nucleus. Around 98.9% of carbon atoms in any sample are carbon-12.
        • Carbon-13 is an isotope of carbon where the carbon atoms have 6 protons and 7 neutrons in the nucleus.
      • Isotopes are normally specified by giving their relative atomic mass: e.g. carbon-13, or hydrogen-2.

    • Because neutrons have no charge, the number of neutrons doesn't change an atom's chemical reactivity. Therefore isotopes of an element have identical chemical properties to each other isotope!

    • Because neutrons have a relative atomic mass of 1 amu (the same as protons), isotopes do affect the relative atomic mass of elements as they are written in the periodic table. Ice cubes made of normal water (H2O) are less dense than liquid water. Ice cubes made with deuterated water (D2O), where the hydrogen atoms are hydrogen-2 atoms, sink in regular liquid water!

    • It is possible to calculate molar mass of an elemental sample when given relative abundance of each isotope and individual masses. You can use the formula:

      • relative atomic mass (r.a.m)=∑(M1∗RA1)+(M2∗RA2)+...(r.a.m)= \sum (M_{1}*RA_{1}) + (M_{2}*RA_{2}) + ...

      Where:
      • Mn is the relative isotopic mass of the nth isotope.
      • RAn is the relative abundance of the nth isotope, expressed as a decimal (for example 50% abundance is 0.5).

      An example calculation for this is with boron. Boron occurs as two stable isotopes: 10B which has 19.9% abundance and 11B which has 80.1% abundance. The calculation for the relative atomic mass of boron using these figures would be:

      (r.a.m)=∑(10∗0.199)+(11∗0.801)=10.801(r.a.m)= \sum (10 * 0.199) + (11 * 0.801) = 10.801

      Rearranging this equation, an isotope's relative abundance can be found if the relative mass is known and the other isotope abundances are too.

    • (IB) Mass spectrometry is very useful for identifying different isotopes in elemental samples. Because different isotopes (e.g. 12C and 13C) have identical chemical properties, you can’t separate or identify different isotopes using chemical reactions – they will react the same way!
      A mass spectrum shows the mass-to-charge ratio (m/z, effectively the mass) of atoms or molecules run through it. Since even a milligram of a substance contains billions of individual molecules or atoms, it can paint an accurate picture of the relative abundance of elemental atoms if an elemental substance was run through it.
      • An example of this is chlorine. In a mass spectrometer, chlorine shows clear signals at 35 and 37 m/z in a ratio of 3:1 abundance. This means that a given sample of chlorine will contain around 75% 35Cl atoms and 25% 37Cl atoms. Using the relative abundance calculation, this works out as 35.5, which is the atomic mass of chlorine as shown in the periodic table.
      There is much more detail on mass spectrometry in the lesson Mass spectrometry.

    • (IB) There are many examples of isotopes of an element that are unstable. Eventually, they will physically break down and release some form of radiation, becoming a different chemical species in the process. This time frame of them breaking down (measured by their half-life) varies by isotope; it can be a few minutes or thousands of years. Unstable isotopes that break down and release radiation are called radioisotopes.
      These are valuable as ‘tracer’ molecules where radioisotopes act as chemical ‘labels’ because we can measure the radiation released or the amount of radioisotope remaining. This has several real-world uses:
      • How much radioisotope remaining, compared to stable isotopes, could reveal the age of a sample. This is the basis of carbon dating. Living organisms continually ‘replace’ carbon, including the radioisotope 14C, during respiration and photosynthesis. Dead organisms do not, so levels of 14C start decreasing as it decays away, compared to the stable 12C.
        In this way, the ratio of 14C:12C found in a mass spectrum can estimate a sample’s age.
      • How much radiation is (not) detected can show us accumulation of a substance, for example in part of a human body. This is useful in nuclear medicine: cancer cells generally have a higher metabolic rate than regular cells, so glucose and oxygen are more concentrated in them. Fluorodeoxyglucose (FDG) is very similar in structure to glucose but it contains the radioisotope 18F. Because of the higher metabolism of cancer cells, FDG accumulates in cancer cells noticeably more than regular cells. This is observed in the radiation given off by 18F in positron emission tomography (PET).
    Concept

    Introduction to Isotopes

    Isotopes are a fundamental concept in chemistry, playing a crucial role in various scientific fields. This lesson begins with an engaging introduction video that sets the stage for our exploration of isotopes. Our main objectives are to grasp the definition of isotopes, understand their unique properties, and discover their wide-ranging applications. Isotopes are atoms of the same element with different numbers of neutrons, resulting in varying atomic masses. Their importance in chemistry cannot be overstated, as they provide valuable insights into atomic structure, radioactivity, and nuclear processes. Throughout this lesson, we'll delve into how isotopes are used in diverse areas such as carbon dating, medical diagnostics, and environmental studies. By the end of this module, you'll have a solid foundation in isotope chemistry, enabling you to appreciate their significance in both scientific research and practical applications. Join us on this exciting journey into the world of isotopes!

    FAQs

    1. What is an isotope?

      An isotope is an atom of a chemical element with the same number of protons but a different number of neutrons in its nucleus. This means isotopes have the same atomic number but different mass numbers. For example, carbon-12, carbon-13, and carbon-14 are all isotopes of carbon, each with 6 protons but 6, 7, and 8 neutrons, respectively.

    2. How are isotopes used in carbon dating?

      Carbon dating uses the radioactive isotope carbon-14 to determine the age of organic materials. Living organisms continuously absorb carbon-14 from the atmosphere. When an organism dies, it stops taking in new carbon, and the carbon-14 it contains begins to decay at a known rate. By measuring the ratio of carbon-14 to stable carbon-12 in a sample, scientists can calculate how long ago the organism died, up to about 50,000 years in the past.

    3. What are some medical applications of isotopes?

      Isotopes have numerous medical applications, particularly in diagnostics and treatment. In medical imaging, radioisotopes are used in techniques like PET (Positron Emission Tomography) scans to visualize metabolic processes in the body. For example, fluorine-18 is used in FDG-PET scans to detect cancer. In cancer treatment, targeted radiotherapy uses specific isotopes to deliver precise doses of radiation to tumors while minimizing damage to surrounding healthy tissue.

    4. How do isotopes contribute to environmental studies?

      Isotopes are valuable tools in environmental research. They are used as tracers to study water cycles, pollution dispersal, and ecosystem dynamics. For instance, oxygen and hydrogen isotopes in water molecules help track groundwater movement and understand precipitation patterns. Carbon isotopes are used to study the carbon cycle and historical atmospheric CO2 levels. These applications are crucial for climate studies, water resource management, and understanding environmental changes over time.

    5. What is the difference between stable and radioactive isotopes?

      Stable isotopes have a balanced ratio of protons to neutrons and do not undergo radioactive decay. They maintain their nuclear composition indefinitely. Examples include carbon-12 and oxygen-16. Radioactive isotopes, also known as radioisotopes, have unstable nuclei that decay over time, emitting radiation in the process. The rate of decay is measured by the isotope's half-life. Examples include carbon-14 and uranium-235. Both types of isotopes have important applications in science and industry, with stable isotopes often used in tracing and analysis, while radioactive isotopes are used in areas like nuclear medicine and radiometric dating.

    Prerequisites

    To fully grasp the concept of isotopes, it's crucial to have a solid foundation in several key areas of chemistry. One of the most fundamental prerequisites is understanding atomic structure. This knowledge forms the basis for comprehending how isotopes differ from one another at the subatomic level.

    Atomic structure is essential because isotopes are variants of a chemical element with the same number of protons but different numbers of neutrons. Without a clear understanding of how atoms are composed, it becomes challenging to differentiate between isotopes and regular atoms. This prerequisite topic helps students visualize the internal composition of atoms, which is crucial when discussing isotopic variations.

    Another critical prerequisite for understanding isotopes is familiarity with the physical properties of elements in the periodic table. This knowledge is vital because isotopes can exhibit slightly different physical properties due to their mass differences, even though they belong to the same element. Understanding how elements are organized in the periodic table and their characteristic properties provides context for how isotopes fit into the broader picture of chemical elements.

    The periodic table serves as a roadmap for elements and their isotopes. It helps students recognize patterns in atomic structure and properties, which is crucial when studying isotopes. For instance, knowing an element's atomic number and typical mass from the periodic table allows students to identify when an atom of that element has an unusual number of neutrons, indicating an isotope.

    Lastly, a working knowledge of mass spectrometry techniques is incredibly beneficial when delving into the study of isotopes. Mass spectrometry is a powerful analytical tool used to measure the mass-to-charge ratio of ions, making it invaluable for identifying and quantifying different isotopes of an element.

    Understanding mass spectrometry helps students appreciate how scientists can precisely determine the abundance of various isotopes in a sample. This technique is fundamental in fields such as radiometric dating, nuclear physics, and environmental science, where isotope analysis plays a crucial role. By grasping the principles of mass spectrometry, students can better understand how isotopes are detected, measured, and applied in real-world scientific research and applications.

    In conclusion, a strong grasp of atomic structure, properties of elements in the periodic table, and mass spectrometry techniques provides the necessary foundation for understanding isotopes. These prerequisite topics interweave to create a comprehensive framework that allows students to fully appreciate the significance and applications of isotopes in various scientific disciplines.