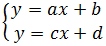Introduction to Systems of Linear Equations
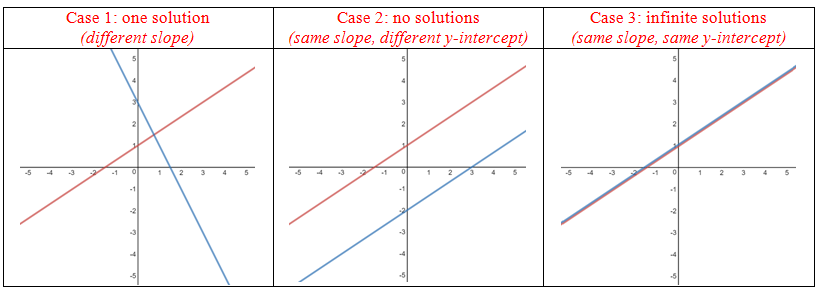
Systems of linear equations are fundamental in mathematics, playing a crucial role in various fields such as physics, engineering, and economics. Our introduction video serves as an essential starting point for understanding this concept, providing a visual and intuitive approach to grasping the basics. In this article, we'll delve deeper into the nature of linear equations and explore how to determine the number of solutions in a system. Linear equations are characterized by variables raised to the first power and the absence of products between variables. When multiple linear equations are combined, they form a system. These systems can have one unique solution, infinitely many solutions, or no solution at all. Understanding how to analyze and solve these systems is key to mastering more advanced mathematical concepts. We'll guide you through the process of identifying the number of solutions, equipping you with valuable problem-solving skills applicable in real-world scenarios.
Systems of linear equations are a crucial topic in algebra. By using a visual approach to linear equations, students can better understand the relationships between variables. One important aspect is determining the number of solutions a system can have. This can range from a single unique solution to no solution in linear systems at all. Mastering these concepts is essential for progressing in mathematics.