Inverse functions

Everything You Need in One Place

Homework problems? Exam preparation? Trying to grasp a concept or just brushing up the basics? Our extensive help & practice library have got you covered.

Learn and Practice With Ease

Our proven video lessons ease you through problems quickly, and you get tonnes of friendly practice on questions that trip students up on tests and finals.

Instant and Unlimited Help

Our personalized learning platform enables you to instantly find the exact walkthrough to your specific type of question. Activate unlimited help now!

Make math click 🤔 and get better grades! 💯Join for Free

Get the most by viewing this topic in your current grade. Pick your course now.

Intros

Lessons

• What is "inverse", and what does "inverse" do to a function?
• Inverse: switch "x" and "y"
• Inverse: reflect the original function in the line "y = x"

Examples

Lessons

Graph an inverse
Given the graph of $y = f\left( x \right)$ $y = f (x)$ as shown,
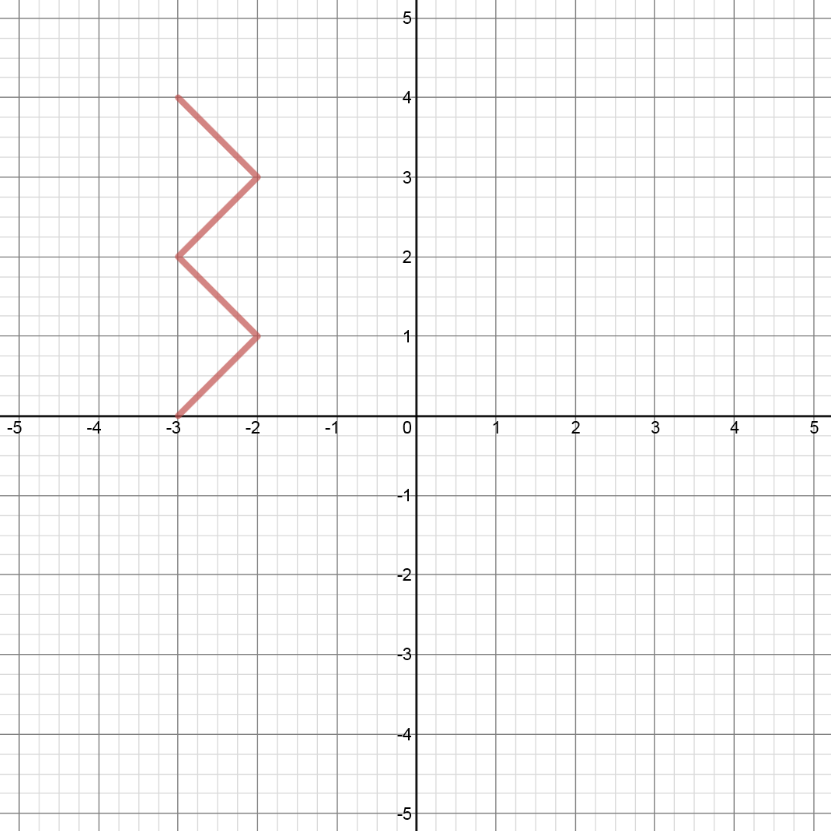
1. Sketch the graph of the inverse $y = {f^{ - 1}}\left( x \right)$ in 2 ways:
  i) by reflecting $f\left( x \right)$ in the line $y = x$
  ii) by switching the x and y coordinates for each point on $f\left( x \right)$
2. Is $f\left( x \right)$ a function?
  Is ${f^{ - 1}}\left( x \right)$ a function?
Inverse of a Quadratic Function
Consider the quadratic function: $f(x) = (x+4)^2 + 2$ $f (x) = (x + 4)^{2} + 2$
1. Graph the function $f\left( x \right)$ and state the domain and range.
2. Graph the inverse ${f^{ - 1}}\left( x \right)$ and state the domain and range.
3. Is ${f^{ - 1}}\left( x \right)$ a function?
  If not, describe how to restrict the domain of $f\left( x \right)$ so that the inverse of $f\left( x \right)$ can be a function.
Determine the equation of the inverse.
Algebraically determine the equation of the inverse ${f^{ - 1}}\left( x \right)$ $f^{- 1} (x)$ , given:
1. $f\left( x \right) = - 5x + 4$
2. $f\left( x \right) = {\left( {7x - 8} \right)^3} - 1$
3. $f\left( x \right) = \frac{{3x}}{{2 + x}}$

No Javascript

It looks like you have javascript disabled.

You can still navigate around the site and check out our free content, but some functionality, such as sign up, will not work.

If you do have javascript enabled there may have been a loading error; try refreshing your browser.